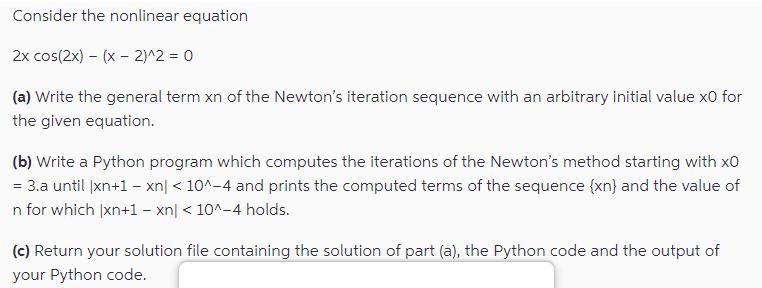
Consider the nonlinear equation 2x cos(2x) - (x-2)^2 = 0 (a) Write the general term xn of the Newton's iteration sequence with an arbitrary initial value x0 for the given equation. (b) Write a Python program which computes the iterations of the Newton's method starting with x0 = 3.a until |xn+1 - xn| < 10^-4 and prints the computed terms of the sequence (xn) and the value of n for which |xn+1 - xn| < 10^-4 holds. (c) Return your solution file containing the solution of part (a), the Python code and the output of your Python code.