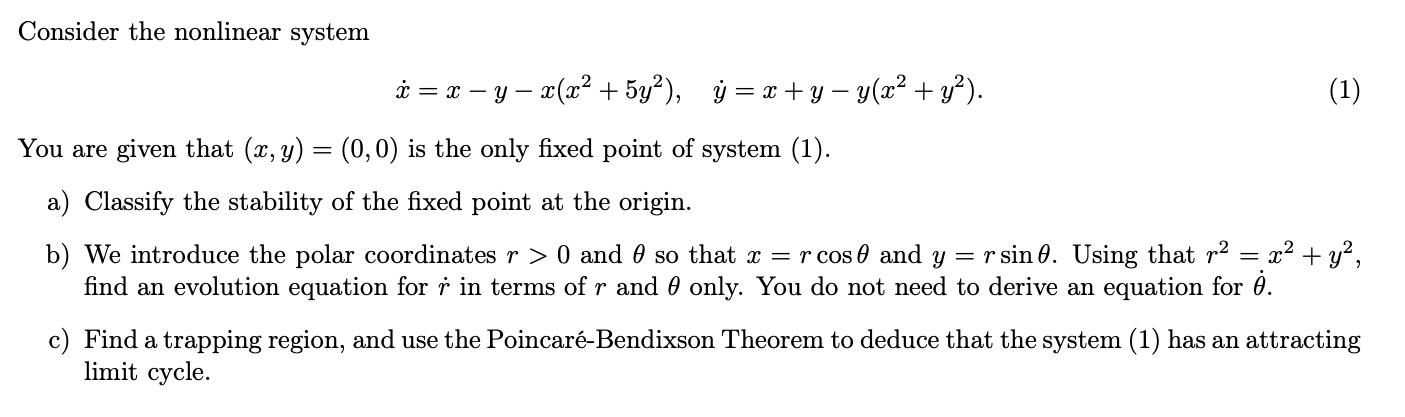
Consider the nonlinear system * = x y x(x +5), =x+yg ( +). (1) You are given that (x, y) = (0,0) is the only fixed point of system (1). - a) Classify the stability of the fixed point at the origin. = b) We introduce the polar coordinates r >0 and so that x = r cos 0 and y =rsin. Using that r x + y, find an evolution equation for r in terms of r and only. You do not need to derive an equation for 8. c) Find a trapping region, and use the Poincar-Bendixson Theorem to deduce that the system (1) has an attracting limit cycle.