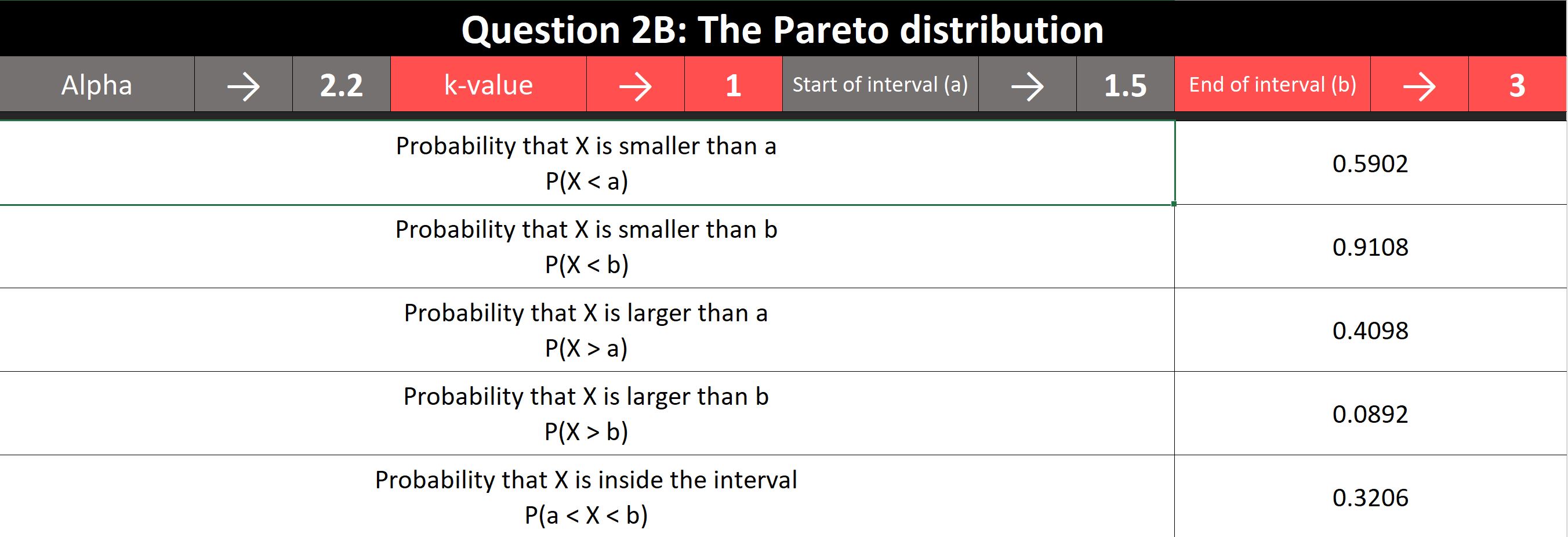
Consider the Pareto distribution and interval for insurance payouts, in thousands of dollars, shown in Figure 1. This distribution shows how the value of insurance claims fit a Pareto distribution. The indicated interval is an arbitrarily chosen interval, starting at 1.5 and ending at 3. In this distribution, most claims are for small values of X (where X denotes the value of the claim, in thousands of dollars), but a few larger claims will always have a probability of being made.

Start of interval = 1.5 (corresponding to a claim of $1,500)

End of interval = 3 (corresponding to a claim of $3,000)

k = 1 (corresponding to an excess, or deductible, of $1,000)

? (Alpha) = 2.2 (corresponding to a suitable parameter value)

What is the probability that a claim will be smaller than the interval? What does this probability tell us about insurance claims in a real-world context?

What is the probability that a claim will fall within the interval?

What is the probability that a claim will be larger than the interval? What does this probability tell us about insurance claims in a real-world context?

Question 23: The Pareto distribution 2,2 k-Value 9 1 Start of interval (a) 9 LS End ofinterval (b) 9 Probability that X is smaller than a 0.5902 P(X a) Probability that X is larger than b O 0892 P(X > b) ' Probability that X is inside the interval 0.3206 P(a