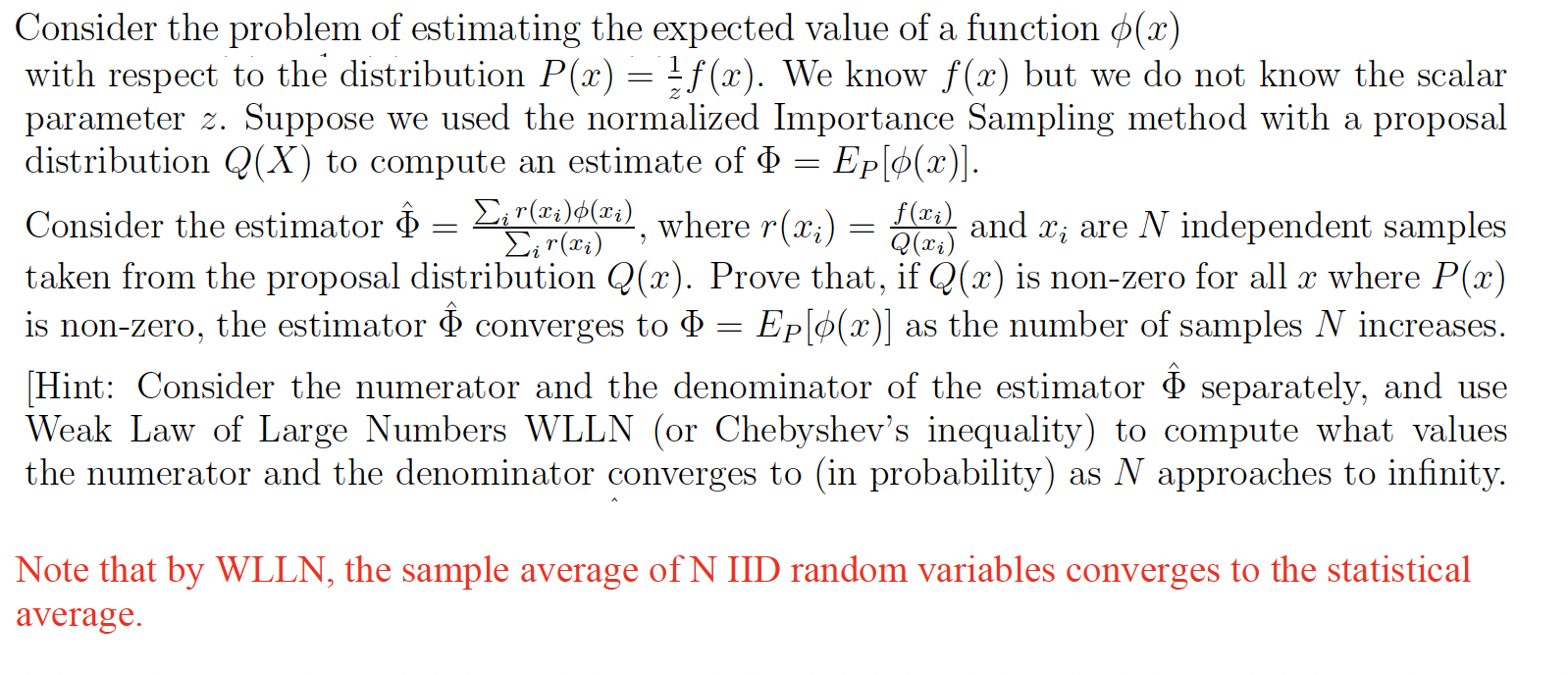
Consider the problem of estimating the expected value of a function (x) with respect to the distribution P(x) = f(x). We know f(x) but we do not know the scalar parameter z. Suppose we used the normalized Importance Sampling method with a proposal distribution Q(X) to compute an estimate of $ = Ep[0(x)]. Q(xi) Consider the estimator = r (10))(2), where r(x) = ((r) and x; are N independent samples ir(xi) taken from the proposal distribution Q(x). Prove that, if Q(x) is non-zero for all x where P(x) is non-zero, the estimator & converges to = Ep[0(x)] as the number of samples N increases. [Hint: Consider the numerator and the denominator of the estimator & separately, and use Weak Law of Large Numbers WLLN (or Chebyshev's inequality) to compute what values the numerator and the denominator converges to (in probability) as N approaches to infinity. Note that by WLLN, the sample average of N IID random variables converges to the statistical average.