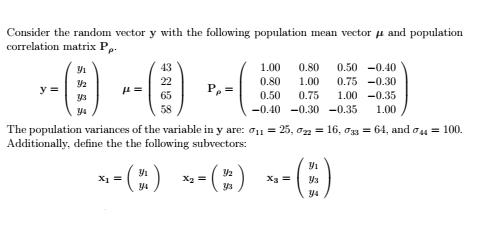
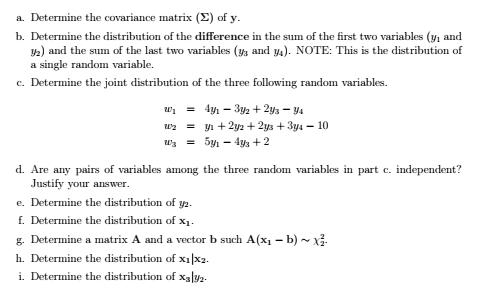
Consider the random vector y with the following population mean vector and population correlation matrix P y = Y/1 3/2 (0) H = X1 = 43 22 65 58 The population variances of the variable in y are: Additionally, define the the following subvectors: x = (1/h) y/ y4 P = 1.00 0.80 0.50 -0.40 0.80 1.00 0.75 -0.30 0.50 0.75 1.00 -0.35 -0.40 -0.30 -0.35 1.00 1 = 25, 022=16, 033 = 64, and 44 = 100. X3 = y/ Y3 y4 a. Determine the covariance matrix (E) of y. b. Determine the distribution of the difference in the sum of the first two variables (y and 32) and the sum of the last two variables (ya and y). NOTE: This is the distribution of a single random variable. c. Determine the joint distribution of the three following random variables. W = 4y-3y2 + 2y3 - Y4 202 = + 2y2+2y+3y4 - 10 wx = 5y -4ys +2 d. Are any pairs of variables among the three random variables in part c. independent? Justify your answer. e. Determine the distribution of y2. f. Determine the distribution of x. g. Determine a matrix A and a vector b such A(x - b) ~ x- h. Determine the distribution of x1|x2. i. Determine the distribution of x3y2.