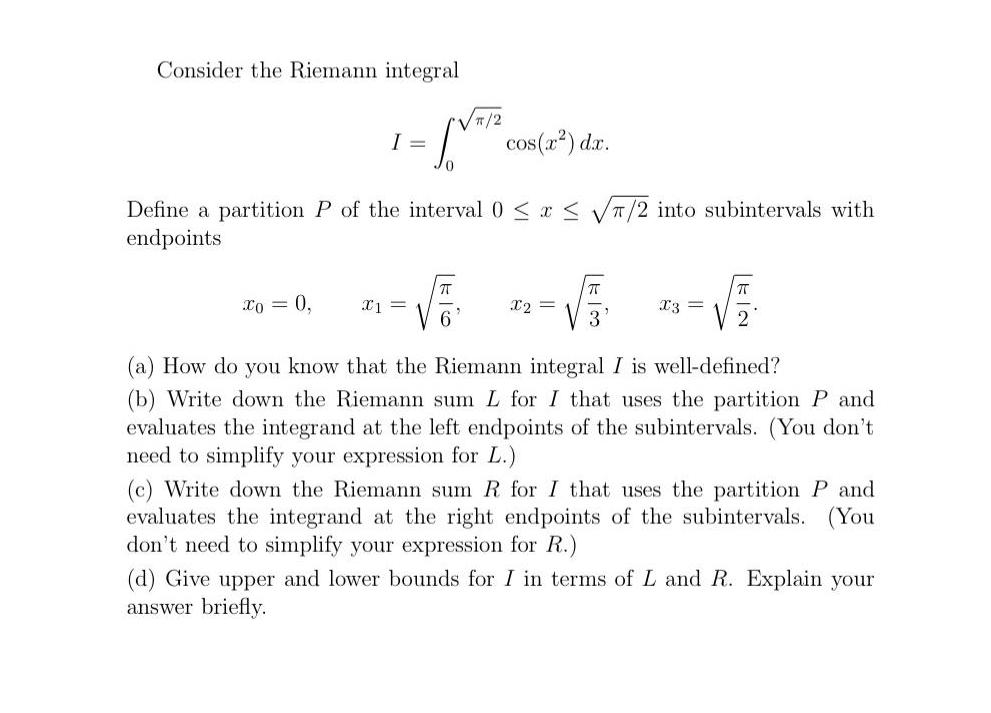
Consider the Riemann integral I = - [V* cos(x) dx. Define a partition P of the interval 0 x /2 into subintervals with endpoints Xo = 0, x1 = 6 x2 = 70 3 x3 = LEIN (a) How do you know that the Riemann integral I is well-defined? (b) Write down the Riemann sum L for I that uses the partition P and evaluates the integrand at the left endpoints of the subintervals. (You don't need to simplify your expression for L.) (c) Write down the Riemann sum R for I that uses the partition P and evaluates the integrand at the right endpoints of the subintervals. (You don't need to simplify your expression for R.) (d) Give upper and lower bounds for I in terms of L and R. Explain your answer briefly.