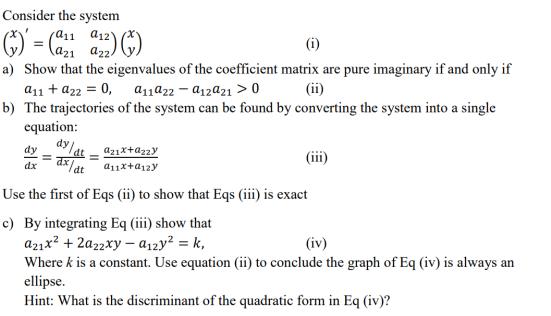
Consider the system (3) = (1 a21 azz a) Show that the eigenvalues of the coefficient matrix are pure imaginary if and only if a11 + a22 = 0, 911922-a12a21 > 0 (ii) b) The trajectories of the system can be found by converting the system into a single equation: dy dy/dt_ax+azzy dx dx/dax+any Use the first of Eqs (ii) to show that Eqs (iii) is exact c) By integrating Eq (iii) show that a21x + 2a22xy-a12y = k, (iv) Where k is a constant. Use equation (ii) to conclude the graph of Eq (iv) is always an ellipse. Hint: What is the discriminant of the quadratic form in Eq (iv)? (iii)