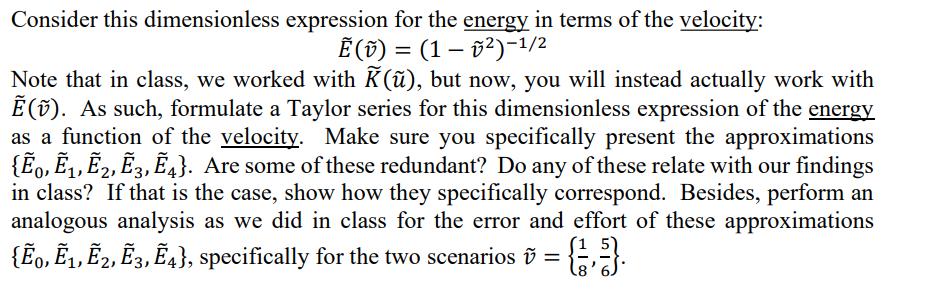
Consider this dimensionless expression for the energy in terms of the velocity: (v) = (1 )-1/2 Note that in class, we worked with K(), but now, you will instead actually work with (). As such, formulate a Taylor series for this dimensionless expression of the energy as a function of the velocity. Make sure you specifically present the approximations {0, E, E2, E3, 4}. Are some of these redundant? Do any of these relate with our findings in class? If that is the case, show how they specifically correspond. Besides, perform an analogous analysis as we did in class for the error and effort of these approximations {0, 2, E3, 4}, specifically for the two scenarios = {1}.