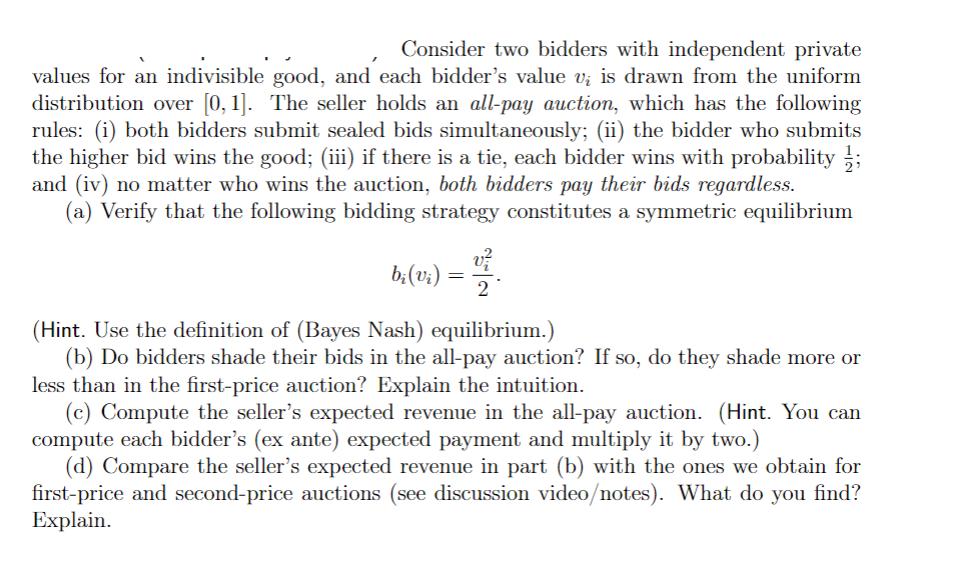
Consider two bidders with independent private values for an indivisible good, and each bidder's value vi is drawn from the uniform distribution over [0, 1]. The seller holds an all-pay auction, which has the following rules: (i) both bidders submit sealed bids simultaneously; (ii) the bidder who submits the higher bid wins the good; (iii) if there is a tie, each bidder wins with probability ; and (iv) no matter who wins the auction, both bidders pay their bids regardless. (a) Verify that the following bidding strategy constitutes a symmetric equilibrium bi (vi) = 2 (Hint. Use the definition of (Bayes Nash) equilibrium.) (b) Do bidders shade their bids in the all-pay auction? If so, do they shade more or less than in the first-price auction? Explain the intuition. (c) Compute the seller's expected revenue in the all-pay auction. (Hint. You can compute each bidder's (ex ante) expected payment and multiply it by two.) (d) Compare the seller's expected revenue in part (b) with the ones we obtain for first-price and second-price auctions (see discussion video/notes). What do you find? Explain.