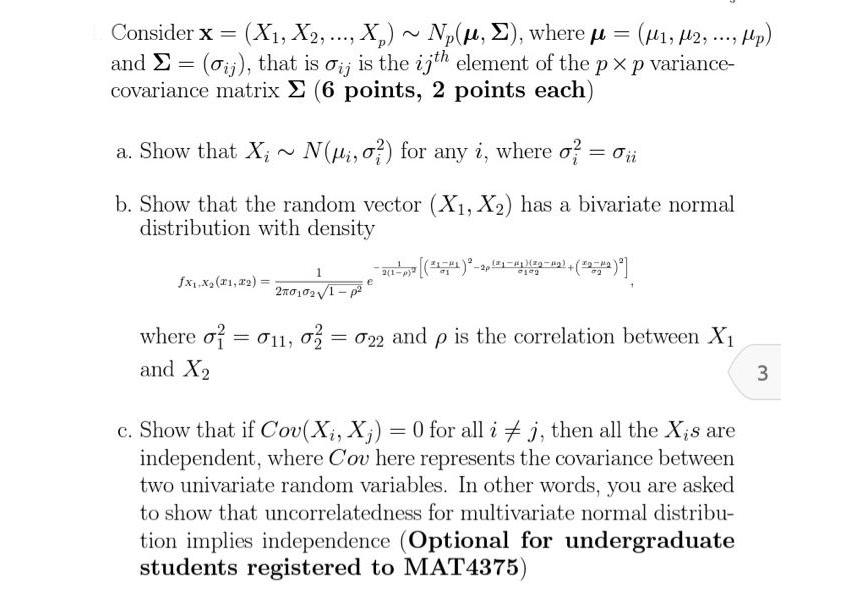
Consider x = (X1, X2,..., X)~N(, ), where = (1, 2, ..., Hp) and (ij), that is ij is the ijth element of the pp variance- covariance matrix (6 points, 2 points each) a. Show that X; ~N(, o) for any i, where = ii Xi b. Show that the random vector (X1, X2) has a bivariate normal distribution with density fx1.x, (1,2)= 1 2 1 - = 2(1 - p) (21-1)-2, (#1-11) (22-2)+(2-2)] where =11, 02022 and p is the correlation between X and X2 c. Show that if Cov(Xi, X;) = 0 for all i j, then all the Xis are independent, where Cou here represents the covariance between two univariate random variables. In other words, you are asked to show that uncorrelatedness for multivariate normal distribu- tion implies independence (Optional for undergraduate students registered to MAT4375) 3