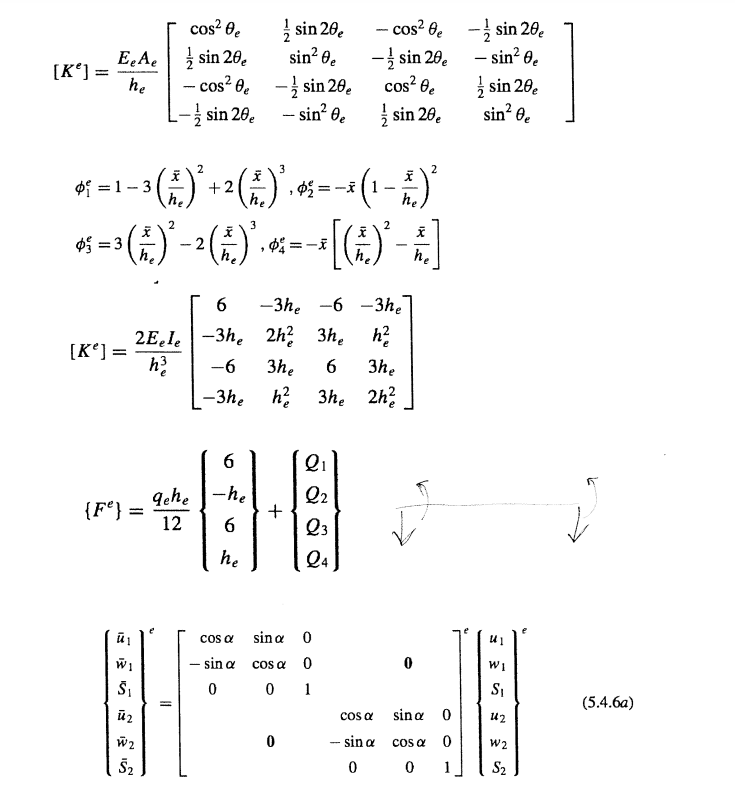
cos e sin 20 -cos e E.A sin 20 sin e -sin 20 - sin 20 sin 0. [K] = he - cos -sin 20 cos be sin 20 - - sin e sin 20 sin O sin 20 *-1-3 (A)+2(A) = 2 3 2 3 3 = (1-)* -x (1) = (#) - (#)' --[(#)**] =3 ' = -x [() 6 -3he -6 -3he ] 2Eele -3he 2h2 3he h [Ke] = h -6 3he 6 3he -3he h 3he 2h 6 Q1 gehe -he Q2 {Fe} = ==== + 12 6 Q3 he Q4 W1 S EES COS sina 0 - sin a cos 0 0 WI 001 S (5.4.6a) 2 cos sina 0 142 W2 0 - sin a cos a 0 W2 52 00 1 S 2. (40 points) 1. Using a neat figure of an Euler-Bernoulli beam element, state (no need to derive) the corresponding strong form and the weak form. 2. A four-node, three-element planar frame structure is shown below. Nodes 1,3 and 4 are built-in supports. 3 m 2 m The 2 m B ZA 4 m X Fc The The force Fc is horizontal, and is acting at the point of element 3 which is a third of the way along BD. The distributed force fb is acting vertically as shown, and is specified as vertical force per unit length (this length being along the element 3, i.e., this is force per unit length along BD). For Element 3, obtain the elemental force vector {f} in the global X - Z coordinate system.