could you please solve this question of heat transfer in details as soon as possible?

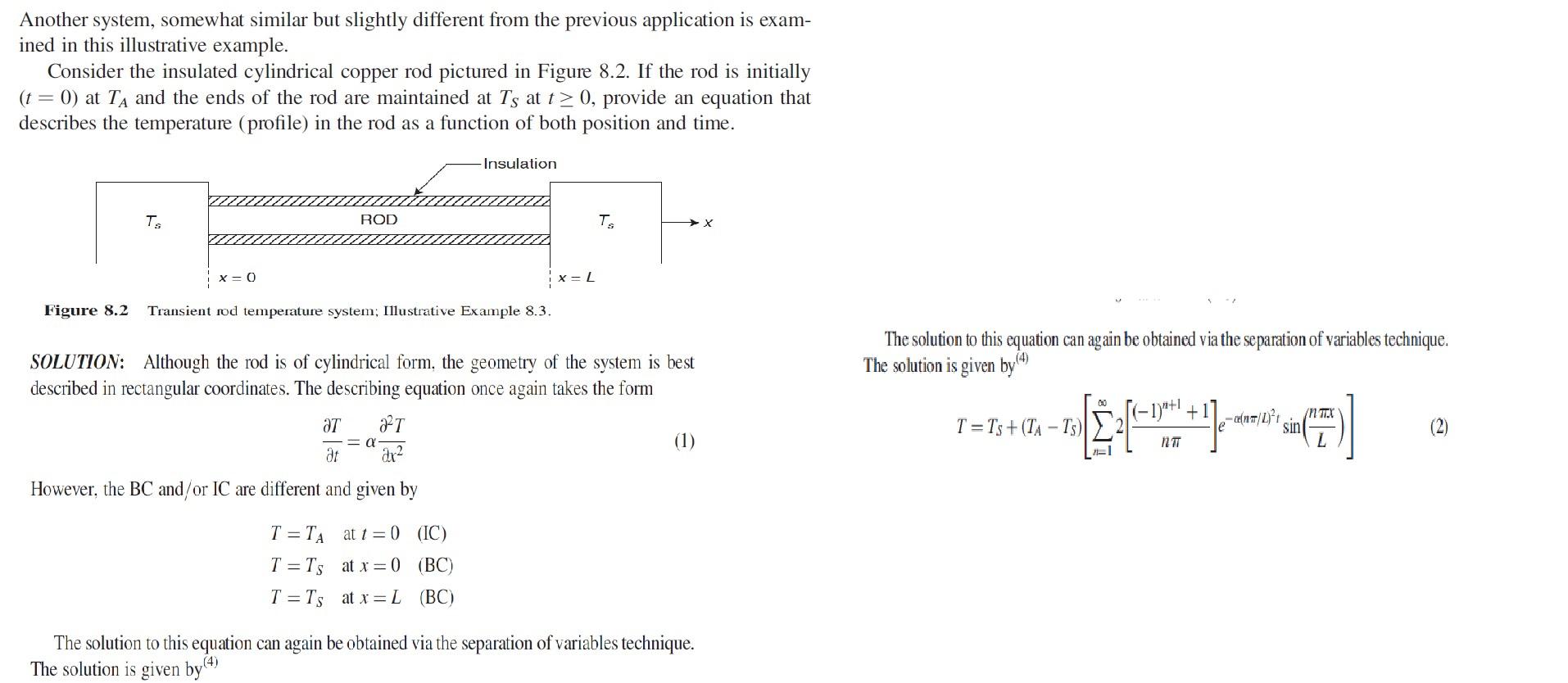
Another system, somewhat similar but slightly different from the previous application is exam- ined in this illustrative example. Consider the insulated cylindrical copper rod pictured in Figure 8.2. If the rod is initially (t = 0) at TA and the ends of the rod are maintained at Ts at t> 0, provide an equation that describes the temperature (profile) in the rod as a function of both position and time. -Insulation Ts ROD Ts x=0 x=L Figure 8.2 Transient rod temperature system; Illustrative Example 8.3. SOLUTION: Although the rod is of cylindrical form, the geometry of the system is best described in rectangular coordinates. The describing equation once again takes the form at # (1) at dx? However, the BC and/or IC are different and given by The solution to this equation can again be obtained via the separation of variables technique. The solution is given by (-1)*+1+ T = Ts+ (TA - Ts) 2 -(077Lt sm) in TX sin =a NTT II T = TA at 1= 0) (IC) T =Ts at x = 0) (BC) T=Ts at x=L(BC) The solution to this equation can again be obtained via the separation of variables technique. The solution is given by(4) ) Another system, somewhat similar but slightly different from the previous application is exam- ined in this illustrative example. Consider the insulated cylindrical copper rod pictured in Figure 8.2. If the rod is initially (t = 0) at TA and the ends of the rod are maintained at Ts at t> 0, provide an equation that describes the temperature (profile) in the rod as a function of both position and time. -Insulation Ts ROD Ts x=0 x=L Figure 8.2 Transient rod temperature system; Illustrative Example 8.3. SOLUTION: Although the rod is of cylindrical form, the geometry of the system is best described in rectangular coordinates. The describing equation once again takes the form at # (1) at dx? However, the BC and/or IC are different and given by The solution to this equation can again be obtained via the separation of variables technique. The solution is given by (-1)*+1+ T = Ts+ (TA - Ts) 2 -(077Lt sm) in TX sin =a NTT II T = TA at 1= 0) (IC) T =Ts at x = 0) (BC) T=Ts at x=L(BC) The solution to this equation can again be obtained via the separation of variables technique. The solution is given by(4) )