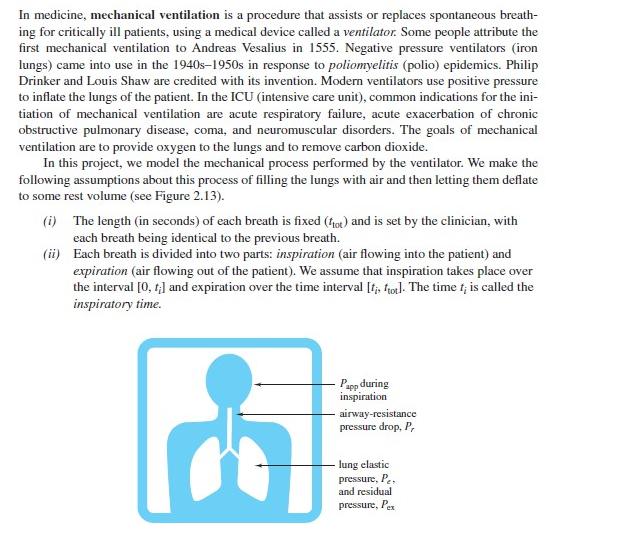???

In medicine, mechanical ventilation is a procedure that assists or replaces spontaneous breath- ing for critically ill patients, using a medical device called a ventilator. Some people attribute the first mechanical ventilation to Andreas Vesalius in 1555. Negative pressure ventilators (iron lungs) came into use in the 1940s-1950s in response to poliomyelitis (polio) epidemics. Philip Drinker and Louis Shaw are credited with its invention. Modern ventilators use positive pressure to inflate the lungs of the patient. In the ICU (intensive care unit), common indications for the ini- tiation of mechanical ventilation are acute respiratory failure, acute exacerbation of chronic obstructive pulmonary disease, coma, and neuromuscular disorders. The goals of mechanical ventilation are to provide oxygen to the lungs and to remove carbon dioxide. In this project, we model the mechanical process performed by the ventilator. We make the following assumptions about this process of filling the lungs with air and then letting them deflate to some rest volume (see Figure 2.13). (i) The length (in seconds) of each breath is fixed (fot) and is set by the clinician, with each breath being identical to the previous breath. (ii) Each breath is divided into two parts: inspiration (air flowing into the patient) and expiration (air flowing out of the patient). We assume that inspiration takes place over the interval [0, t;] and expiration over the time interval [ti, fot]. The time t; is called the inspiratory time. [1] Papp during inspiration airway-resistance pressure drop, P, lung elastic pressure, Pe and residual pressure, Pex (iii) During inspiration the ventilator applies a constant pressure Papp to the patient s air- way, and during expiration this pressure is zero, relative to atmospheric pressure. This is called pressure-controlled ventilation. (iv) We assume that the pulmonary system (lung) is modeled by a single compartment. Hence, the action of the ventilator is similar to inflating a balloon and then releasing the pressure. (v) At the airway there is a pressure balance: (1) P+ P + Pex = P + where P, denotes pressure losses due to resistance to flow into and out of the lung, P, is the elastic pressure due to changes in volume of the lung, Pex is a residual pressure that remains in the lung at the completion of a breath, and Paw denotes the pressure applied to the airway. (Paw = Papp during inspiration and Paw = 0 during expiration.) The residual pressure P is called the end-expiratory pressure. (vi) Let V(t) denote the volume of the lung at time t, with V.(t), 0 t t;, denoting its volume during inspiration and V.(t), t; sts for its volume during expiration. We assume that V;(0) = V. (f) = 0. The number V;(1) = Vr is called the tidal volume of the breath. (vii) We assume that the resistive pressure P, is proportional to the flows into and out of the lung such that P, = R(dv/dt), and we assume that the proportionality constant R is the same for inspiration and expiration. (viii) Furthermore, we assume that the elastic pressure is proportional to the instantaneous volume of the lung. That is, P = (1/C)V, where the constant C is called the compliance of the lung. (2) R Using the pressure equation in (1) together with the above assumptions, a mathematical model for the instantaneous volume in the single compartment lung is given by the following pair of first-order linear differential equations: (3) R Group Projects for Chapter 2 dt dv dt + 81 + V; + P = P = Papp 0tt;, Ve + Pex=0, 1; 1 hot. The initial conditions, as indicated in assumption (vi), are V;(0) = 0 and V(t) = V(t) = VT. The constant Per is not known a priori but is determined from the end condition on the expiratory volume: V(1) = 0. This will make each breath identical to the previous breath. To obtain a for- mula for Pex, complete the following steps. The initial conditions, as indicated in assumption (vi), are V;(0) = 0 and V(1) = V (1) = VT. The constant Per is not known a priori but is determined from the end condition on the expiratory volume: V(c) = 0. This will make each breath identical to the previous breath. To obtain a for- mula for Pex, complete the following steps. (a) Solve equation (2) for V;(1) with the initial condition V;(0) = 0. (b) Solve equation (3) for V (1) with the initial condition V (1;) = V. (c) Using the fact that V;(1) = V, show that Pes 1) Pupp Au/RC 1 (eV/RC ] (d) For R = 10 cm (HO)/L/sec, C = 0.02 L/cm (HO), Papp = 20 cm (HO), t; = 1 sec and tot = 3 sec, plot the graphs of V;(1) and V(1) over the interval [0, 1]. Compute Pex for