darrThis problem is meant to illustrate properties that can arise in non$-$random

walks. One formulation of the following mathematical problem is in terms of a

person visiting castles. Consider a network that is grid$-$like, such that nearest

neighbors are all connected and each node has three edges connected to it$.$

Starting from some central location, imagine flow of material can happen

either to the "right" or "left". When the flow reaches the next node, it cannot

travel back to the node from which it came, and it must go the opposite

direction $($right versus left or vice versa$)$ than it did at the previous node. As

the flow of material continues to traverse the network, it must alternate

directions through edges forever after that. Prove that if this pattern continues

that the flow of material must eventually return to the starting node. This is

equivalent to proving that the flow cannot get stuck traveling forever through

a loop of edges and nodes of which the starting node is not a part. $($Hint:

Consider the "dual" network constructed by replacing each vessel with a node

at its mid$-$point and connecting these new nodes only if the original edges were

connected at an original node. Map the flow through the original network onto

what flow means through the new "dual" network and consider what this

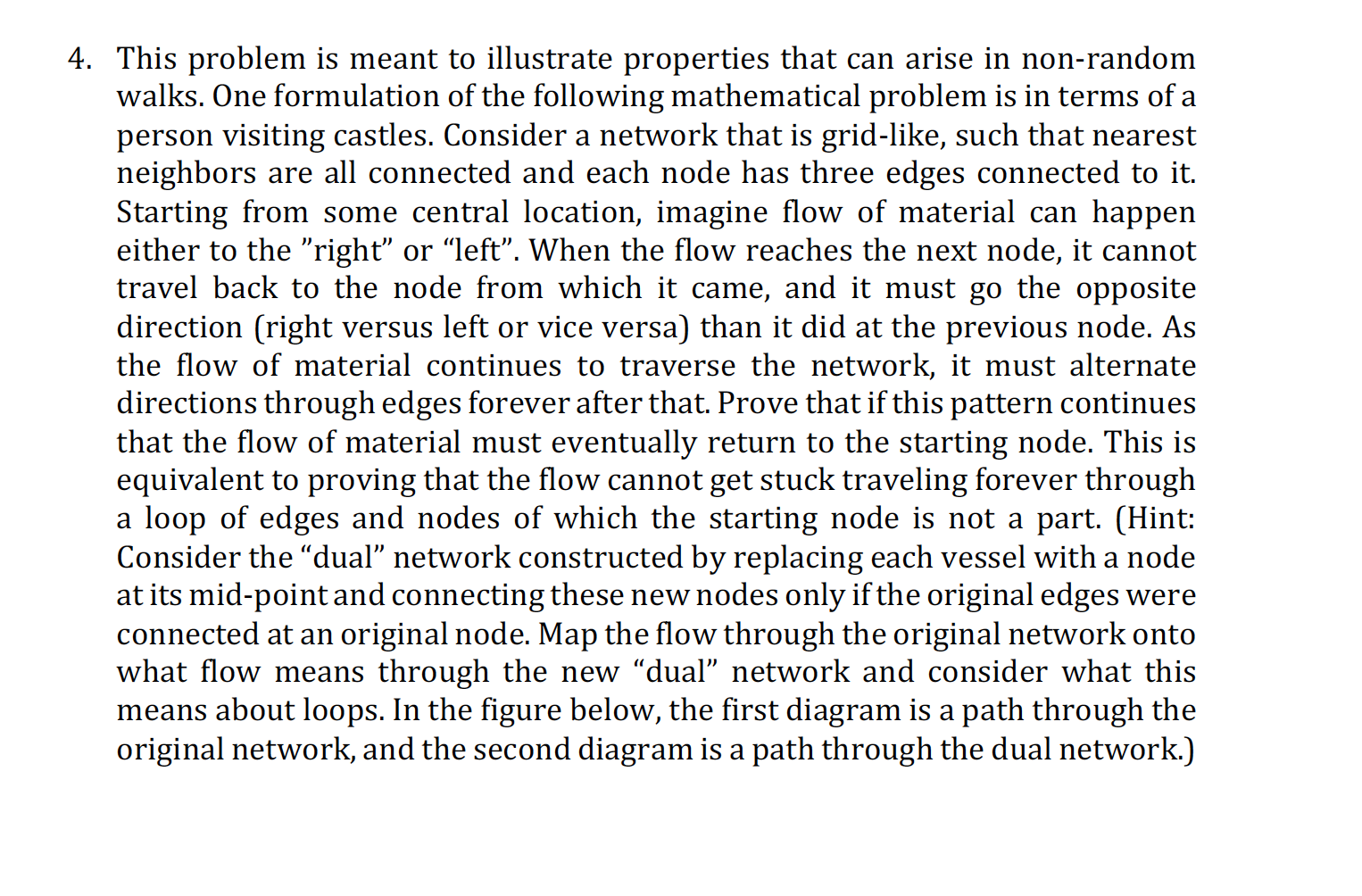
means about loops. In the figure below, the first diagram is a path through the

original network, and the second diagram is a path through the dual network.$)$