



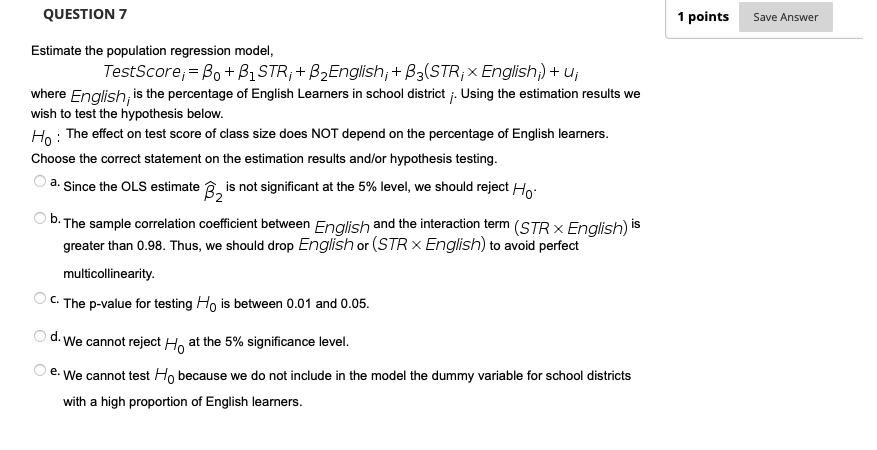
Data and Packages: We use the dataset "MASchools" in the package "AER" like we did in R-Exercise 2. The dataset contains information on test performance, school characteristics and student demographic backgrounds for school districts in Massachusetts (MA). In this exercise, we shall use R-functions in the packages "estimate" and "AER" (or "car"). We wish to learn the effect of student-teacher ratio (STR) on the 4th grade score (TestScore), which is defined as the sum of math, English, and science. In order to control for omitted variable bias, we shall include a few other additional variables and consider various functional forms. For all estimation exercises here the type of standard error should be "HC1" or equivalently "stata".QUESTION 1 1 points Save Answer Estimate the population regression model: TestScore; = Bo+ B1STR; + BzIncome; + B3Lunch; + u; where E[u; | STR,] = 0. Here, Income, and Lunch, are the per capita income and percentage of qualified students for reduced-price lunch in school district . What is the t value for the OLS estimate of the slope coefficient on STR, (two decimal places)? QUESTION 2 1 points Save Answer After estimating the regression model in Question 1, you want to test Ho : B2= B3=0 at the 5% significance level. Choose the correct statement. O a. We reject / because the standard error of ,, is approximately 0.128. We reject Ho because the maximum of the p-values associated with B2 and B, is larger than 0.05. C. We do not have sufficient evidence to reject Ho because p2 = 0.67. O d. We have to test two restrictions jointly and the critical value for this test is 3. O e. For this test, the F statistic is 154.9 and we use the F distribution with degrees of freedom 3 and 216.QUESTION 3 1 points Save Answer Using the estimated coefficients in Question 1 above, predict TestScore for "Dover", which is one of the school districts in the sample (two decimal places). QUESTION 4 1 points Save Answer Estimate the population regression model: TestScore; = Bo+ B1STR;+ B2Income; + ByLunch; + BAincome? + Bsincome} + u, where E[u; | STR;] = 0 and predict TestScore at the sample median of all the right-hand side variables. What is the predicted TestScore (two decimal places)? Note that in tutorial sessions we have done very similar exercises with the sample average of regressors instead of the sample median.QUESTION 5 1 points Save Answer For the population regression model in Question 4 above, we test Ho : The model is linear in Income- Compute the F-statistic for this test (two decimal places). QUESTION 6 1 points Save Answer Estimate the population regression model: In(TestScore;) = Bo+ BiIn(STR)) + BzIncome; + B3Lunch; + u; where In(x) denotes the natural log of x. Note that the R-function log() computes the natural log, i.e., log(x) computes the natural log of x. Choose the correct statement. a. We should not have included Income and Lunch because the hypothesis that B, = 0 and B3 = 0 and cannot be rejected at the 5% significance level. Ob. The model here is worse than the models in Question 1 and Question 4 because the model here has the smallest p2 among the three models. C. The estimated elasticity of TestScore to STR is approximately -0.0167 and it is significant at the 5% level. The estimation results suggest that a 1% increase in S/R would reduce TestScore by 1.67 points on the test. Je. The estimation results suggest that decreasing S / R by one student would induce a 1.67 percent increase in TestScore.QUESTION 7 1 points Save Answer Estimate the population regression model, TestScore; = Bo+ B1STR; + B2English; + B3(STR; x English;) + u; where English; is the percentage of English Learners in school district ;. Using the estimation results we wish to test the hypothesis below. Ho : The effect on test score of class size does NOT depend on the percentage of English learners. Choose the correct statement on the estimation results and/or hypothesis testing. a. Since the OLS estimate is not significant at the 5% level, we should reject H. b. The sample correlation coefficient between English and the interaction term (STR x English) is greater than 0.98. Thus, we should drop English or (STR x English) to avoid perfect multicollinearity. C. The p-value for testing Ho is between 0.01 and 0.05. O d. We cannot reject Ho at the 5% significance level. e. We cannot test Ho because we do not include in the model the dummy variable for school districts with a high proportion of English learners
















