Question
1. Prove each of the following for all n > 1 by the Principle of Mathematical Induction. a) 1 + 3 +5 +.... b)
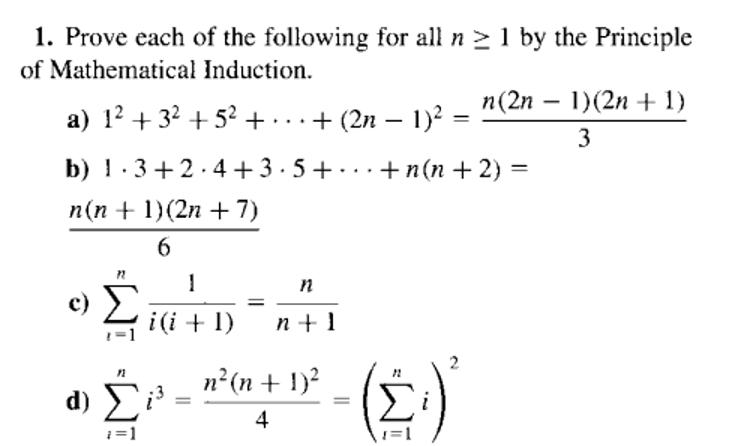
1. Prove each of the following for all n > 1 by the Principle of Mathematical Induction. a) 1 + 3 +5 +.... b) 1.3 +2.4 +3.5 +...+n(n + 2) = n(n + 1)(2n + 7) 6 + (2n 1)2 = n(2n 1)(2n + 1) 3 1 ii + 1) 4 n + 1 ni(n + 1)2 (2) = ;=1
Step by Step Solution
3.29 Rating (146 Votes )
There are 3 Steps involved in it
Step: 1
Solutions a 1 3 5 2n1 3n2n12n13 Stepbystep proof Base case n 1 1 3 1 2 1 1 2 1 1 3 1 1 True Inductive hypothesis Assume the statement is true for n ki...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get StartedRecommended Textbook for
Accounting Business Reporting For Decision Making
Authors: Jacqueline Birt, Keryn Chalmers, Albie Brooks, Suzanne Byrne, Judy Oliver
4th Edition
978-0730302414, 0730302415
Students also viewed these Mathematics questions
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
View Answer in SolutionInn App



