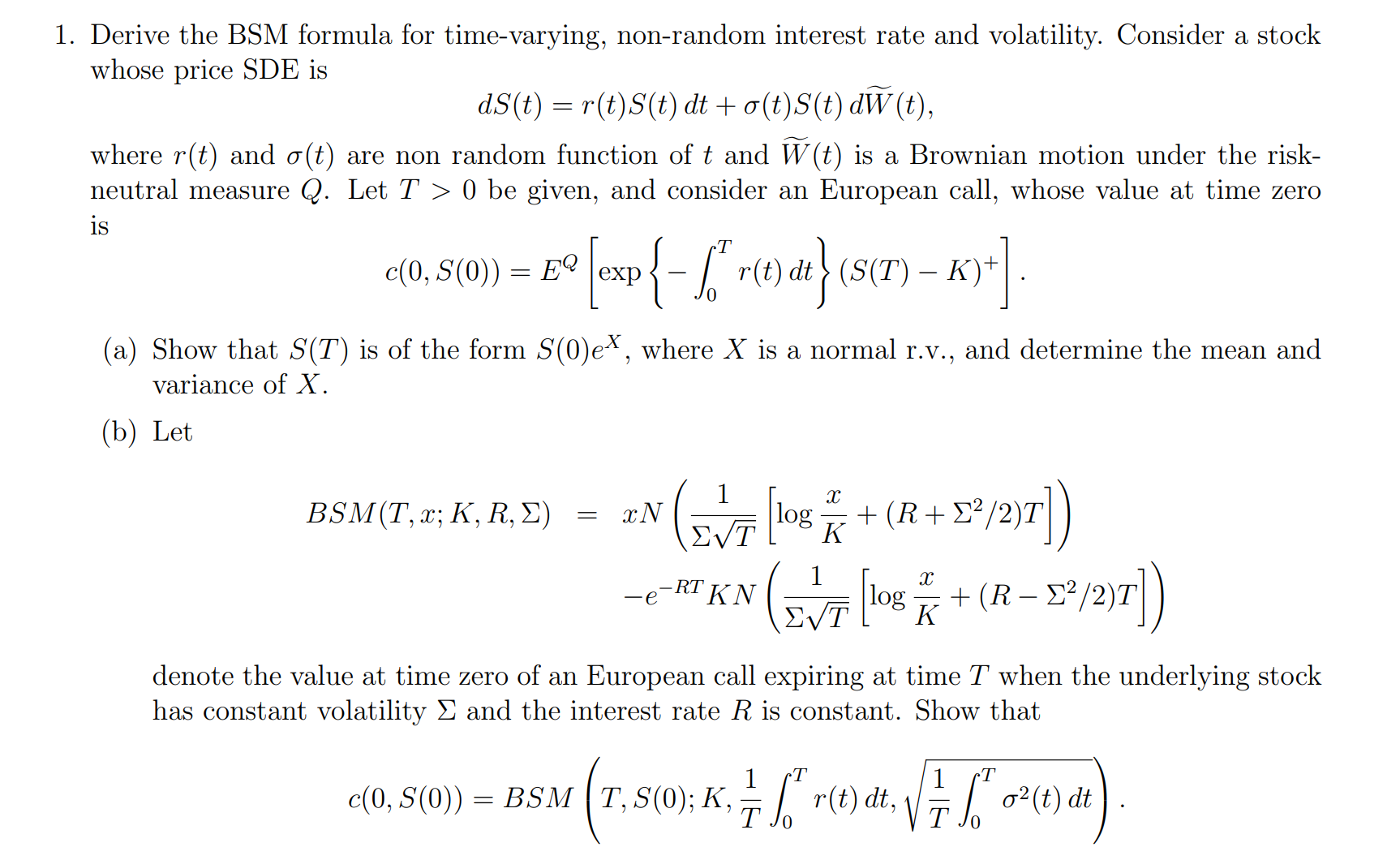
Derive the BSM formula for time$-$varying, non$-$random interest rate and volatility. Consider a stock

whose price SDE is

$dS(t)=r(t)S(t)dt+(t)S(t)dwidetilde(W)(t)$

where $r(t)$ and $(t)$ are non random function of $t$ and widetilde$(W)(t)$ is a Brownian motion under the risk$-$

neutral measure $Q.$ Let $T>0$ be given, and consider an European call, whose value at time zero

is

$c(0,S(0))=E^Q[exp\{-{\displaystyle \underset{0}{\overset{T}{}}}r(t)dt\}(S(T)-K{)}^{+}].$

$($a$)$ Show that $S(T)$ is of the form $S(0)e^x,$ where $x$ is a normal r$.$v$.,$ and determine the mean and

variance of $x.$

$($b$)$ Let

$BSM(T,x$;$K,R,)=xN(\frac{1}{\sqrt[\phantom{2}]{T}}[log(\frac{x}{K})+(R+\frac{{}^2}{2})T])$

$-e^{-RT}KN(\frac{1}{\sqrt[\phantom{2}]{T}}[log(\frac{x}{K})+(R-\frac{{}^2}{2})T])$

denote the value at time zero of an European call expiring at time $T$ when the underlying stock

has constant volatility $$ and the interest rate $R$ is constant. Show that

$c(0,S(0))=BSM(T,S(0)$;$K,\frac{1}{T}{\displaystyle \underset{0}{\overset{T}{}}}r(t)dt,\sqrt[\phantom{2}]{\frac{1}{T}{\displaystyle \underset{0}{\overset{T}{}}}{}^2(t)dt})$