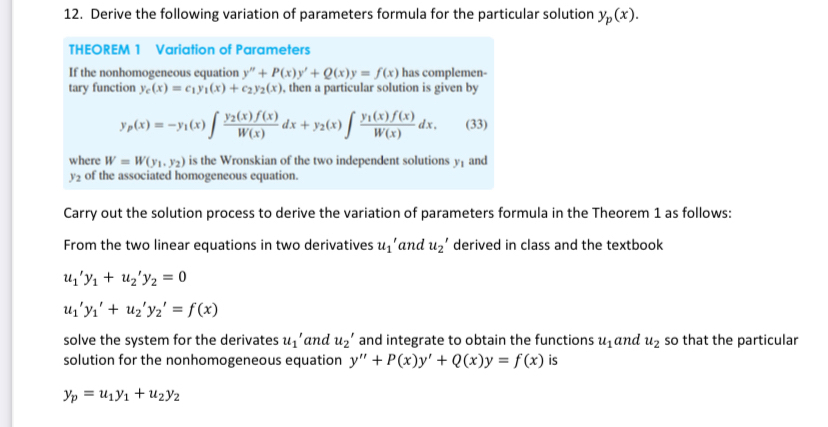
Derive the following variation of parameters formula for the particular solution $y_p(x).$

THEOREM $1$ Variation of Parameters

If the nonhomogeneous equation $y^{\text{'}\text{'}}+P(x)y^{\text{'}}+Q(x)y=f(x)$ has complementary function $y_c(x)=c_1{y}_1(x)+c_2{y}_2(x),$ then a particular solution is given by

$y_p(x)=-y_1(x){\displaystyle \underset{\phantom{}}{\overset{\phantom{}}{}}}\frac{y_2(x)f(x)}{W(x)}dx+y_2(x){\displaystyle \underset{\phantom{}}{\overset{\phantom{}}{}}}\frac{y_1(x)f(x)}{W(x)}dx,$

where $W=W(y_1,y_2)$ is the Wronskian of the two independent solutions $y_1$ and $y_2$ of the associated homogeneous equation.

Carry out the solution process to derive the variation of parameters formula in the Theorem $1$ as follows:

From the two linear equations in two derivatives $u_1^{\text{'}}$ and $u_2^{\text{'}}$ derived in class and the textbook

$u_1^{\text{'}}{y}_1+u_2^{\text{'}}{y}_2=0$

$u_1^{\text{'}}{y}_1^{\text{'}}+u_2^{\text{'}}{y}_2^{\text{'}}=f(x)$

solve the system for the derivates $u_1^{\text{'}}$ and $u_2^{\text{'}}$ and integrate to obtain the functions $u_1$ and $u_2$ so that the particular solution for the nonhomogeneous equation $y^{\text{'}\text{'}}+P(x)y^{\text{'}}+Q(x)y=f(x)$ is

$y_p=u_1{y}_1+u_2{y}_2$