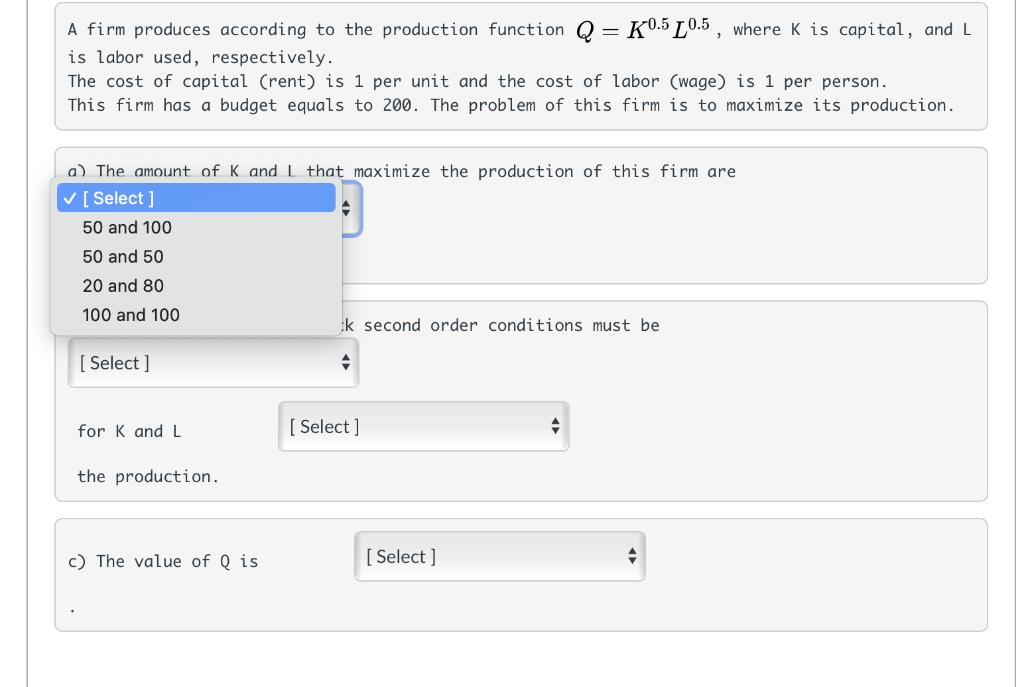
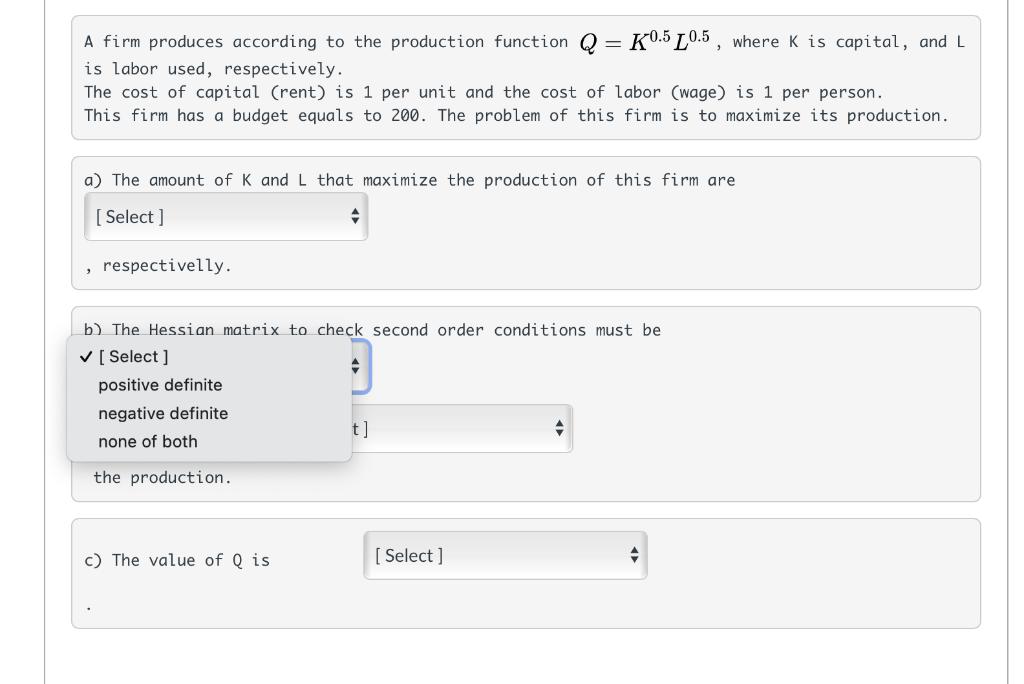
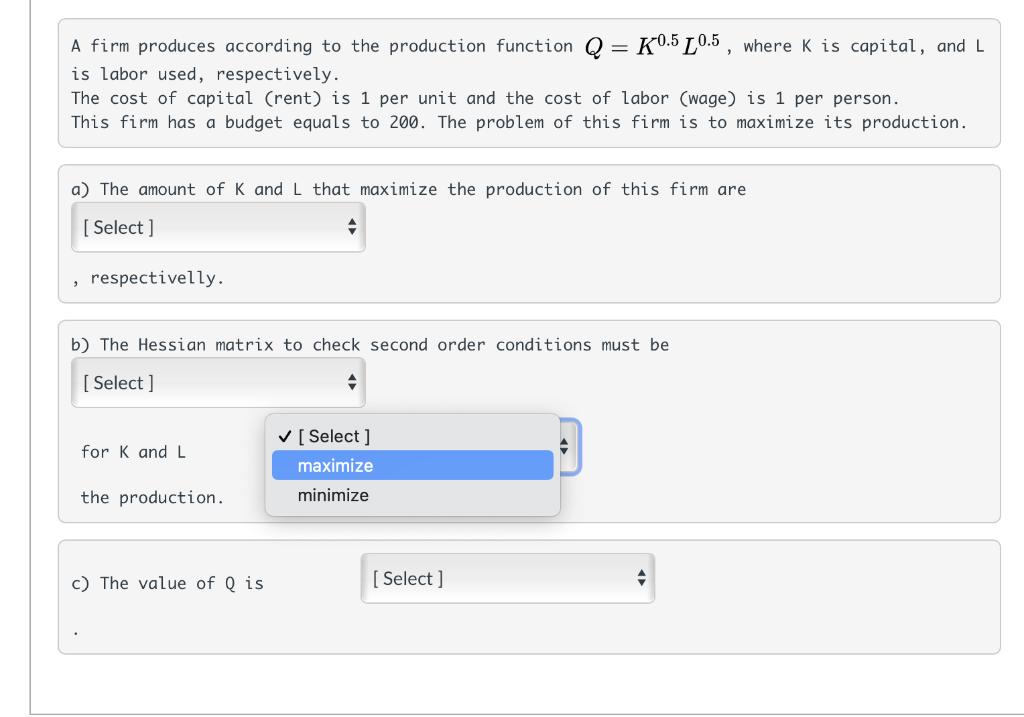
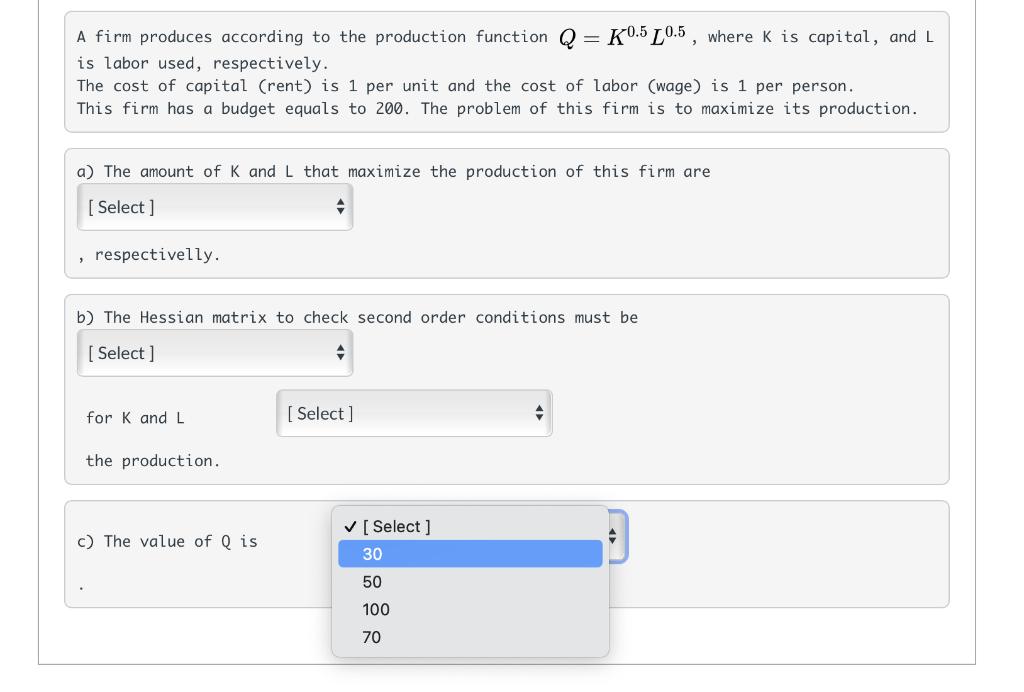
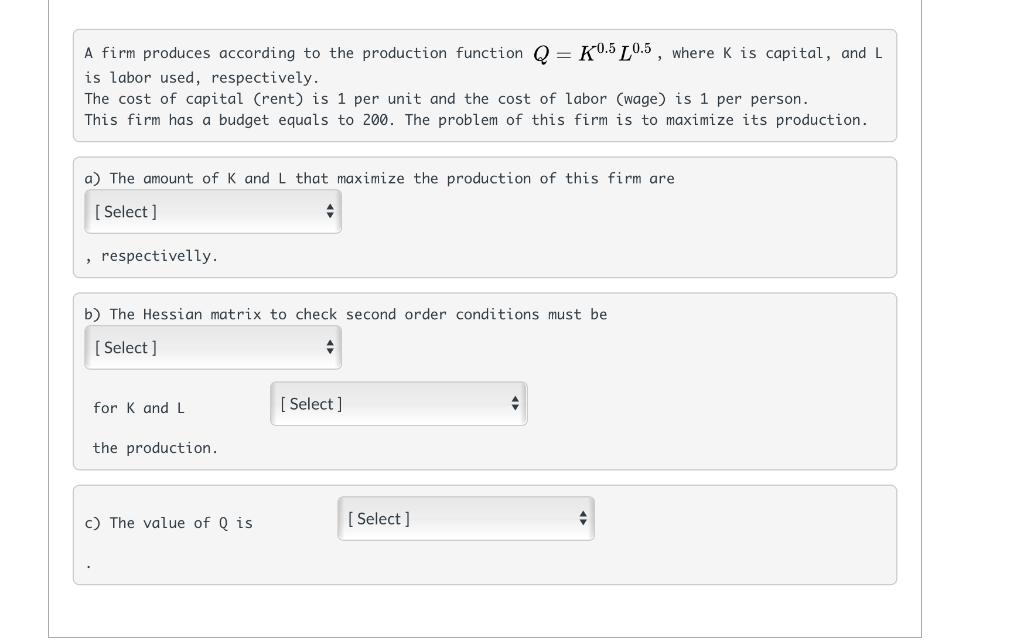
A firm produces according to the production function is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. 50 and 100 50 and 50 20 and 80 100 and 100 a) The amount of K and L that maximize the production of this firm are [Select] [Select] for K and L the production. c) The value of Q is [Select] K0.5 10.5, where K is capital, and L = k second order conditions must be + [Select] + A firm produces according to the production function is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. , respectivelly. a) The amount of K and L that maximize the production of this firm are [Select] + positive definite negative definite none of both the production. b) The Hessian matrix to check second order conditions must be [Select] + c) The value of Q is t] = K0.5 10.5, where K is capital, and L [Select] + A firm produces according to the production function Q=K0.5 10.5, where K is capital, and L is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. a) The amount of K and L that maximize the production of this firm are [Select] , respectivelly. b) The Hessian matrix to check second order conditions must be [Select] for K and L the production. c) The value of Qis [Select] maximize minimize [Select] A firm produces according to the production function is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. , respectivelly. a) The amount of K and L that maximize the production of this firm are [Select] for K and L b) The Hessian matrix to check second order conditions must be [Select] the production. c) The value of Qis [Select] [Select] = K0.5 10.5, where K is capital, and L 30 50 100 70 + A firm produces according to the production function Q = is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. a) The amount of K and L that maximize the production of this firm are [Select] respectivelly. b) The Hessian matrix to check second order conditions must be [Select] + for K and L the production. c) The value of Q is. K0.5 10.5, where K is capital, and L [Select] [Select] + A firm produces according to the production function is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. 50 and 100 50 and 50 20 and 80 100 and 100 a) The amount of K and L that maximize the production of this firm are [Select] [Select] for K and L the production. c) The value of Q is [Select] K0.5 10.5, where K is capital, and L = k second order conditions must be + [Select] + A firm produces according to the production function is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. , respectivelly. a) The amount of K and L that maximize the production of this firm are [Select] + positive definite negative definite none of both the production. b) The Hessian matrix to check second order conditions must be [Select] + c) The value of Q is t] = K0.5 10.5, where K is capital, and L [Select] + A firm produces according to the production function Q=K0.5 10.5, where K is capital, and L is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. a) The amount of K and L that maximize the production of this firm are [Select] , respectivelly. b) The Hessian matrix to check second order conditions must be [Select] for K and L the production. c) The value of Qis [Select] maximize minimize [Select] A firm produces according to the production function is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. , respectivelly. a) The amount of K and L that maximize the production of this firm are [Select] for K and L b) The Hessian matrix to check second order conditions must be [Select] the production. c) The value of Qis [Select] [Select] = K0.5 10.5, where K is capital, and L 30 50 100 70 + A firm produces according to the production function Q = is labor used, respectively. The cost of capital (rent) is 1 per unit and the cost of labor (wage) is 1 per person. This firm has a budget equals to 200. The problem of this firm is to maximize its production. a) The amount of K and L that maximize the production of this firm are [Select] respectivelly. b) The Hessian matrix to check second order conditions must be [Select] + for K and L the production. c) The value of Q is. K0.5 10.5, where K is capital, and L [Select] [Select] +