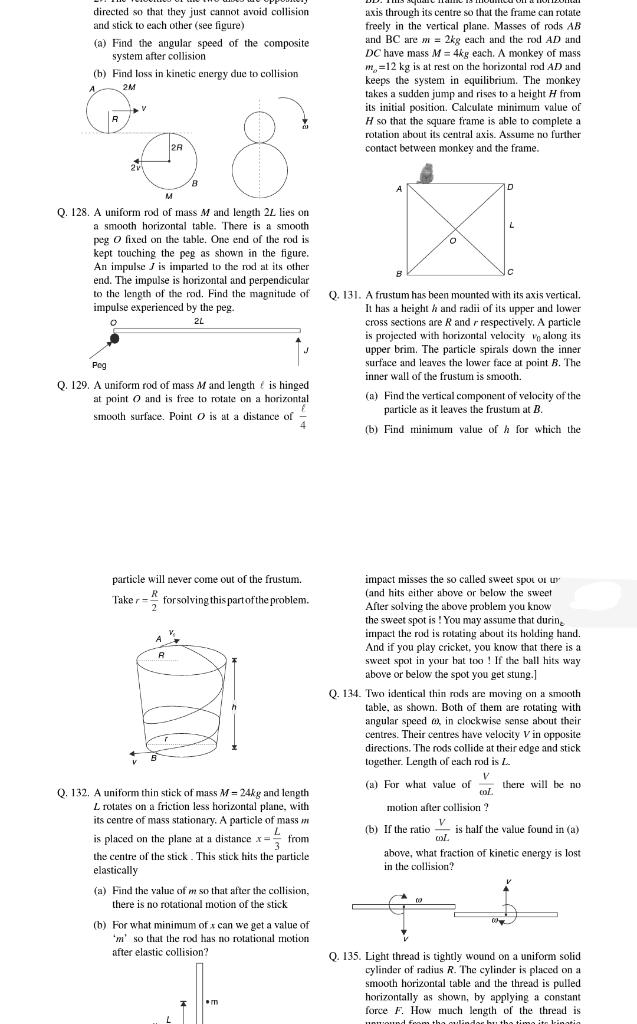
directed so that they just cannot avoid collision and stick to each other (see figure) (a) Find the angular speed of the composite system after collision (h) Find loss in kinetic energy due to collision 2M axis through its centre so that the frame can rotate freely in the vertical plane. Masses of rods AB and BC are m = 2kg each and the rod AD and DC have mass M = 4kg each. A monkey of mass m=12 kg is at rest on the horizontal rod AD and keeps the system in equilibrium. The monkey takes a sudden jump and rises to a height H from its initial position. Calculate minimum value of H so that the square frame is able to complete a rotation about its central axis. Assume no further contact between monkey and the frame. A R 2R 2v B D 2 0 M Q. 128. A uniform rod of mass M and length 22 lies on a smooth horizontal table. There is a smooth peg O fixed on the table. One end of the rod is kept touching the peg as shown in the figure. An impulse 1 is imparted to the rod at its other end. The impulse is horizontal and perpendicular to the length of the rod. Find the magnitude of Q. 131. A frustum has been mounted with its axis vertical. impulse experienced by the peg. It has a heighth and radii of its upper and lower 2L cross sections are R and r respectively. A particle is projected with horizontal velocity v along its upper brim. The particle spirals down the inner surface and leaves the lower face at point B. The inner wall of the frustum is smooth. Q. 129. A uniform rod of mass M and length is hinged at point and is free to rotate on a horizontal (a) Find the vertical component of velocity of the smooth surface. Point O is at a distance of particle as it leaves the frustum at B. 4 (b) Find minimum value of h for which the Peg particle will never come out of the frustum. Taker- forsolving this part of the problem. A R impact misses the so called sweet spot Ol Lr (and hits either above or below the sweet After solving the above problem you know the sweet spot is! You may assume that during impact the rod is rotating about its holding hand. And if you play cricket, you know that there is a sweet spot in your bat too! If the ball hits way above or below the spot you get stung.1 Q. 134. Two identical thin rods are moving on a smooth table, as shown. Both of them are rotating with angular speed as in clockwise sense about their centres. Their centres have velocity Vin opposite directions. The rods collide at their edge and stick together. Length of each rod is L. (a) For what value of there will be no col motion after collision ? V (b) If the ratio is half the value found in (a) ul above, what fraction of kinetic energy is lost in the collision? Q. 132. A uniform thin stick of mass M = 24kg and length L rotates on a friction less horizontal plane, with its centre of mass stationary. A particle of mass m L is placed on the plane at a distance x 3 from the centre of the stick. This stick hits the particle elastically (a) Find the value of m so that after the collision, a there is no rotational motion of the stick (b) For what minimum of can we get a value of 'm' so that the rod has no rotational motion after elastic collision? 0 Q. 135. Light thread is tightly wound on a uniform solid cylinder of radius R. The cylinder is placed on a smooth horizontal table and the thread is pulled horizontally as shown, by applying a constant force F. How much length of the thread is om the uliodor the time it list directed so that they just cannot avoid collision and stick to each other (see figure) (a) Find the angular speed of the composite system after collision (h) Find loss in kinetic energy due to collision 2M axis through its centre so that the frame can rotate freely in the vertical plane. Masses of rods AB and BC are m = 2kg each and the rod AD and DC have mass M = 4kg each. A monkey of mass m=12 kg is at rest on the horizontal rod AD and keeps the system in equilibrium. The monkey takes a sudden jump and rises to a height H from its initial position. Calculate minimum value of H so that the square frame is able to complete a rotation about its central axis. Assume no further contact between monkey and the frame. A R 2R 2v B D 2 0 M Q. 128. A uniform rod of mass M and length 22 lies on a smooth horizontal table. There is a smooth peg O fixed on the table. One end of the rod is kept touching the peg as shown in the figure. An impulse 1 is imparted to the rod at its other end. The impulse is horizontal and perpendicular to the length of the rod. Find the magnitude of Q. 131. A frustum has been mounted with its axis vertical. impulse experienced by the peg. It has a heighth and radii of its upper and lower 2L cross sections are R and r respectively. A particle is projected with horizontal velocity v along its upper brim. The particle spirals down the inner surface and leaves the lower face at point B. The inner wall of the frustum is smooth. Q. 129. A uniform rod of mass M and length is hinged at point and is free to rotate on a horizontal (a) Find the vertical component of velocity of the smooth surface. Point O is at a distance of particle as it leaves the frustum at B. 4 (b) Find minimum value of h for which the Peg particle will never come out of the frustum. Taker- forsolving this part of the problem. A R impact misses the so called sweet spot Ol Lr (and hits either above or below the sweet After solving the above problem you know the sweet spot is! You may assume that during impact the rod is rotating about its holding hand. And if you play cricket, you know that there is a sweet spot in your bat too! If the ball hits way above or below the spot you get stung.1 Q. 134. Two identical thin rods are moving on a smooth table, as shown. Both of them are rotating with angular speed as in clockwise sense about their centres. Their centres have velocity Vin opposite directions. The rods collide at their edge and stick together. Length of each rod is L. (a) For what value of there will be no col motion after collision ? V (b) If the ratio is half the value found in (a) ul above, what fraction of kinetic energy is lost in the collision? Q. 132. A uniform thin stick of mass M = 24kg and length L rotates on a friction less horizontal plane, with its centre of mass stationary. A particle of mass m L is placed on the plane at a distance x 3 from the centre of the stick. This stick hits the particle elastically (a) Find the value of m so that after the collision, a there is no rotational motion of the stick (b) For what minimum of can we get a value of 'm' so that the rod has no rotational motion after elastic collision? 0 Q. 135. Light thread is tightly wound on a uniform solid cylinder of radius R. The cylinder is placed on a smooth horizontal table and the thread is pulled horizontally as shown, by applying a constant force F. How much length of the thread is om the uliodor the time it list







