Direction: Answer the exercise and I provide some example or guide on how you do it.

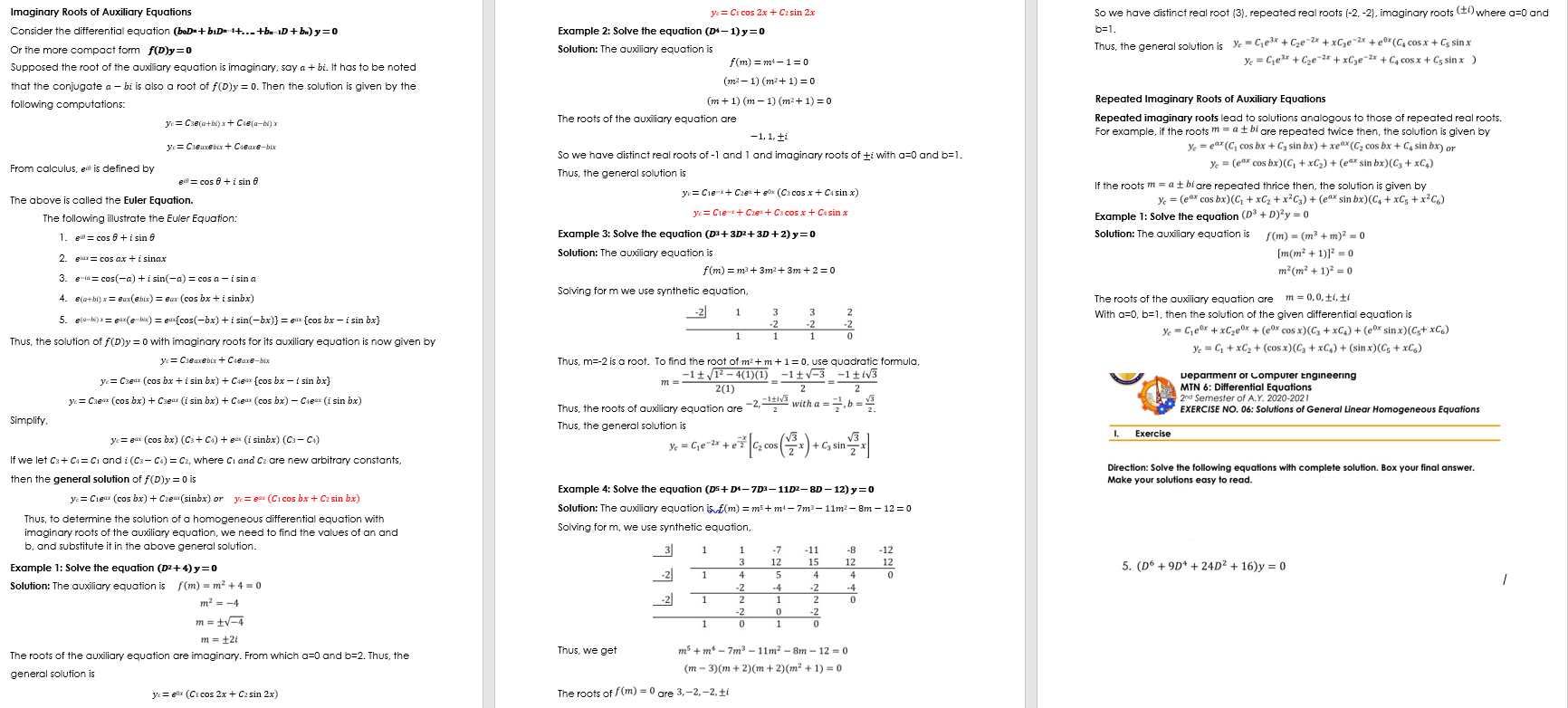
Imaginary Roots of Auxiliary Equations ye = Ci cos 2x + Czsin 2x So we have distinct real root (3), repeated real roots (-2, -2), imaginary roots () where a=0 and Consider the differential equation (boD= + biD" it..- +b. ID + b.) y=0 Example 2: Solve the equation (D'-1) y=0 b=1. Or the more compact form f(D)y=0 Solution: The auxiliary equation is Thus, the general solution is ye = Cielx + Cze-2x 4 xCze-2x + ex(C, cosx + Cs sinx Supposed the root of the auxiliary equation is imaginary, say a + bi. It has to be noted f(m) = m -1=0 ye = Cielx + Cze-2x + xCge"Zx + Cacosx + (s sinx ) that the conjugate a - bi is also a root of f(D)y = 0. Then the solution is given by the (m2 - 1) (m + 1) =0 following computations: (m + 1) (m - 1) (m + 1) =0 Repeated Imaginary Roots of Auxiliary Equations ye = Cze(atb() x + Cus(a-W)x The roots of the auxiliary equation are Repeated imaginary roots lead to solutions analogous to those of repeated real roots. -1, 1, ti For example, if the roots m = a 1 bi are repeated twice then, the solution is given by ye= Caeaxebix + Cieuxe-bix So we have distinct real roots of -1 and 1 and imaginary roots of ti with a=0 and b=1. ye = eax (C, cos bx + Cy sin bx) + xeax (Cz cos bx + Casinbx) or From calculus, " is defined by ye = (eax cos bx)(6, + xC2) + (ex sin bx)(C3 + xC4) el = cos 8 + i sin 8 Thus, the general solution is ye= Cie-s+ Crew + Box (Cacos x + CAsin x) If the roots m = a t bi are repeated thrice then, the solution is given by The above is called the Euler Equation. ye = (ex cos bx)(C1 + xCz + x2Ca) + (ex sin bx)(C4 + xCs + x2C6) The following illustrate the Euler Equation: ye = Cie-+ Cze* + Cocos x + Cosin x Example 1: Solve the equation (D3 + D) y = 0 1. el = cos 8 + i sin 6 Example 3: Solve the equation (D3+ 3D2+ 3D + 2) y=0 Solution: The auxiliary equation is f(m) = (m3 + m)? = 0 2. giux = cos ax + i sinax Solution: The auxiliary equation is [m(m3 + 1)]? = 0 3. @ in= cos(-a) + i sin(-a) = cosa - i sin a f(m) = m3 + 3m? + 3m + 2 =0 m (m' + 1)? = 0 . e(atbi) x = eux(ebix) = eax (cos bx + i sinbx) Solving for m we use synthetic equation, The roots of the auxiliary equation are m = 0,0, ti, ti 5. (e-W)x= ear(e wit) = eux(cos(-bx) + i sin(-bx)} = eux {cos bx - i sin bx} -2 1 3 -2 With a=0, b=1, then the solution of the given differential equation is ONN ye = Cielx + xCzeox + (ex cosx)(C3 + xC4) + (ex sinx)(Cs+ x(6) Thus, the solution of f (D)y = 0 with imaginary roots for its auxiliary equation is now given by Ye = C + x62 + (cosx)(C3 + xC4) + (sinx)(Cs + xC6) ye = CaeaxSbix + Cieuxe-bix Thus, m=-2 is a root. To find the root of m? + m + 1=0, use quadratic formula, ye = Caeux (cos bx + i sin bx) + Cieux {cos bx - i sin bx) Deparment or Computer Engineering 2(1) 2 MTN 6: Differential Equations ye = Caex (cos bx) + Caeux (i sin bx) + Cieux (cos bx) - Cieux (i sin bx) Thus, the roots of auxiliary equation are -2.-2" with a , b = ya 2nd Semester of A. Y. 2020-2021 EXERCISE NO. 06: Solutions of General Linear Homogeneous Equations Simplify, Thus, the general solution is ye = eax (cos bx) (C3+ C4) + sex (i sinbx) (Ca -CA) ye = Ce-2x + ez Ca cos (x) + Casin 2x Exercise If we let C3 + C.= Ci and i (Cs- C.) = Cz, where Ci and Cz are new arbitrary constants. Direction: Solve the following equations with complete solution. Box your final answer. then the general solution of f (D)y = 0 is Make your solutions easy to read. Example 4: Solve the equation (DS + D'-7D3-1102-8D -12) y= 0 ye= Cieux (cos bx) + Crew(sinbx) or ye= ex (Cicos bx + Czsin bx) Solution: The auxiliary equation is f(m) =m5+ m' - 7m3- 11m - 8m - 12 = 0 Thus, to determine the solution of a homogeneous differential equation with maginary roots of the auxiliary equation, we need to find the values of an and Solving for m, we use synthetic equation, b, and substitute it in the above general solution. -12 Example 1: Solve the equation (Dz+ 4) y= 0 5. (D6 + 9D* + 24D2 + 16)y = 0 Solution: The auxiliary equation is f (m) = m' +4 = 0 ONNNAGE m? = -4 ONNNA m = tv-4 m = 121 The roots of the auxiliary equation are imaginary. From which a=0 and b=2. Thus, the Thus, we get m' + m' - 7m3 - 11m? - 8m - 12 = 0 general solution is (m - 3)(m + 2)(m + 2)(m3 + 1) = 0 ye= elx (Ci cos 2x + Czsin 2x) The roots of f (m) = 0 are 3,-2,-2, tiSolutions of General Linear Homogeneous Equations Solution: The differential equation is translated in terms of the operation D as ye= Cle-2x + C262x + xC362x or yc=Cie-2x + 62x (C2+ xC3) Day - Dry - 2Dy = 0 or (D - D2- 2D) y = 0 Example 3: Solve the equation (4D1-403-23D2+ 12D + 36) y=0 Solutions of General Linear Homogeneous Equations The auxiliary equation is f(m) = ml - m - 2m = m (m? -m - 2) =m (m -2) (m + 1) =0 Solution: The auxiliary equation is The form of a general linear homogeneous differential equation with constant whose roots are 0, 2, -1. Then the general solution is f(m) =4m' -4m] -23m2+ 12m + 36 = 0 coefficients is given by yr= Cleor + Cze2x + Cae-1x To find the roots, we use synthetic division, d" y no dyn + a dxa-it...+an-idx+ ay+ any = 0 ye = Ci + Cze2x + Cae-x -23 12 36 This form may also be written in a compact form as f(D)y = 0 where f(D) is a linear 492 - 13+ 6y = 0 8 -30 -36 Example 4: Solve the equation * ax -15 -18 differential operation. Solution: The differential equation is translated in terms of the operation D as Theorem 1. If m is a root of the equation f(m)=0, then 4Day - 13Dy + 6y = 0 or (403 -13D + 6) y= 0 Thus, m=2 is a root. Again by synthetic division; f (D)y = f(D)emx = 0. The auxiliary equation is f(m) = 4m3 - 13m + 6 =0. 2 -15 -18 The above theorem asserts that y = aux is a solution of f(D)y = 0. In general, we 24 18 By synthetic division, we have: 12 9 0 have the solution as y = Cows, C is constant. We define f(m) = 0 as the auxiliary Thus, m=2 is another root. equation. -8 16 Thus . Distinct Real Roots of Auxiliary Equation f(m) =4m' - 4m] - 23m + 12m + 36 = 0 Let the roots of the auxiliary equation by mi, my .. . m. Then these will produce k Thus, 4m3 - 13m + 6 =(m + 2) (4m? - 8m + 3) = (m + 2) (2m -3) (2m - 1) =0. solutions as follows: The roots of the auxiliary equation are 27'2. The general solution is (m - 2) (m - 2) (4m2+ 12m + 9) =0 yl = 6mix, V2 = 6mix, . . .. yk = 6mix Ye = Cie-2x + CzeT + Cze# (m - 2) (m - 2) (2m + 3) (2m + 3) = 0 The general solution, denoted by y of the general linear homogeneous differential Thus, the roots of the auxiliary equation are 2 .2. The solution is equation with mi, ma,... me roots of the auxiliary equation is Repeated Real Roots of Auxiliary Equation ye = ezx (C + xCz) +e " (C3 + xC.) ye = Clemix + Czemart . ... +Ckemix if the auxiliary equation has a repeated root mi, then the general solution is Example 4: Solve the equation (D' + 303-602-280 -24) y=0 Example 1: Solve the differential equation y ty-2y = 0. yc = Clemx + xC26mix or ye= emix (Ci + xC2) Solution: The auxiliary equation is f(m) = m' + 3m] - 6m? -28m - 24 = 0 Solution: The differential equation is translated in terms of the operation D as If the auxiliary equation has roots mi that are repeated 3 times, then the general solution is To find the roots, we use synthetic division, Day + Dy - 2y =0 or (D2+ D - 2) y=0 ye = Clemix + xCzemix + x2Caemix or ye = emix (C1 + xC2 + x2Ca) 3 The auxiliary equation is f(m) = m>+m - 2 = (m +2) (m -1) =0. If the auxiliary equation has roots mi that are repeated n times, then the general solution is -6 -28 -24 16 24 ye= Clemix + xC26mix+ x2C38mix+.... +xx-1Chemix or ye=wix (Ci+ xCa+ x Cs+.... +x-1Cn) - 8 -12 0 The roots of f (m) = 0 are mi= -2, mz= 1. Thus, the general solution is Example 1: Solve the differential equation y"- 2y ty= 0. Thus, m=-2 is a root. Again by synthetic division; ye = C18-2x + C26x Solution: The differential equation is translated in terms of the operation D as 3 -8 -12 Example 2: Solve the equation (D2+ 20 -1) y =0. Day - 2Dy + y= 0 or (D2- 2D+ 1) y=0 4 0 The auxiliary equation is f(m) = m? - 2m + 1= (m -1) (m -1) =0. Thus, m=3 is another root. Solution: The auxiliary equation is f(m) = m?+ 2m -1= 0. The roots of the auxiliary The roots of f (m) = 0 ore mi= 1, ma= 1. The roots are repeated. Thus, the general solution is Thus, equation are obtained by quadratic formula, ye = Cier+ xCzer f(m) = m'+ 3m3- 6m2- 28m - 24 = 0 m = - -2+2" -4(1)(-1) -21 V8 -2+2v2 2(1) = -1+ V2 Example 2: Solve (DZ-4) (D -2) y=0. (m + 2) (m - 3) (m2 + 4m + 4) =0 Thus, the solution is given by Solution: The auxiliary equation is (m + 2) (m -3) (m + 2) (m + 2) =0 f(m) = (mz -4) (m - 2) =0 Thus, the roots of the auxiliary equation are -2, 3, -2, -2. The solution is ye = C18 (-1+V2) x + C28 (-1-V2) x (m + 2) (m - 2) (m - 2) =0 ye = e-2x (C1 + xC2 + x2C3) + C403x Example 3: Solve the equation y"-y"-2y'=0. The roots are -2, 2, 2. Thus, the general solution is