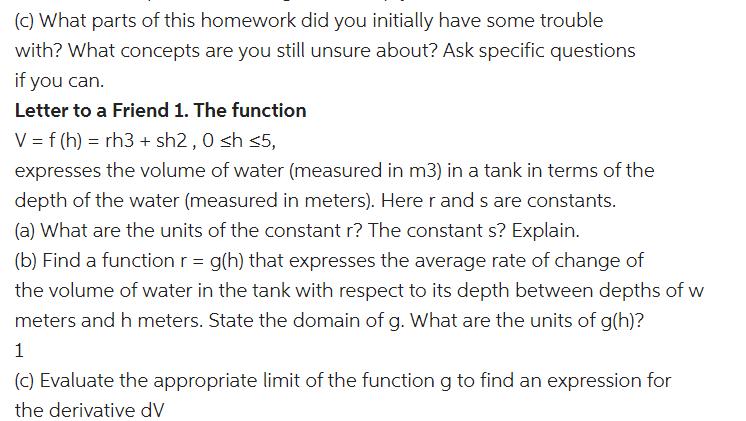
Directions: Use only the material in Sections 2.1-2.3, 2.7 and the class videos for this homework. No credit awarded for solutions that use later material or ideas beyond the scope of this class. Be sure to type your solution, using standard font and double-spacing. As in all assignments, pay particular attention to units. See the document Homework Checklist for the proper way to define a function. In particular, use meaningful variables (ie. not x and y) for the input and output. After completing the final version of your letter, answer the following questions. Put your answers to these questions before your letter. (a) List all the sources you used to do this homework. This includes, but is not limited to websites, textbooks, and people. (b) What concepts did this assignment help you to better understand? (c) What parts of this homework did you initially have some trouble with? What concepts are you still unsure about? Ask specific questions if you can. Letter to a Friend 1. The function V = f (h) = rh3 + sh2, 0 dh IIIh=w Check that your expression is dimensionally correct. (d) Suppose the tank holds 4 cubic meters of water when the depth is 2 meters, and 24 cubic meters when the depth is 4 meters. (i) Algebraically determine the values of the constants r and s. (ii) Evaluate the derivative dv dh IIIh=2 (iii) Explain the meaning of the derivative in part (ii) as it applies in this particular scenario. Include units in your explanation. (iv) Use part (ii) to approximate the change AV = f(h) -f (2) in volume in terms of a small change Ah-h-2~0 in depth. Then use this to approximate the volume of water in the thank when the depth is 1.9 meters. Explain your thinking. (v) Interpret the meaning of the derivative dv dh Illh=2 as it relates to the geometry of the tank. Letter to a Friend 2: (5 points). The equation b2(h -200)2 + (v -60)2 = c2 (1) relates the height h of an eagle (measured in feet) and it speed v (measured in ft/sec). Here b, and c are constants. (a) Determine the units of each of the constants b, and c. Explain. Let b = 1/2 and c = 50 for the remainder of the problem. 2 (b) Graph the relation (1) in desmos. Include a screenshot. (c) At 12:30pm the eagle is at a height of 140 feet. At the same time it is both gaining altitude and slowing down. Use this information to find a function v = f (h) that expresses the speed of the eagle in terms of its height for clock times near 12:30pm. Show all the algebra and explain your reasoning in making your final choice of the function. (d) Use the function f and Definition 1 of the derivative on page 141 of our text to evaluate the derivative dv dh |||h=140 at 12:30pm. (e) Explain the meaning of the derivative in part (d) as it applies in this particular scenario. Include units in your explanation. (f) Use your explanation from part (e) to approximate the change Av = f (h) -f (140) in speed in terms of a small change Ah = h -140~0 in altitude. Then use this to approximate the ealge's speed at a height of 142 feet and a time near 12:30pm. Explain. (g) Find an equation of the tangent line (using the given variables) to the curve (1) at the point corresponding to the derivative of part (d). Then graph the curve and the tangent line in desmos to check your computation of the derivative.