



Disclaimer: Must have an understanding of set theory to solve. Please solve by proving either side is a subset of the other.
I attached resources that may be useful in solving.
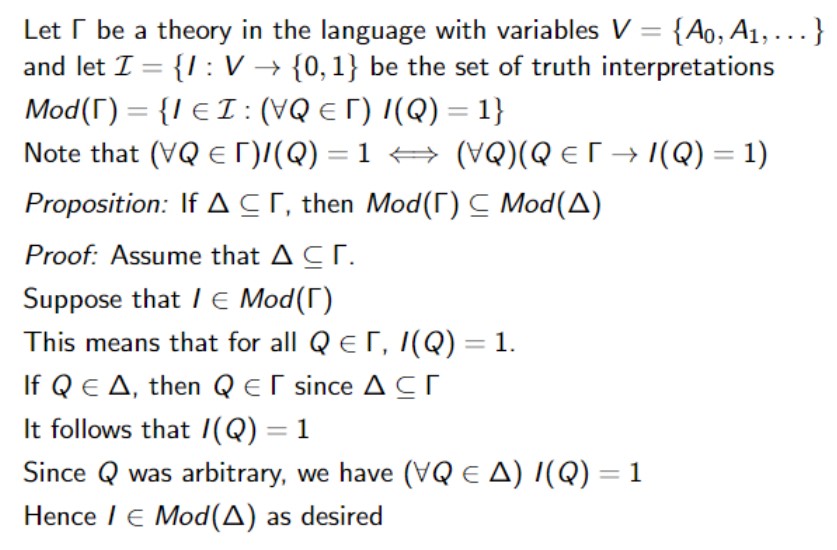
4. Show that for any sets F and A of sentences, Mod(F) n Mod(A) = Mod(I' u A). 1.8 Logical versus Topological Compactness In this section, we show how the set of truth interpretations may viewed as a topological space, in fact the classical Cantor space {0, 1}N.. We see that the Compactness Theorem for propositional logic may be seen as showing the compactness of this space. Fix the set of propositional variables PVcr : {A0, A1, . . .} and let I = {I : P Var )- {0, 1}} be the set of interpretations of the variables. Denition 1.8.1. 1. For any theory 1", let Mod(F) E I be the set of interpretations (or models) I : PVer > {E}, 1} such that I satises 1", that is, I (P) = 1 for all P E F. 2. The topology on I has for its basic open sets {Mod(I') : l" is nite}. This means that a set U Q I is open if it is the union of basic open sets, that is, for any I E U, there is some nite set 1" of sentences such that I E Mada") and Mcd(F) Q U. The notation Mod(F) is shorthand for the set of models of I'; the term 'model' is used here as synonymous with 'interpretation'. Note here that the sets T may contain arbitrary sentences, whereas we are restricting the interpretations I to the propositional variables. If 1" = {P} for some sentence P, then we just write Mod (P) for Mod ({P}) It is also important here that the family of basic open sets is countable, since there are only countably many sentences and thus only countably many nite sets of sentences. It follows that every open set is a countable union of basic open sets. Then, as usual, every union of Open sets is Open. Lemma 1.8.2. 1. For any nite theory T, there is a single sentence P each that Mod(F) = Mod(P). 2. For any theory P, I\\ Mod') is also a basic open set. 3. For any theories I' and A, Mod(F) Mod(A) = Moda' U A). The second item implies that every basic open set is also a closed set; such sets are said to be ctopen. The third item is needed to ensure that the intersection of two open sets is open. The proofs are left as exercises. Now a closed set in a topological space is simply the complement of an open set. Thus the union of two closed sets is closed and the intersection of any family of closed sets is closed. Let I be a theory in the language with variables V = { Ao, Al, ...} and let I = {/ : V -> {0, 1} be the set of truth interpretations {I = (0)/ (JP DA) : IP /} = (J)POW Note that (VQ E T)/(Q) = 1 (VQ)(QET -> 1(Q) = 1) Proposition: If A C T, then Mod(r) C Mod(A) Proof: Assume that A C T. Suppose that / E Mod(T) This means that for all Q ET, I(Q) = 1. If Q E A, then Q E I since A C T It follows that / (Q) = 1 Since Q was arbitrary, we have (VQ E A) I(Q) = 1 Hence / E Mod(A) as desiredCorollary Given two theories A and [ InACT and InACA It follows that Mod(r) C Mod(In A) and Mod(A) C Mod(r n A) Thus Mod(r) U Mod(A) C Mod(In A) The other inclusion is not true in general Example: Let [ = {A1 } and let A = {--A1} Then In A = 0, so Mod(In A) = I However, if / (A1) = 0, then I & Mod(r) U Mod(A)Given twotheories A and F Fgl'uand 4;qu It follows that Mod(F U A) Q 5400'\") and Mod(F U A) g Mod(A) Thus Mod\") U A) 9 Mod\") r1 Mod(A) 50 only the other direction needs to be proved now

















