Question: Discrete random variable X is described by the PMF {K Px (x) - -2, if x = 0, 1, 2 for all other values

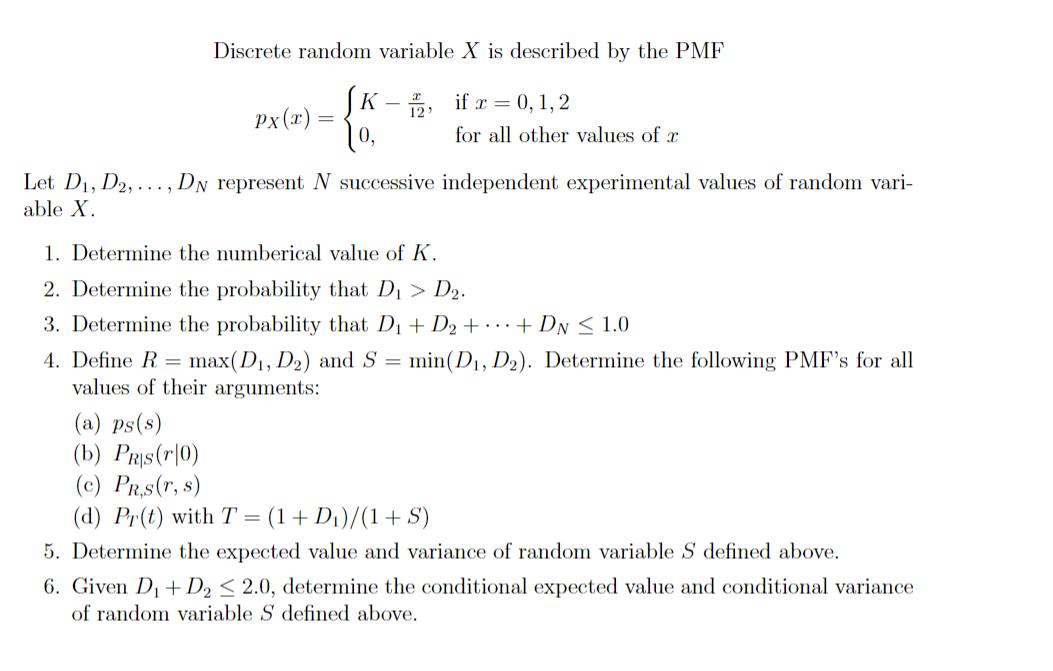
Discrete random variable X is described by the PMF {K Px (x) - -2, if x = 0, 1, 2 for all other values of r Let D, D2,.. , DN represent N successive independent experimental values of random vari- able X. 1. Determine the numberical value of K. 2. Determine the probability that D > D. 3. Determine the probability that D + D + + DN 1.0 4. Define R= max(D, D) and S = min(D, D). Determine the following PMF's for all values of their arguments: (a) ps(s) (b) PRIS (0) (c) PR,S(r, s) (d) Pr(t) with T = (1 + D)/(1+S) 5. Determine the expected value and variance of random variable S defined above. 6. Given D + D 2.0, determine the conditional expected value and conditional variance of random variable S defined above.

Step by Step Solution

★★★★★

3.31 Rating (151 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related General Management Questions!

The factorial moment-generating function of a discrete random variable X is given by Show that the rth derivative of FX(t) with respect to t at t = 1 is µ'(r), the rth factorial moment defined...

The probability distribution of the discrete random variable x is p(x) = krx, 0

1. Three times we randomly draw a number from the following numbers: 1 2 3. If Xi represents the ith draw, i = 1, 2, 3 then the probability mass function of Xi is given by And P(Xi = a) = 0 for all...

In the study described in the paper Exposure to Diesel Exhaust Induces Changes in EEG in Human Volunteers (Particle and Fibre Toxicology [2007]), 10 healthy men were exposed to diesel exhaust for 1...

Determine whether the statement is true or false. Justify your answer. 1. The area of the figure described by the system is 99 square units. 2. The graph shows the solution of the system 3. After...

Identification of pole positions in a system consider the system described by the difference equation y(n) = -r2y(n 2) + x(n) (a) Let r = 0.9 and x(n) = (n). Generate the output sequence y(n) for 0...

Suppose that independent trials are performed, with trial being a success with probability 1/(2i + 1). Let P n denote the probability that the total number of successes that result is an odd number....

The Fischer model with unbalanced price-setting. Suppose the economy is described by the model of Section 7.2, except that instead of half of firms setting their prices each period, fraction f set...

Determine the value c so that each of the following functions can serve as a probability distribution of the discrete random variable X: (a) f(x) = c (x2 + 4), for a: = 0, 1, 2, 3; (b) f(x) =...

Determine the values of c so that the following functions represent joint probability distributions of the random variables A" and Y: (a) f(x, y) cxy, for x = 1, 2, 3; y = 1, 2, 3; (b) f(x, y) = c\x...

1) Which of the following characteristics is not indicative of an enterprise quah ty? which significantly impact beneficiary with a controlling financial interest in a variable interest enti A) The...

Chikako is applying for a full-time, salaried position as an administrative assistant with Hitachi Systems, LLC. During the interview process, Chikako explains that she has low vision and will need...

Mike, Miko, Michael and Mikhael formed a partnership several years ago. They have decided to terminate operations and liquidate the business. The following balance sheet is drawn up as a guideline...

Millikan Oil Drop Lab Purpose . to explore the Millikan Oil Drop experiment . determine the value of the elementary charge Apparatus...

What is commonly used by a SOC to engage the IR team as soon as possible

CNBE, a prominent news channel, is gearing up to provide insightful coverage of recent elections, recognizing the importance of data-driven analysis. A comprehensive survey has been conducted,...

47 Portfolio Y is made up of RM9,000 in Security A with the beta of 0.7, and RM5,000 in Security B with the beta of 1.4. What is Portfolio Y's beta? Select one: A, 5.45 B. 1.15 C 0.55 D. 0.95

Juanita owns a home in Richardson, TX. She purchases a Homeowners Policy (HO-3) from Farm State Ins. Co. The policy provides $100,000 in liability coverage (coverage E) and $5,000 in Med Pay coverage...

Consider the loan processing cycle time data in Exercise 8.15. Set up a CUSUM chart for monitoring this process. Does the process seem to be in statistical control? x ï»p50...

An inspector for a military agency desires a variables sampling plan for use with an AQL of 1.5%, assuming that lots are of size 7,000. If the standard deviation of the lot or process is unknown,...

Discuss why it is important to establish control on the R chart first when using x and R control charts to bring a process into statistical control

Gnap (1995) conducted a survey to estimate the teacher workload in Maricopa County, Arizona, public school districts. Her target population was all first through sixth grade full-time public school...

An accounting firm is interested in estimating the error rate in a compliance audit it is conducting. The population contains 828 claims, and the firm audits an SRS of 85 of those claims. In each of...

Construct a sample ANOVA table for the replacement cost data in Exercise 8. What is your estimate for R2a? Do books on the same shelf tend to have more similar replacement costs? Suppose that c1 = 10...