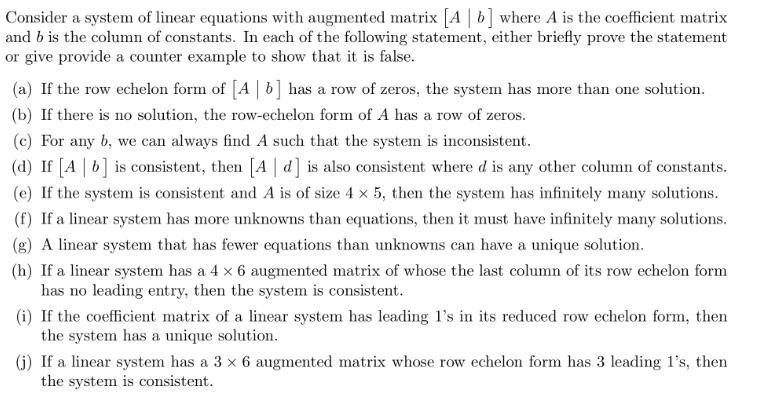
Consider a system of linear equations with augmented matrix [Ab] where A is the coefficient matrix and b is the column of constants. In each of the following statement, either briefly prove the statement or give provide a counter example to show that it is false. (a) If the row echelon form of [A | b] has a row of zeros, the system has more than one solution. (b) If there is no solution, the row-echelon form of A has a row of zeros. (c) For any b, we can always find A such that the system is inconsistent. (d) If [Ab] is consistent, then [A d] is also consistent where d is any other column of constants. (e) If the system is consistent and A is of size 4 x 5, then the system has infinitely many solutions. (f) If a linear system has more unknowns than equations, then it must have infinitely many solutions. (g) A linear system that has fewer equations than unknowns can have a unique solution. (h) If a linear system has a 4 x 6 augmented matrix of whose the last column of its row echelon form has no leading entry, then the system is consistent. (i) If the coefficient matrix of a linear system has leading I's in its reduced row echelon form, then the system has a unique solution. (j) If a linear system has a 3 x 6 augmented matrix whose row echelon form has 3 leading 1's, then the system is consistent.