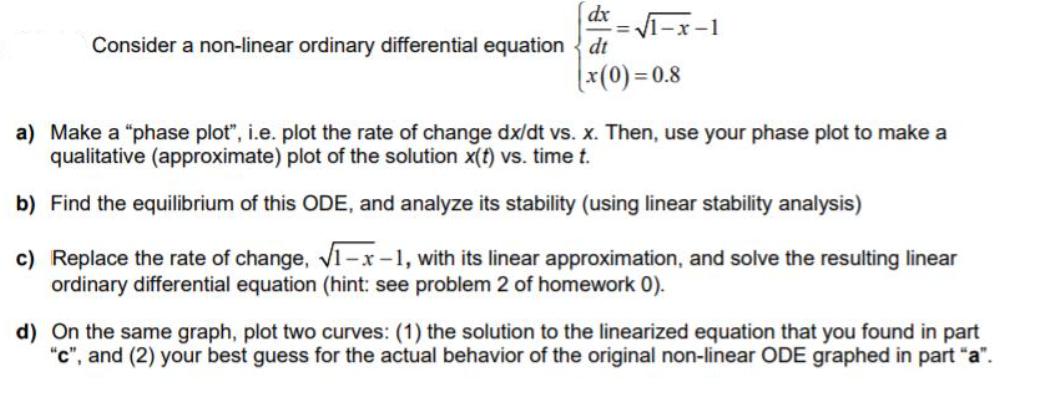
dx =1-x-1 Consider a non-linear ordinary differential equation dt (x(0) = 0.8 a) Make a "phase plot", i.e. plot the rate of change dx/dt vs. x. Then, use your phase plot to make a qualitative (approximate) plot of the solution x(t) vs. time t. b) Find the equilibrium of this ODE, and analyze its stability (using linear stability analysis) c) Replace the rate of change, 1-x-1, with its linear approximation, and solve the resulting linear ordinary differential equation (hint: see problem 2 of homework 0). d) On the same graph, plot two curves: (1) the solution to the linearized equation that you found in part "c", and (2) your best guess for the actual behavior of the original non-linear ODE graphed in part "a".