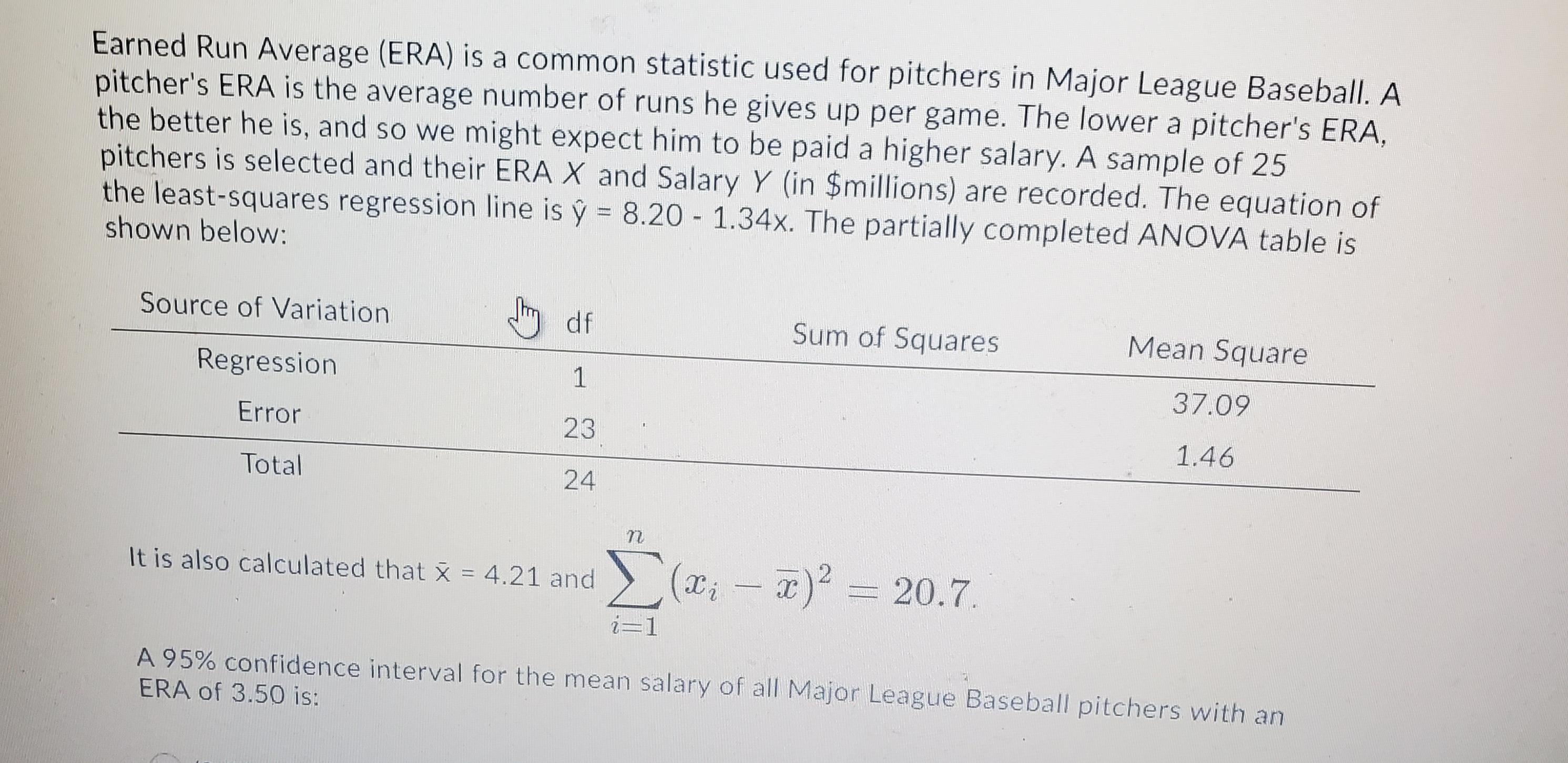
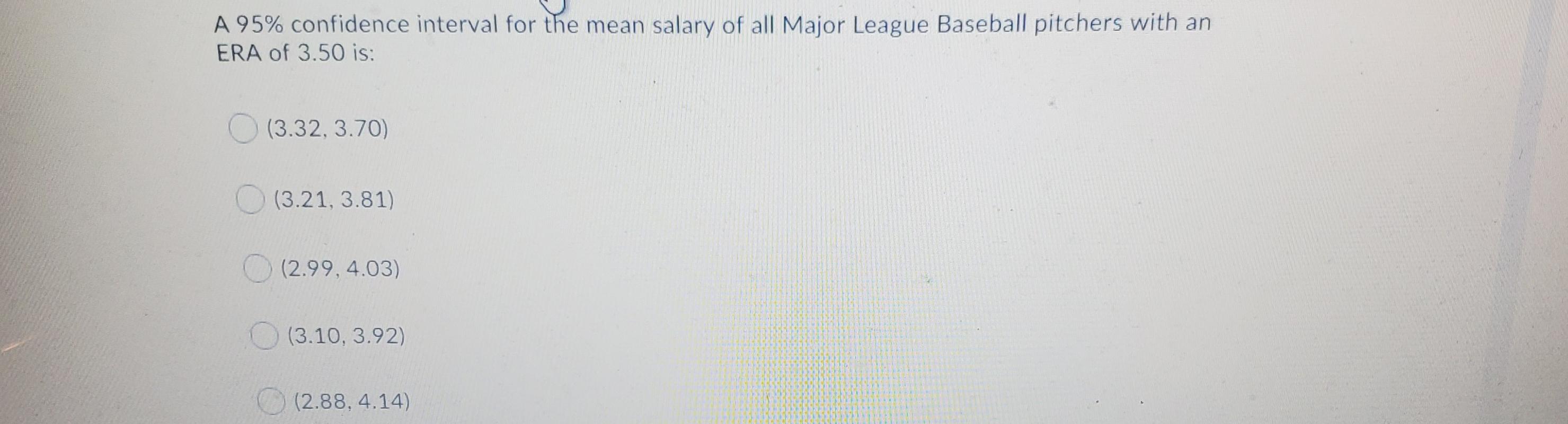
Earned Run Average (ERA) is a common statistic used for pitchers in Major League Baseball. A pitcher's ERA is the average number of runs he gives up per game. The lower a pitcher's ERA, the better he is, and so we might expect him to be paid a higher salary. A sample of 25 pitchers is selected and their ERA X and Salary Y (in $millions) are recorded. The equation of the least-squares regression line is y = 8.20- 1.34x. The partially completed ANOVA table is shown below: Source of Variation df Sum of Squares Mean Square Regression 1 37.09 Error 23 1.46 Total 24 It is also calculated that X = 4.21 and > (; - ) = 20.7 i=1 A 95% confidence interval for the mean salary of all Major League Baseball pitchers with an ERA of 3.50 is: A 95% confidence interval for the mean salary of all Major League Baseball pitchers with an ERA of 3.50 is: (3.32, 3.70) O(3.21, 3.81) (2.99, 4.03) (3.10, 3.92) (2.88, 4.14)