


Econ assignment.
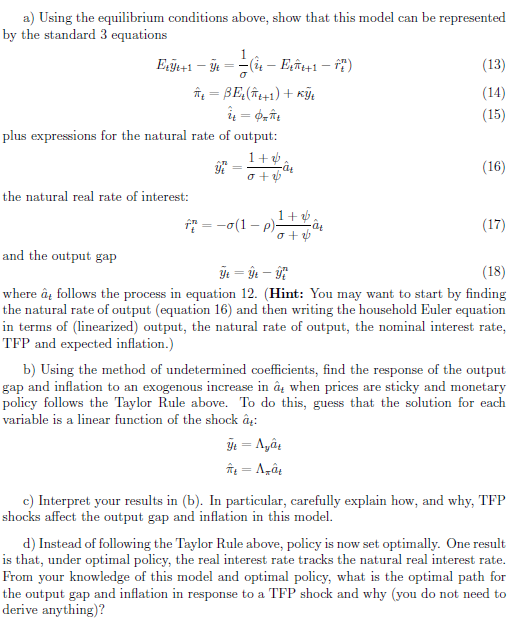
Question 1 {EH points] Consider the MortensenPissarides model in continuous time. Labor force is nor malized to l. Unemployed workers, with measure is E l, and rms with one vacancy each and total measure tr search for each other1 and e is determined endogenously by free entry. A CH5 matching function1 m[u,v}, brings together unemployed workers and vacant rms; In is increasing in both arguments. As is standard, let I? E rfu denote the market tightness and qIIH] = info the arrival rate of unemployed workers to the typical rm. 'What is dilterent here compared to the baseline model is that a "'match'1 and a \"productive job\" are not equivalent by default. 1v'v'hen a worker and a vacant rm meet, the rm must train the worker before she can start producing. A. formed match turns into a productive job at a stochastic rate, a. E [I], +oo}, so that Us. can be thought of as the average time necessary for the training to he completed. Assume that the rm and the worker determine the wage level when they rst meet [i.e., even before training starts}, through Nash bargaining, with ,8 E [l], 1] representing the worker's power. However, the wage upon which they have agreed will only be paid to the worker when she starts producing.1 To close the model1 we will make a few more standard assumptions. The output of a productive job is ,p :2:- [l per unit of time, and while a rm is searching for a worker it has to pay a search [or recruiting] cost, pc 2.: [1, per unit of time. Firms that are training their workers do not pay this cost [they are done recruiting}. Productive jobs are exogenously destroyed at rate J. 3' B {only productive jobs are subject to this shock; matches at the training stage cannot be terminated]. all agents discount Future at the rate r 2.:- D, and unemployed workers enjoy a benet 3 Lt:- I] per unit of time. Ii"4|"_hile at the training stage the worker does not receive an unemployment benet [a trainee is not unemployed]. a} Dene the value Function of the typical rm for all the possible states of the world it may nd itself in. b} Do the same for the typical worker. c] Combine the free entry condition with the expressions you provided in part {a} in order to derive thejob creation {JG} curve of this economy. :1} Using the same methodology as in the lectures {adjusted to accommodate the differences in the new environment}, derive the wage curve [WC] for this economy. a} Write down the household's problem in recursive form and write dorm the rm's maximization problem. Derive the household's rst order conditions and the rm's optimal hiring rules b} Carefully dene a recursive competitive equilibrium. Take care to distinguish between the aggregate and individual state variables and explain any market clearing conditions. c] By linearizing the equilibrium condition governing the optimal degree of utiliza- tion, show that 9' mpg [1 [as + E1 MM] [3} ire=i+ois+Elolfire+ where E = a: and 15" and 15'" refer to the rst and second derivatives of the 5 Function with respect to an in steady state. As usual, variablcs 1with a hat denote percentage deviations From steady state. {H.int: once you have linearized the equilibrium condition governing the optimal degree of utilization, you can combine this with the linearized production function in: = s + mt + oh +[1 olfliri]. d} Discuss how the addition of variable capital utilization helps the REC model explain the business cycle [acts in the data? If you can, explain why in the limit 5 = so and E = I] correspond to the cases of no variable capital utilisation and Full variable capital utilization. \fa} Using the equilibrium conditions above1 show that this model can be represented by the standard 3 equations 1 ea: a = 3:3: ea: fr} :13} "IT: =13EE5F+11+Ka {14} it, = (5,151 {15} plus expressions for the natural rate of output: l+w s=,+,,ai :16} the natural real rate of interest: 1 + 'qu o' + if! " = -0E1 p} at {1?} and the output gap if: = if: - if? {13} where Enc follows the process in equation 12. (Hint: 1i'ou may want to start by nding the natural rate of output {equation 15} and then writing the household Euler equation in terms of {linearized} output, the natural rate of output, the nominal interest rate1 TFP and expected inflation} b} Using the method of undetermined meflicicnts, nd the response of the output gap and ination to an exogenous increase in El; when prices are sticky and monetary policy follows the Taylor Rule above. To do this, guess that the solution for each variable is a linear function of the shock of: if: = hut}: \"Pr: = air c] Interpret your results in {b} In particular, carefully explain how1 and why, TFP shocks affect the output gap and ination in this model. d} Instead of following the Taylor Rule above, policy is now set optimally. Cine result is that, under optimal policy, the real interest rate tracks the natural real interest rate. From your knowledge of this model and optimal policy, what is the optimal path for the output gap and ination in response to a TFF' shock and why {you do not need to derive anything}?
















