NB: Titles: Price (p), Quantity (Q), Elasticity (????????), Absolute value of Elasticity (|????????|), Type of elasticity (Elastic, Unit-Elastic, Inelastic), Total Revenue (???????? =???? × ????) and Marginal Revenue (MR1). You will need to create a spreadsheet using Microsoft Excel (or any other spreadsheet software) that matches the one below.

Price (P)	Quantity (Q)	Elasticity	Absolute value of Elasticity	Type of Elasticity	TR	MR1
5
10
15
20
25
30
35
40
45
50
55
60
65
70
75

  

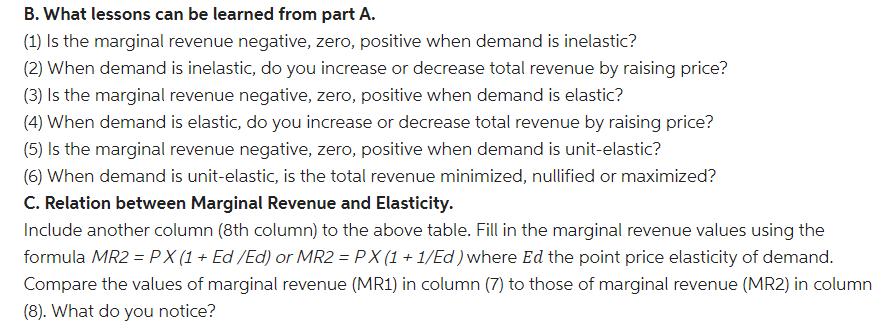
1. Q = 160 - 2p is the equation for the demand function of a good Z, where p is the price in dollars per unit of good Z and Q is the quantity demanded of good Z in million per year. Sometimes, it is convenient to rewrite a demand function with price on the left side. This relationship is referred to as the inverse demand function. Therefore, the inverse demand function for good Z is p = 80-1/2Q. For a linear inverse demand p =a - bQ, the marginal revenue is given by MR = a - 2bQ. Therefore, the marginal revenue of good Z is given by MR1 = 80 - Q. This is denoted MR1 because another marginal revenue (MR2) will be introduced in part C of this problem. Use the point price elasticity for the following questions. A. Calculate the price elasticity of demand for each and every price between $5 and $75. Round the elasticity to four digits after the decimal point in the third column. Then, fill in the other columns using the appropriate equations and the equations given above. NB: Titles: Price (p), Quantity (Q), Elasticity (Ed), Absolute value of Elasticity (Ed), Type of elasticity (Elastic, Unit-Elastic, Inelastic), Total Revenue (TR =p x Q) and Marginal Revenue (MR1). You will need to create a spreadsheet using Microsoft Excel (or any other spreadsheet software) that matches the one below. 1. Q = 160 - 2p is the equation for the demand function of a good Z, where p is the price in dollars per unit of good Z and Q is the quantity demanded of good Z in million per year. Sometimes, it is convenient to rewrite a demand function with price on the left side. This relationship is referred to as the inverse demand function. Therefore, the inverse demand function for good Z is p = 80-1/2Q. For a linear inverse demand p =a - bQ, the marginal revenue is given by MR = a - 2bQ. Therefore, the marginal revenue of good Z is given by MR1 = 80 - Q. This is denoted MR1 because another marginal revenue (MR2) will be introduced in part C of this problem. Use the point price elasticity for the following questions. A. Calculate the price elasticity of demand for each and every price between $5 and $75. Round the elasticity to four digits after the decimal point in the third column. Then, fill in the other columns using the appropriate equations and the equations given above. NB: Titles: Price (p), Quantity (Q), Elasticity (Ed), Absolute value of Elasticity (Ed), Type of elasticity (Elastic, Unit-Elastic, Inelastic), Total Revenue (TR =p x Q) and Marginal Revenue (MR1). You will need to create a spreadsheet using Microsoft Excel (or any other spreadsheet software) that matches the one below. 1. Q = 160 - 2p is the equation for the demand function of a good Z, where p is the price in dollars per unit of good Z and Q is the quantity demanded of good Z in million per year. Sometimes, it is convenient to rewrite a demand function with price on the left side. This relationship is referred to as the inverse demand function. Therefore, the inverse demand function for good Z is p = 80-1/2Q. For a linear inverse demand p =a - bQ, the marginal revenue is given by MR = a - 2bQ. Therefore, the marginal revenue of good Z is given by MR1 = 80 - Q. This is denoted MR1 because another marginal revenue (MR2) will be introduced in part C of this problem. Use the point price elasticity for the following questions. A. Calculate the price elasticity of demand for each and every price between $5 and $75. Round the elasticity to four digits after the decimal point in the third column. Then, fill in the other columns using the appropriate equations and the equations given above. NB: Titles: Price (p), Quantity (Q), Elasticity (Ed), Absolute value of Elasticity (Ed), Type of elasticity (Elastic, Unit-Elastic, Inelastic), Total Revenue (TR =p x Q) and Marginal Revenue (MR1). You will need to create a spreadsheet using Microsoft Excel (or any other spreadsheet software) that matches the one below. 1. Q = 160 - 2p is the equation for the demand function of a good Z, where p is the price in dollars per unit of good Z and Q is the quantity demanded of good Z in million per year. Sometimes, it is convenient to rewrite a demand function with price on the left side. This relationship is referred to as the inverse demand function. Therefore, the inverse demand function for good Z is p = 80-1/2Q. For a linear inverse demand p =a - bQ, the marginal revenue is given by MR = a - 2bQ. Therefore, the marginal revenue of good Z is given by MR1 = 80 - Q. This is denoted MR1 because another marginal revenue (MR2) will be introduced in part C of this problem. Use the point price elasticity for the following questions. A. Calculate the price elasticity of demand for each and every price between $5 and $75. Round the elasticity to four digits after the decimal point in the third column. Then, fill in the other columns using the appropriate equations and the equations given above. NB: Titles: Price (p), Quantity (Q), Elasticity (Ed), Absolute value of Elasticity (Ed), Type of elasticity (Elastic, Unit-Elastic, Inelastic), Total Revenue (TR =p x Q) and Marginal Revenue (MR1). You will need to create a spreadsheet using Microsoft Excel (or any other spreadsheet software) that matches the one below. 1. Q = 160 - 2p is the equation for the demand function of a good Z, where p is the price in dollars per unit of good Z and Q is the quantity demanded of good Z in million per year. Sometimes, it is convenient to rewrite a demand function with price on the left side. This relationship is referred to as the inverse demand function. Therefore, the inverse demand function for good Z is p = 80-1/2Q. For a linear inverse demand p =a - bQ, the marginal revenue is given by MR = a - 2bQ. Therefore, the marginal revenue of good Z is given by MR1 = 80 - Q. This is denoted MR1 because another marginal revenue (MR2) will be introduced in part C of this problem. Use the point price elasticity for the following questions. A. Calculate the price elasticity of demand for each and every price between $5 and $75. Round the elasticity to four digits after the decimal point in the third column. Then, fill in the other columns using the appropriate equations and the equations given above. NB: Titles: Price (p), Quantity (Q), Elasticity (Ed), Absolute value of Elasticity (Ed), Type of elasticity (Elastic, Unit-Elastic, Inelastic), Total Revenue (TR =p x Q) and Marginal Revenue (MR1). You will need to create a spreadsheet using Microsoft Excel (or any other spreadsheet software) that matches the one below.