Answered step by step
Verified Expert Solution
Question
1 Approved Answer
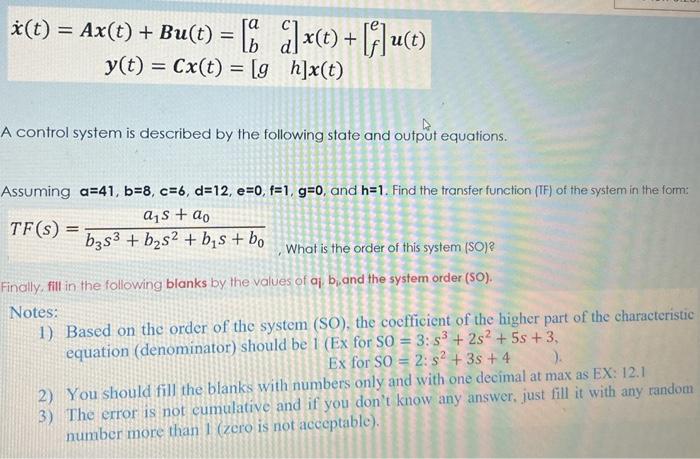
[ egin{array}{c} dot{x}(t)=A oldsymbol{x}(t)+oldsymbol{B} oldsymbol{u}(t)=left[egin{array}{ll} a & c b & d end{array} ight] oldsymbol{x}(t)+left[egin{array}{l} e f end{array} ight] oldsymbol{u}(t) oldsymbol{y}(t)=oldsymbol{C} oldsymbol{x}(t)=left[egin{array}{ll} g
[ egin{array}{c} dot{x}(t)=A oldsymbol{x}(t)+oldsymbol{B} oldsymbol{u}(t)=left[egin{array}{ll} a & c \ b & d end{array} ight] oldsymbol{x}(t)+left[egin{array}{l} e \ f end{array} ight] oldsymbol{u}(t) \ oldsymbol{y}(t)=oldsymbol{C} oldsymbol{x}(t)=left[egin{array}{ll} g & h end{array} ight] oldsymbol{x}(t) end{array} ] A control system is described by the following state and output equations. Assuming ( a=41, b=8, c=6, d=12, e=0, f=1, g=0 ), and ( h=1 ). Find the transfer function (IF) of the system in the form: [ T F(s)=rac{a_{1} s+a_{0}}{b_{3} s^{3}+b_{2} s^{2}+b_{1} s+b_{0}} ] What is the order of this system ( (mathrm{SO}) ) ? Finally, fill in the following blanks by the values of ( a_{i}, b_{i} ), and the system order (SO). Notes: 1) Based on the order of the system (SO), the coefficient of the higher part of the characteristic equation (denominator) should be 1 (Ex for ( mathrm{SO}=3: s^{3}+2 s^{2}+5 s+3 ), [ ext { Ex for } left.S O=2: s^{2}+3 s+4 quad ight) ext {. } ] 2) You should fill the blanks with numbers only and with one decimal at max as EX: 12.1 3) The error is not cumulative and if you don't know any answer, just fill it with any random number more than 1 (zero is not acceptable).
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


