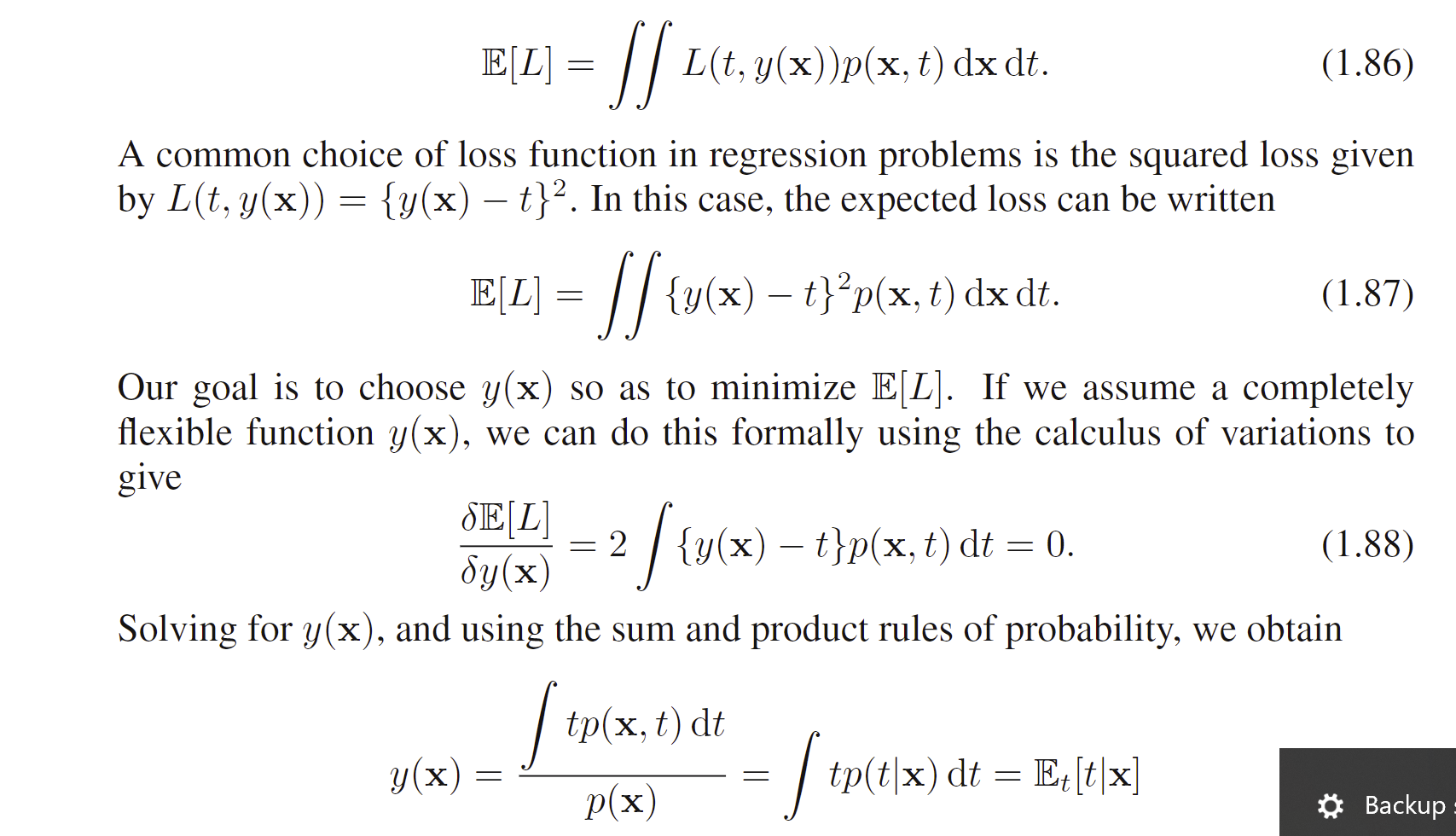
E[L] = [] L(t, y(x))p(x, t) dx dt. (1.86) A common choice of loss function in regression problems is the squared loss given by L(t, y(x)) = {y(x) - t}. In this case, the expected loss can be written E[L] = [] {y(x) t}p(x, t) dx dt. - (1.87) Our goal is to choose y(x) so as to minimize E[L]. If we assume a completely flexible function y(x), we can do this formally using the calculus of variations to give SE[L] Sy(x) [ = 2 {y(x) - t}p(x, t) dt = 0. (1.88) Solving for y(x), and using the sum and product rules of probability, we obtain tp(x, t) dt [tp y(x) = p(x) = [ tp(t|x) dt: tp(t|x) dt = Et[t|x Backup