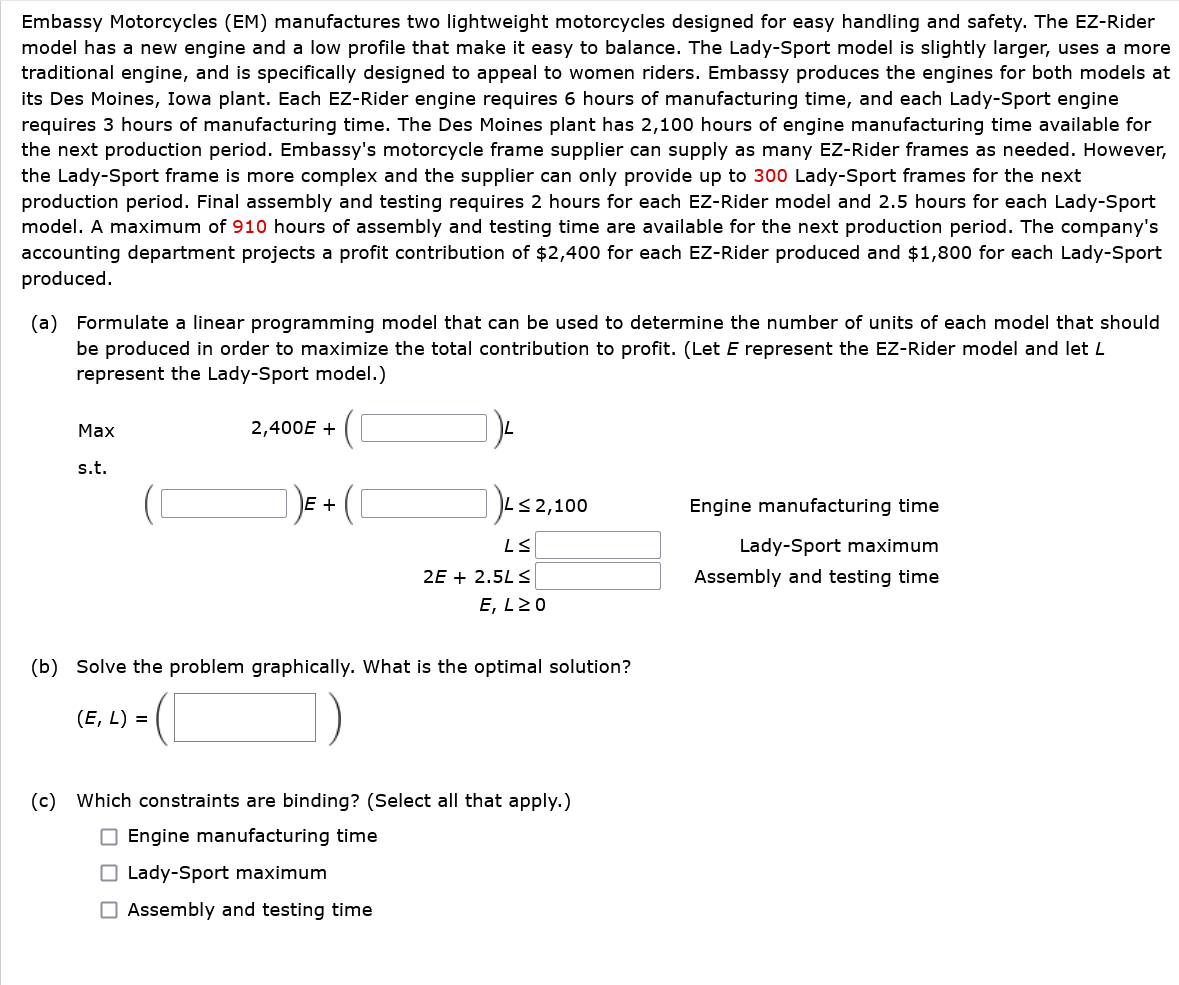
Embassy Motorcycles (EM) manufactures two lightweight motorcycles designed for easy handling and safety. The EZ-Rider model has a new engine and a low profile that make it easy to balance. The Lady-Sport model is slightly larger, uses a more traditional engine, and is specifically designed to appeal to women riders. Embassy produces the engines for both models at its Des Moines, Iowa plant. Each EZ-Rider engine requires 6 hours of manufacturing time, and each Lady-Sport engine requires 3 hours of manufacturing time. The Des Moines plant has 2,100 hours of engine manufacturing time available for the next production period. Embassy's motorcycle frame supplier can supply as many EZ-Rider frames as needed. However, the Lady-Sport frame is more complex and the supplier can only provide up to 300 Lady-Sport frames for the next production period. Final assembly and testing requires 2 hours for each EZ-Rider model and 2.5 hours for each Lady-Sport model. A maximum of 910 hours of assembly and testing time are available for the next production period. The company's accounting department projects a profit contribution of $2,400 for each EZ-Rider produced and $1,800 for each Lady-Sport produced. (a) Formulate a linear programming model that can be used to determine the number of units of each model that should be produced in order to maximize the total contribution to profit. (Let E represent the EZ-Rider model and let L represent the Lady-Sport model.) Max s.t. 2,400E+()L )E+(L2E+2.5LE,L2,1000 Engine manufacturing time Lady-Sport maximum Assembly and testing time (b) Solve the problem graphically. What is the optimal solution? (E,L)=() (c) Which constraints are binding? (Select all that apply.) Engine manufacturing time Lady-Sport maximum Assembly and testing time Embassy Motorcycles (EM) manufactures two lightweight motorcycles designed for easy handling and safety. The EZ-Rider model has a new engine and a low profile that make it easy to balance. The Lady-Sport model is slightly larger, uses a more traditional engine, and is specifically designed to appeal to women riders. Embassy produces the engines for both models at its Des Moines, Iowa plant. Each EZ-Rider engine requires 6 hours of manufacturing time, and each Lady-Sport engine requires 3 hours of manufacturing time. The Des Moines plant has 2,100 hours of engine manufacturing time available for the next production period. Embassy's motorcycle frame supplier can supply as many EZ-Rider frames as needed. However, the Lady-Sport frame is more complex and the supplier can only provide up to 300 Lady-Sport frames for the next production period. Final assembly and testing requires 2 hours for each EZ-Rider model and 2.5 hours for each Lady-Sport model. A maximum of 910 hours of assembly and testing time are available for the next production period. The company's accounting department projects a profit contribution of $2,400 for each EZ-Rider produced and $1,800 for each Lady-Sport produced. (a) Formulate a linear programming model that can be used to determine the number of units of each model that should be produced in order to maximize the total contribution to profit. (Let E represent the EZ-Rider model and let L represent the Lady-Sport model.) Max s.t. 2,400E+()L )E+(L2E+2.5LE,L2,1000 Engine manufacturing time Lady-Sport maximum Assembly and testing time (b) Solve the problem graphically. What is the optimal solution? (E,L)=() (c) Which constraints are binding? (Select all that apply.) Engine manufacturing time Lady-Sport maximum Assembly and testing time







