Employee	Work Hours Missed	Annual Wages
1	155	8.8
2	127	8.3
3	72	10
4	6	15.8
5	36	14.5
6	56	21
7	63	10.8
8	11	17.2
9	49	12.8
10	485	12.2
11	79	9.7
12	91	10.2
13	191	7.8
14	34	11.5
15	82	10.9

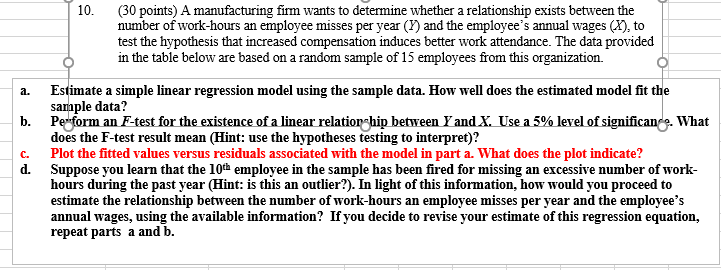
10. (30 points) A manufacturing firm wants to determine whether a relationship exists between the number of work-hours an employee misses per year (Y) and the employee's annual wages (X), to test the hypothesis that increased compensation induces better work attendance. The data provided in the table below are based on a random sample of 15 employees from this organization. a. b. Estimate a simple linear regression model using the sample data. How well does the estimated model fit the sample data? Pe-form an F-test for the existence of a linear relatior hip between Y and X. Use a 5% level of significanc. What does the F-test result mean (Hint: use the hypotheses testing to interpret)? Plot the fitted values versus residuals associated with the model in part a. What does the plot indicate? Suppose you learn that the 10th employee in the sample has been fired for missing an excessive number of work- hours during the past year (Hint: is this an outlier?). In light of this information, how would you proceed to estimate the relationship between the number of work-hours an employee misses per year and the employee's annual wages, using the available information? If you decide to revise your estimate of this regression equation, repeat parts a and b. 10. (30 points) A manufacturing firm wants to determine whether a relationship exists between the number of work-hours an employee misses per year (Y) and the employee's annual wages (X), to test the hypothesis that increased compensation induces better work attendance. The data provided in the table below are based on a random sample of 15 employees from this organization. a. b. Estimate a simple linear regression model using the sample data. How well does the estimated model fit the sample data? Pe-form an F-test for the existence of a linear relatior hip between Y and X. Use a 5% level of significanc. What does the F-test result mean (Hint: use the hypotheses testing to interpret)? Plot the fitted values versus residuals associated with the model in part a. What does the plot indicate? Suppose you learn that the 10th employee in the sample has been fired for missing an excessive number of work- hours during the past year (Hint: is this an outlier?). In light of this information, how would you proceed to estimate the relationship between the number of work-hours an employee misses per year and the employee's annual wages, using the available information? If you decide to revise your estimate of this regression equation, repeat parts a and b