

Enter the correct answer only plz
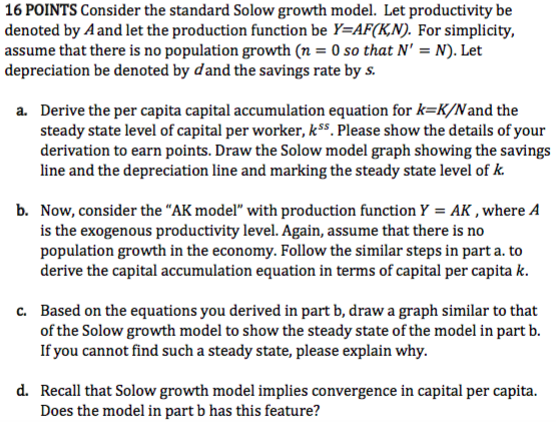
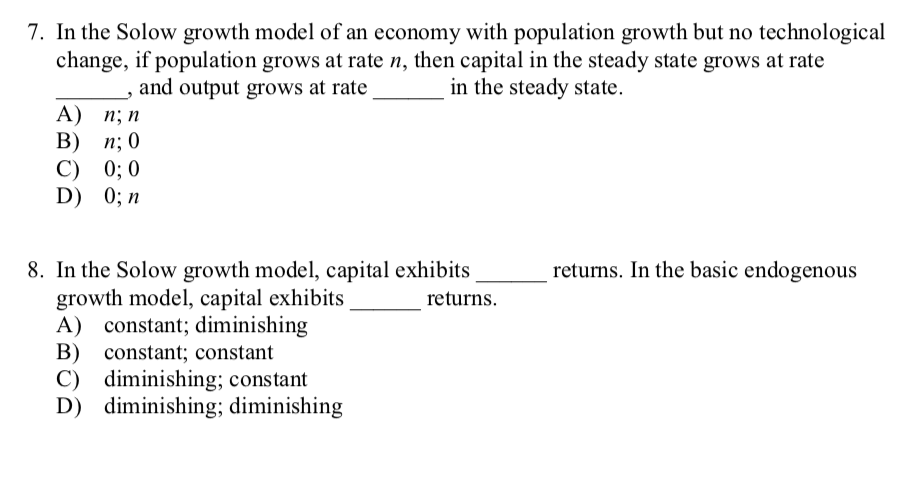
16 POINTS Consider the standard Solow growth model. Let productivity be denoted by A and let the production function be Y=AF(KN). For simplicity, assume that there is no population growth (n = 0 so that N' = N). Let depreciation be denoted by dand the savings rate by s. a. Derive the per capita capital accumulation equation for =K/Nand the steady state level of capital per worker, $s. Please show the details of your derivation to earn points. Draw the Solow model graph showing the savings line and the depreciation line and marking the steady state level of k. b. Now, consider the "AK model" with production function Y = AK , where A is the exogenous productivity level. Again, assume that there is no population growth in the economy. Follow the similar steps in part a. to derive the capital accumulation equation in terms of capital per capita k. c. Based on the equations you derived in part b, draw a graph similar to that of the Solow growth model to show the steady state of the model in part b. If you cannot find such a steady state, please explain why. d. Recall that Solow growth model implies convergence in capital per capita. Does the model in part b has this feature?7. In the Solow growth model of an economy with population growth but no technological change, if population grows at rate a, then capital in the steady state grows at rate and output grows at rate in the steady state. A) n; n B) n; 0 C) 0; 0 D) 0; n 8. In the Solow growth model, capital exhibits growth model, capital exhibits returns. A) constant; diminishing B) constant; constant C) diminishing; constant D) diminishing; diminishing returns. In the basic endogenous {a} (hi {a} (hi (3} (b) Assume the demand for good K is fog linear: with (it: = c +(Pxim. If the quantity demanded is 'IEEI when Pi? is $21112]. what is the quantity demanded when P: rises to $4.5? HINT: Begin by finding the value of the constant c. Using your answer in part (a). find the precise price elasticity between SEED and $4.50. Assume that demand for good it is a function of its price {F's}. the price of good '1' (Pv), and Income (M) as follows: Q: = {(lvl + Pv}!(2Pv}} - 1 Given the following values for these variables: P1=2;Pv=5;lvl=51 lCalculate the {own} price elasticity En Calculate the cross price elasticity Exv Calculate the income elasticity Em Assume that demand for a good {[1] is a function of its price (P), as follows 0 = s- P, for i] -: P s e, where sis Euler's number (a constant 2 2.?1323). If the equilibrium price P' = 31: What is the price elasticity of demand (= dedF' . PM} in equilibrium? What is the dollar value of consumer surplus (CS) in equilibrium? HINT:e P] = [eF' - isF'E] What is the total dollar benefit to buyers (C3 + total paid] in equilibrium? Eh} {a} (h) A city government is considering renting space in an all day parking garage for its 10D employees. The government estimates these employees' demand function for parking spaces is 15D EDP {F' a $1}. where F' is the per-day price of parking, and the city will pass on the cost. If the city needs n_ot charge each of its employees the same price for a parking space, what is the maximum amount the city could pay for the 1m spaces, and what would be the average cost per space? Assume the employees' union insists that per their contract each employee must be charged the same price for parking. and the city's response is to intend charging the price that maximizes its parking fee revenue. What price per space would the city charge under this circumstance. and how much less total dollar benefit would the employees receive? You are shown four envelopes. Envelope A contains $1,5IJEI; envelopes B, C and D contain uncertain amounts of money but with the following probabilities: Envelope E. -- $5,D with a 1i] percent probability. $1.5D with an E19 percent probability, and $50 with a one percent probability. Envelope C $1,5DD with an 11 percent probability and $50 with an 39 percent probability. Envelope D $5,1'l with a 10 percent probability and $50 with a 912] percent probability. Show mathematically that if envelope A has a higher expectedoogrthan envelope B, then it must be true that envelope C has a higher expectedowiifgxthan envelope D. (Note: For a given value of lvl, U{lv1) is a fixed but unknown number; [it] NUT assume um; = lvl!!} A graduating MBA student has job offers from two brokerage firms. Firm #1 pays a straight salary of WEDGE} (but no commission bonuses). Firm #2 pays a salary of sauce plus a commission bonus, with a fixed bonus schedule based on annual sales; the potential commission bonus for firm #E'sjob is as follows: $1 ll with a probability of 11%, $5lll with a probability of 33%. SEDJJEID with a probability of 5'35, and zero with a probability of 1%. What is the expected monetary value of Firm #E'sjob? The student claims to be :hofffemnrbetween the two job offers. If this is true, is the student risk averse. risk loving, or risk neutral. and why














