Question: erences over two periods, Consider an agent (Anna) with pref- U(x1, x2) = u(x1) + Bu(x2) Anna is endowed with 1 unit of x1,

erences over two periods, Consider an agent (Anna) with pref- U(x1, x2) = u(x1) + Bu(x2) Anna is endowed with 1 unit of x1,

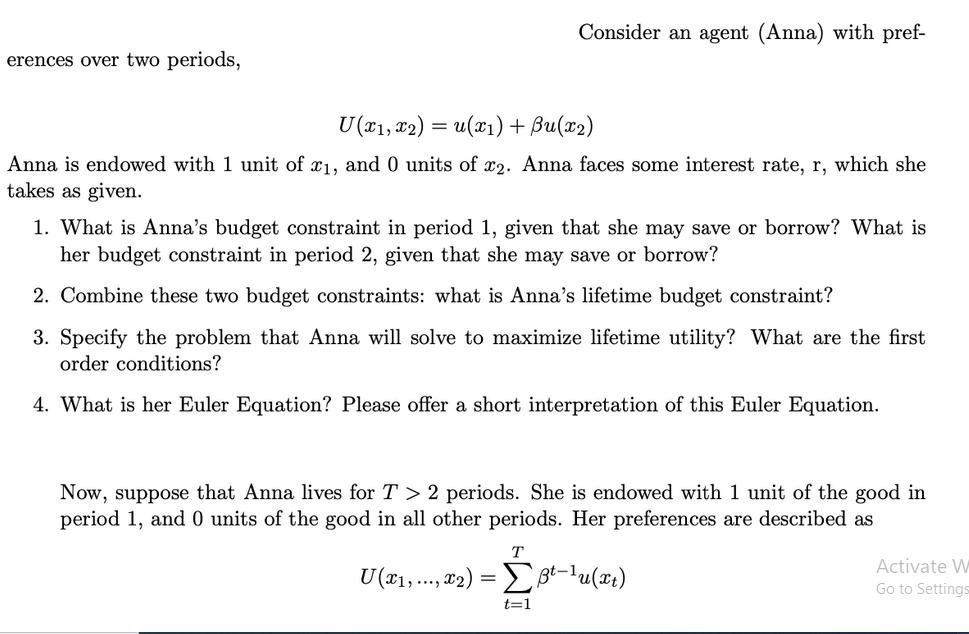
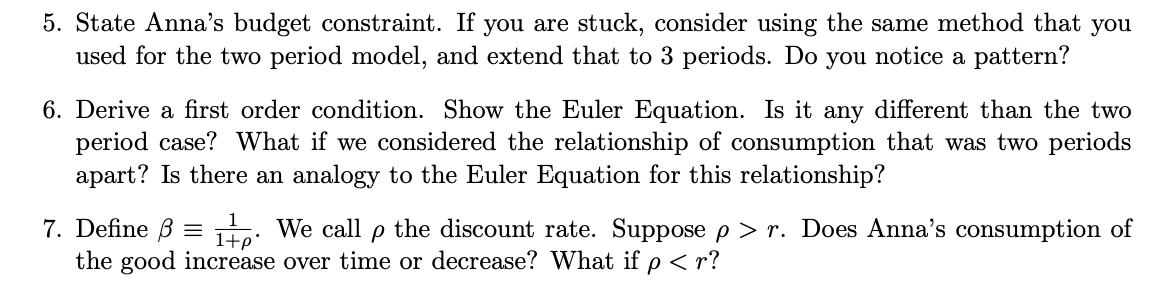
erences over two periods, Consider an agent (Anna) with pref- U(x1, x2) = u(x1) + Bu(x2) Anna is endowed with 1 unit of x1, and 0 units of x2. Anna faces some interest rate, r, which she takes as given. 1. What is Anna's budget constraint in period 1, given that she may save or borrow? What is her budget constraint in period 2, given that she may save or borrow? 2. Combine these two budget constraints: what is Anna's lifetime budget constraint? 3. Specify the problem that Anna will solve to maximize lifetime utility? What are the first order conditions? 4. What is her Euler Equation? Please offer a short interpretation of this Euler Equation. Now, suppose that Anna lives for T > 2 periods. She is endowed with 1 unit of the good in period 1, and 0 units of the good in all other periods. Her preferences are described as T U(x1,...,x2) = t u(x) t=1 Activate W Go to Settings 5. State Anna's budget constraint. If you are stuck, consider using the same method that you used for the two period model, and extend that to 3 periods. Do you notice a pattern? 6. Derive a first order condition. Show the Euler Equation. Is it any different than the two period case? What if we considered the relationship of consumption that was two periods apart? Is there an analogy to the Euler Equation for this relationship? 1 . 7. Define = We call p the discount rate. Suppose p > r. Does Anna's consumption of the good increase over time or decrease? What if p < r?

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Business Communication Questions!

Question 1: What are the variable names and which measures do they represent? Can someone please help me answer this ? Variables being - Willingness to try, buy and pay for plant-based and insect...

Q1. You have identified a market opportunity for home media players that would cater for older members of the population. Many older people have difficulty in understanding the operating principles...

CANMNMM January of this year. (a) Each item will be held in a record. Describe all the data structures that must refer to these records to implement the required functionality. Describe all the...

Probability and Statistics - Problem Set c Keith M. Chugg October 2, 2015 1 Preliminaries, Combinatorics, Set Probability 1.1. A number of bats are in a cave. 2 bats can see out of their left eye. 3...

ecoty 1. Suppose that a consumer with utility U(x1, 12) is given an initial endowment 71, 12 so that his total budget set is pill , p232 (a) Derive a formula for the slutsky equation with endowments...

Let A, B be sets. Define: (a) the Cartesian product (A B) (b) the set of relations R between A and B (c) the identity relation A on the set A [3 marks] Suppose S, T are relations between A and B, and...

make sure to tackle al (i) According to the Ramsey model, what is the impact of a "fiscal stimulus" on investment, consumption and output if the stimulus is financed with lump-sum taxes? What if it...

business quiz Exercises 7 for half our current forests to be cleared? (Use annual compounding and solve for the fewest number of whole years.) Exercise 1.8 (Moderate) World population was about 679...

give correct only678 16. Consider three stocks: Q. R and S. Beta STD (annual) Forecast for Nov 2008 Dividend Stock Price Q 0.45 35% $0.50 $45 R 1.45 10% 0 $75 S -0.20 40% $1.00 $20 33 2008, Andrew W....

12580 Consider an exchange economy with two goods, ale and bread. There are two individuals, Ann and Bob, whose initial endowments are as fol lows: Ann has 40 units of ale and 20 units of bread, and...

Question) Differentiate: y = (e x+2 ) 2

Would a profit-maximizing firm continue to operate if the price in the market fell below its average cost of production in the short run?

Solve for the portion. Note: Round to the nearest tenth. 2 5 % o f 4 1 0 ,

The financial statements of Marks and Spencer plc (M&S) are available at the books companion website or can be accessed at corporate.marksandspencer....

Venice InLine, Inc., was founded by Russ Perez to produce a specialized in-line skate he had designed for doing aerial tricks. Up to this point, Russ has financed the company with his own sayings and...

(Amortization ScheduleEffective-Interest) Assume the same information as E14-6. Set up a schedule of interest expense and discount amortization under the effective-interest method.

Debt Service Fund Trial Balance. Following is Franklin Countys debt service fund pre-closing trial balance for the fiscal year ended June 30, 2011. Required Using information provided by the trial...

Calculate the torque (magnitude and direction) about point 0 due to the force F in each of the cases sketched in Fig. 10.37. In each case, the force F and the rod both lie in the plane of the page,...

The return of goods to the supplier will affect the purchase account. Question 27Answer True False

Superstores like Costco and Sams Club serves as wholesalers to businesses but also target consumers who are willing to pay a fixed fee in order to get access to the lower wholesale prices offered in...

In Chapter 22, we worked with models in which high and low cost customers compete for insurance. Consider the level x of health insurance that consumers might choose to buy, with higher levels of x...

Investor Tastes over Risk and Return: Suppose you are considering where to Invest money for the future. A: Like most investors, you care about the expected return on your investment as well as the...

The San Luis Obispo, California, Transit Program provides daily fixed-route transit service to the general public within the city limits and to Cal Poly State Universitys staff and students. The most...

Revisit Problem 6-55, but examine whether it would matter when you started monitoring the 911 calls if the time between occurrences had a uniform distribution with a mean of 2 and a range of 4. a....

Rolls-Royce PLC provides forecasts for the business jet market and covers the regional and major aircraft markets. In a recent release, Rolls-Royce indicated that in both North America and Europe the...