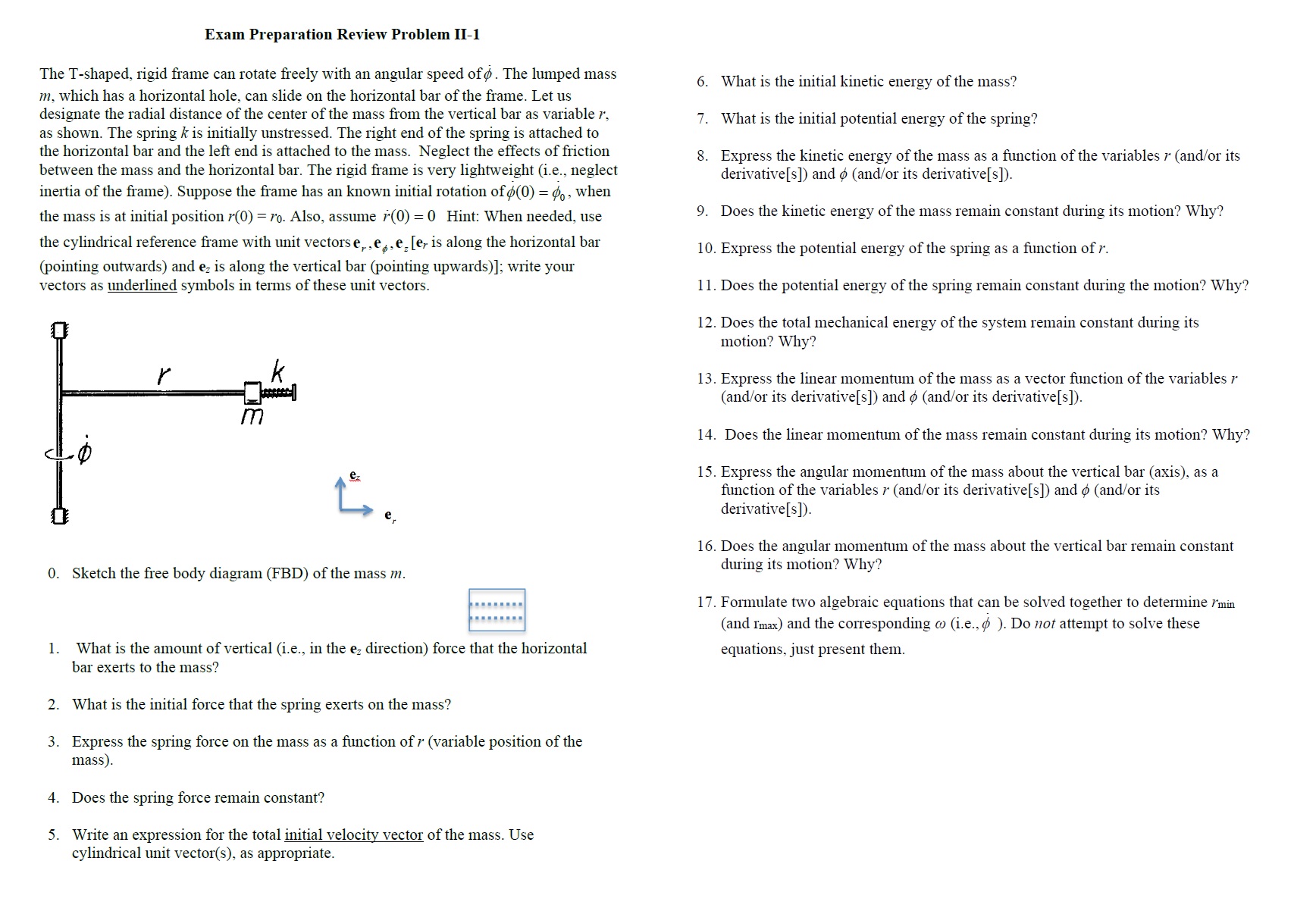
Exam Preparation Review Problem II-1 The T-shaped, rigid frame can rotate freely with an angular speed of . The lumped mass m, which has a horizontal hole, can slide on the horizontal bar of the frame. Let us designate the radial distance of the center of the mass from the vertical bar as variable r, as shown. The spring k is initially unstressed. The right end of the spring is attached to the horizontal bar and the left end is attached to the mass. Neglect the effects of friction between the mass and the horizontal bar. The rigid frame is very lightweight (i.e., neglect inertia of the frame). Suppose the frame has an known initial rotation of (0) = 0, when the mass is at initial position (0) = ro. Also, assume (0)=0 Hint: When needed, use the cylindrical reference frame with unit vectors e,,e,e, [e, is along the horizontal bar (pointing outwards) and ez is along the vertical bar (pointing upwards)]; write your vectors as underlined symbols in terms of these unit vectors. M ez L 0. Sketch the free body diagram (FBD) of the mass m. 1. What is the amount of vertical (i.e., in the ez direction) force that the horizontal bar exerts to the mass? 2. What is the initial force that the spring exerts on the mass? 3. Express the spring force on the mass as a function of r (variable position of the mass). 4. Does the spring force remain constant? 5. Write an expression for the total initial velocity vector of the mass. Use cylindrical unit vector(s), as appropriate. 6. What is the initial kinetic energy of the mass? 7. What is the initial potential energy of the spring? 8. Express the kinetic energy of the mass as a function of the variables 7 (and/or its derivative[s]) and 6 (and/or its derivative[s]). 9. Does the kinetic energy of the mass remain constant during its motion? Why? 10. Express the potential energy of the spring as a function of r. 11. Does the potential energy of the spring remain constant during the motion? Why? 12. Does the total mechanical energy of the system remain constant during its motion? Why? 13. Express the linear momentum of the mass as a vector function of the variables r (and/or its derivative[s]) and 6 (and/or its derivative[s]). 14. Does the linear momentum of the mass remain constant during its motion? Why? 15. Express the angular momentum of the mass about the vertical bar (axis), as a function of the variables (and/or its derivative[s]) and 6 (and/or its derivative[s]). 16. Does the angular momentum of the mass about the vertical bar remain constant during its motion? Why? 17. Formulate two algebraic equations that can be solved together to determine /min (and I'max) and the corresponding w (i.e., 6). Do not attempt to solve these equations, just present them.