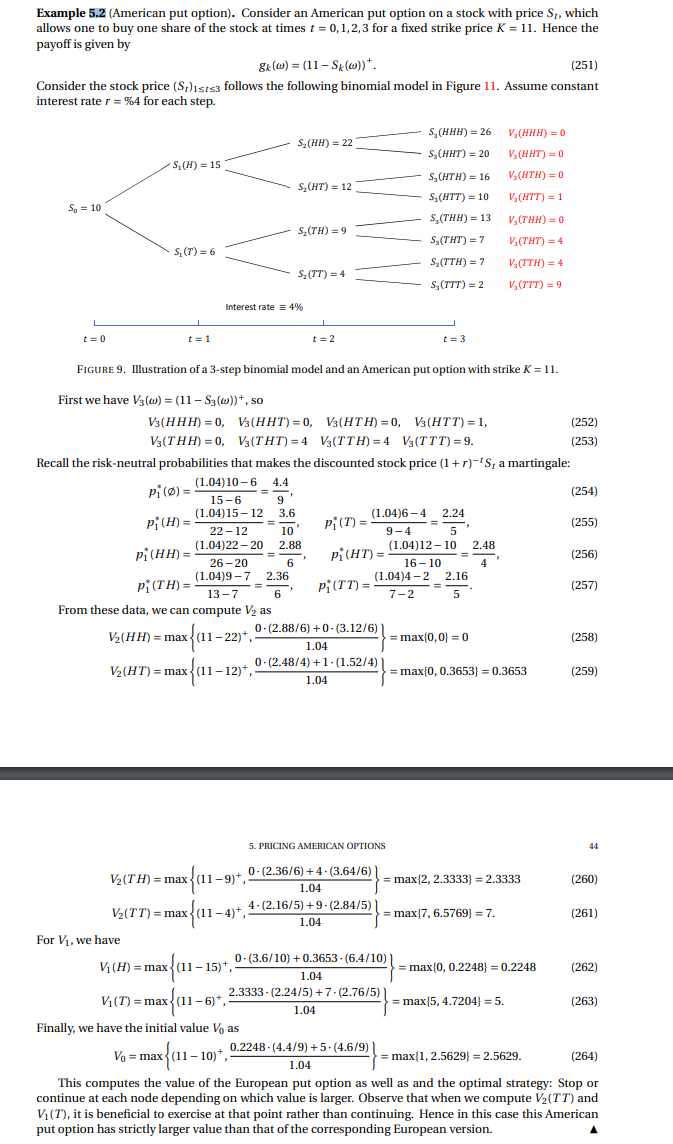
Example 5.2 (American put option). Consider an American put option on a stock with price S, which allows one to buy one share of the stock at times t = 0,1,2,3 for a fixed strike price K = 11. Hence the payoff is given by gx(w) = (11-Sk)) (251) Consider the stock price (St)ists follows the following binomial model in Figure 11. Assume constant interest rate r = %4 for each step. S(HH) = 22 S.(H) = 15 S(HT) = 12 Se = 10 S,(HHH) = 26 V (HHH) = 0 S (HHT) = 20 V(HHT)= 0 SCHTH) = 16 V (HTH) = 0 S(HTT) = 10 V.(HTT) = 1 S(THB) = 13 V, (THH) = 0 S(THT) = 7 V.(THT) = 4 STTH) = 7 VSCTTH) = 4 S, (TTT) = 2 V. (TTT) = 9 S(TH) = 9 S. (T) = 6 S. (77) = 4 Interest rate = 4% t=0 t = 1 t = 2 t = 3 FIGURE 9. Illustration of a 3-step binomial model and an American put option with strike K = 11. pi)= 9 First we have V3(W) = (11 - Sz(w)), so V3(HHH) = 0, 13(HHT) = 0, V3(HTH) = 0, V(HTT) = 1, (252) V3(THH) = 0, 13(THT)=4 V(TTH) = 4 13(TTT) = 9. (253) Recall the risk-neutral probabilities that makes the discounted stock price (1 + r)-', a martingale: (1.04)10-6 4.4 (254) 15-6 9 (1.04)15-12 3.6 (1.04)6-4 2.24 Pi (H) = pi(T) = (255) 10 9-4 5' ( 1.04)22-20 2.88 (1.04)12-10 2.48 pi(HH)= == pi(HT) (256) 26-20 6 16-10 4 (1.0479-7 2.36 (1.04)4-2 2.16 P(TH) = pi(TT) = (257) 13-7 7-2 5 From these data, we can compute V2 as V2(HH) = max{(11-22), 0.(2.88/6) +0-(3.12/6) = max(0,0) = 0 (258) 1.04 0.(2.48/4) +1-(1.52/4) V(HT) = max (11- (11-12), = max(0, 0.3653) = 0.3653 1.04 (259) 22-12 6 5. PRICING AMERICAN OPTIONS V2(TH) = max (11-9), (, 0-(2.36/6) +4.(3.64/6) = max(2, 2.3333) = 2.3333 (260) 1.04 max (11-4), V2(TT) = max 4.(2.16/5)+9. (2.84/5) = max{7, 6.5769} = 7. (261) 1.04 For Vi, we have 0.(3.6/10) +0.3653 -(6.4/10) V (H) = max (11-15), = max(0, 0.2248) = 0.2248 (262) 1.04 () = 2.3333. (2.24/5)+7.(2.76/5) 1.04 = max{5, 4.7204) = 5. Finally, we have the initial value V, as 0.2248.(4.4/9) + 5. (4.6/9) Vo = max (11-10) 1.04 = max(1, 2.5629) = 2.5629. This computes the value of the European put option as well as and the optimal strategy: Stop or continue at each node depending on which value is larger. Observe that when we compute V2(TT) and V. (T), it is beneficial to exercise at that point rather than continuing. Hence in this case this American put option has strictly larger value than that of the corresponding European version. (263) ax{11-15) {, (264) Exercise 2. In Example 5.2 in LN Chapter 2, compute the price V, of the European put option with the same payoff. Verify that the American version of the put option indeed has strictly larger value than the European version. Example 5.2 (American put option). Consider an American put option on a stock with price S, which allows one to buy one share of the stock at times t = 0,1,2,3 for a fixed strike price K = 11. Hence the payoff is given by gx(w) = (11-Sk)) (251) Consider the stock price (St)ists follows the following binomial model in Figure 11. Assume constant interest rate r = %4 for each step. S(HH) = 22 S.(H) = 15 S(HT) = 12 Se = 10 S,(HHH) = 26 V (HHH) = 0 S (HHT) = 20 V(HHT)= 0 SCHTH) = 16 V (HTH) = 0 S(HTT) = 10 V.(HTT) = 1 S(THB) = 13 V, (THH) = 0 S(THT) = 7 V.(THT) = 4 STTH) = 7 VSCTTH) = 4 S, (TTT) = 2 V. (TTT) = 9 S(TH) = 9 S. (T) = 6 S. (77) = 4 Interest rate = 4% t=0 t = 1 t = 2 t = 3 FIGURE 9. Illustration of a 3-step binomial model and an American put option with strike K = 11. pi)= 9 First we have V3(W) = (11 - Sz(w)), so V3(HHH) = 0, 13(HHT) = 0, V3(HTH) = 0, V(HTT) = 1, (252) V3(THH) = 0, 13(THT)=4 V(TTH) = 4 13(TTT) = 9. (253) Recall the risk-neutral probabilities that makes the discounted stock price (1 + r)-', a martingale: (1.04)10-6 4.4 (254) 15-6 9 (1.04)15-12 3.6 (1.04)6-4 2.24 Pi (H) = pi(T) = (255) 10 9-4 5' ( 1.04)22-20 2.88 (1.04)12-10 2.48 pi(HH)= == pi(HT) (256) 26-20 6 16-10 4 (1.0479-7 2.36 (1.04)4-2 2.16 P(TH) = pi(TT) = (257) 13-7 7-2 5 From these data, we can compute V2 as V2(HH) = max{(11-22), 0.(2.88/6) +0-(3.12/6) = max(0,0) = 0 (258) 1.04 0.(2.48/4) +1-(1.52/4) V(HT) = max (11- (11-12), = max(0, 0.3653) = 0.3653 1.04 (259) 22-12 6 5. PRICING AMERICAN OPTIONS V2(TH) = max (11-9), (, 0-(2.36/6) +4.(3.64/6) = max(2, 2.3333) = 2.3333 (260) 1.04 max (11-4), V2(TT) = max 4.(2.16/5)+9. (2.84/5) = max{7, 6.5769} = 7. (261) 1.04 For Vi, we have 0.(3.6/10) +0.3653 -(6.4/10) V (H) = max (11-15), = max(0, 0.2248) = 0.2248 (262) 1.04 () = 2.3333. (2.24/5)+7.(2.76/5) 1.04 = max{5, 4.7204) = 5. Finally, we have the initial value V, as 0.2248.(4.4/9) + 5. (4.6/9) Vo = max (11-10) 1.04 = max(1, 2.5629) = 2.5629. This computes the value of the European put option as well as and the optimal strategy: Stop or continue at each node depending on which value is larger. Observe that when we compute V2(TT) and V. (T), it is beneficial to exercise at that point rather than continuing. Hence in this case this American put option has strictly larger value than that of the corresponding European version. (263) ax{11-15) {, (264) Exercise 2. In Example 5.2 in LN Chapter 2, compute the price V, of the European put option with the same payoff. Verify that the American version of the put option indeed has strictly larger value than the European version