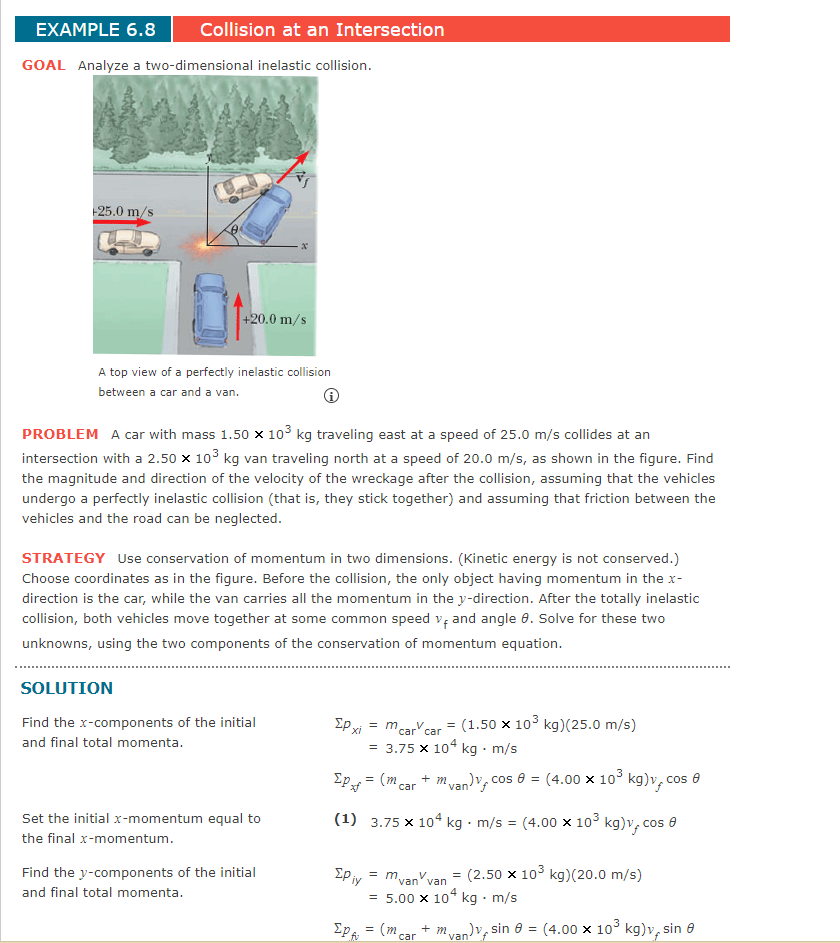
EXAMPLE 6.8 Collision at an Intersection GOAL Analyze a two-dimensional inelastic collision. +25.0 m/s +20.0 m/s A top view of a perfectly inelastic collision between a car and a van. PROBLEM A car with mass 1.50 103 kg traveling east at a speed of 25.0 m/s collides at an intersection with a 2.50 103 kg van traveling north at a speed of 20.0 m/s, as shown in the figure. Find the magnitude and direction of the velocity of the wreckage after the collision, assuming that the vehicles undergo a perfectly inelastic collision (that is, they stick together) and assuming that friction between the vehicles and the road can be neglected. STRATEGY Use conservation of momentum in two dimensions. (Kinetic energy is not conserved.) Choose coordinates as in the figure. Before the collision, the only object having momentum in the x- direction is the car, while the van carries all the momentum in the y-direction. After the totally inelastic collision, both vehicles move together at some common speed vr and angle 0. Solve for these two unknowns, using the two components of the conservation of momentum equation. SOLUTION Find the x-components of the initial and final total momenta. Set the initial x-momentum equal to the final x-momentum. Find the y-components of the initial and final total momenta. = =mcar car car = (1.50 x 103 kg) (25.0 m/s) 3.75 x 104 kg. m/s = (m, + m van), cos 0 = (4.00 103 kg)v, cos car (1) 3.75 x 104 kg m/s = (4.00 103 kg)v, cos = mvan van (2.50 x 103 kg)(20.0 m/s) = 4 = 5.00 x 10 kg. m/s p = (m car+mvan), sin 0 = (4.00 10 kg)v, sin 8 Find the y-components of the initial and final total momenta. Set the initial y-momentum equal to the final y-momentum. Divide Equation (2) by Equation (1) and solve for 0. Piy = mvan van (2.50 x 10 kg)(20.0 m/s) = 4 = 5.00 x 10 kg m/s PA = (m car +mvans sin 0 = (4.00 10 kg)v, sin e (2) 5.00 x 10 kg m/s = (4.00 103 kg)v, sin . 5.00 104 kg - m/s tan = 1.33 3.75 x 104 kg m/s 0 = 53.1 Substitute this angle back into Equation (2) to find v 5.00 x 104 kg m/s (4.00 x 103 kg) sin 53.1 = 15.6 m/s LEARN MORE REMARKS It's also possible to first find the x- and y-components v and v of the resultant velocity. Vfx Vfx The magnitude and direction of the resultant velocity can then be found with the Pythagorean theorem, v +V2, and the inverse tangent function 9 = tan (VA/VA). Setting up this alternate approach is a simple matter of substituting v = cos 0 and v = v sin in Equations (1) and (2). QUESTION If the car and van had identical mass and speed, what would the resultant angle have been? PRACTICE IT Use the worked example above to help you solve this problem. A car with mass 1.43 103 kg traveling east at a speed of 22.4 m/s collides at an intersection with a 2.57 103 kg van traveling north at a speed of 21.5 m/s, as shown in the figure. Find the magnitude and direction of the velocity of the wreckage after the collision, assuming that the vehicles undergo a perfectly inelastic collision (that is, they stick together) and assuming that friction between the vehicles and the road can be neglected. magnitude direction m/s counterclockwise from the +x-axis EXERCISE HINTS: GETTING STARTED | I'M STUCK! A 3.17 kg object initially moving in the positive x-direction with a velocity of +5.14 m/s collides with and sticks to a 2.06 kg object initially moving in the negative y-direction with a velocity of -3.47 m/s. Find the final components of velocity of the composite object. (Indicate the direction with the sign of your answer.) II m/s m/s