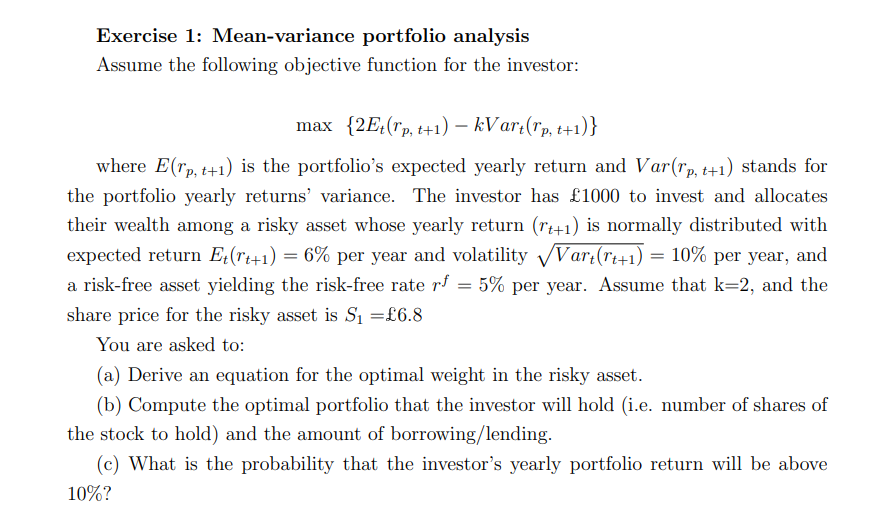
Exercise $1$: Mean$-$variance portfolio analysis

Assume the following objective function for the investor:

max$\{2E_t(r_{p,t+1})-kVa{r}_t(r_{p,t+1})\}$

where $E(r_{p,t+1})$ is the portfolio's expected yearly return and Var$(r_{p,t+1})$ stands for

the portfolio yearly returns' variance. The investor has $1000$ to invest and allocates

their wealth among a risky asset whose yearly return $(r_{t+1})$ is normally distributed with

expected return $E_t(r_{t+1})=6\%$ per year and volatility $\sqrt[\phantom{2}]{Va{r}_t(r_{t+1})}=10\%$ per year, and

a risk$-$free asset yielding the risk$-$free rate $r^f=5\%$ per year. Assume that $k=2,$ and the

share price for the risky asset is $S_1=6\mathrm{.}8$

You are asked to:

$($a$)$ Derive an equation for the optimal weight in the risky asset.

$($b$)$ Compute the optimal portfolio that the investor will hold $($i$.$e$.$ number of shares of

the stock to hold$)$ and the amount of borrowing$/$lending$.$

$($c$)$ What is the probability that the investor's yearly portfolio return will be above

$10\%?$