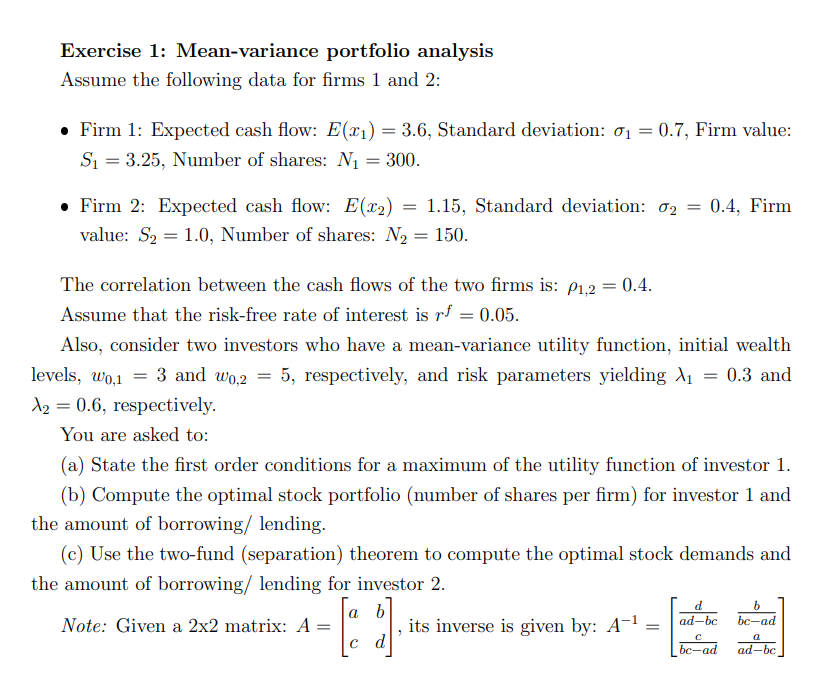
Exercise 1: Mean-variance portfolio analysis Assume the following data for firms 1 and 2 : - Firm 1: Expected cash flow: E(x1)=3.6, Standard deviation: 1=0.7, Firm value: S1=3.25, Number of shares: N1=300. - Firm 2: Expected cash flow: E(x2)=1.15, Standard deviation: 2=0.4, Firm value: S2=1.0, Number of shares: N2=150. The correlation between the cash flows of the two firms is: 1,2=0.4. Assume that the risk-free rate of interest is rf=0.05. Also, consider two investors who have a mean-variance utility function, initial wealth levels, w0,1=3 and w0,2=5, respectively, and risk parameters yielding 1=0.3 and 2=0.6, respectively. You are asked to: (a) State the first order conditions for a maximum of the utility function of investor 1. (b) Compute the optimal stock portfolio (number of shares per firm) for investor 1 and the amount of borrowing/ lending. (c) Use the two-fund (separation) theorem to compute the optimal stock demands and the amount of borrowing/ lending for investor 2. Note: Given a 2x2 matrix: A=[acbd], its inverse is given by: A1=[adbcdbcadcbcadbadbca] Exercise 1: Mean-variance portfolio analysis Assume the following data for firms 1 and 2 : - Firm 1: Expected cash flow: E(x1)=3.6, Standard deviation: 1=0.7, Firm value: S1=3.25, Number of shares: N1=300. - Firm 2: Expected cash flow: E(x2)=1.15, Standard deviation: 2=0.4, Firm value: S2=1.0, Number of shares: N2=150. The correlation between the cash flows of the two firms is: 1,2=0.4. Assume that the risk-free rate of interest is rf=0.05. Also, consider two investors who have a mean-variance utility function, initial wealth levels, w0,1=3 and w0,2=5, respectively, and risk parameters yielding 1=0.3 and 2=0.6, respectively. You are asked to: (a) State the first order conditions for a maximum of the utility function of investor 1. (b) Compute the optimal stock portfolio (number of shares per firm) for investor 1 and the amount of borrowing/ lending. (c) Use the two-fund (separation) theorem to compute the optimal stock demands and the amount of borrowing/ lending for investor 2. Note: Given a 2x2 matrix: A=[acbd], its inverse is given by: A1=[adbcdbcadcbcadbadbca]