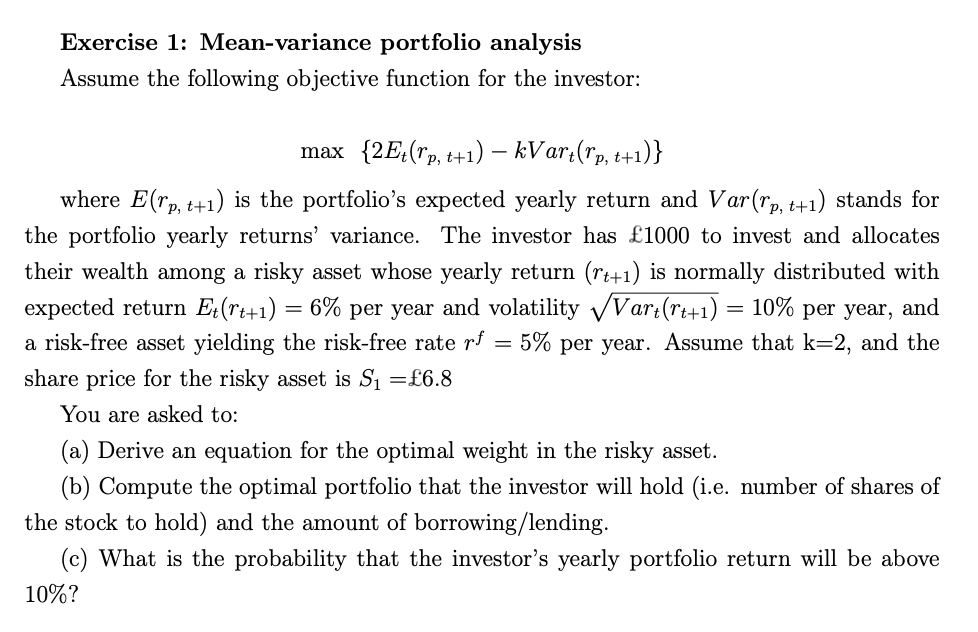Exercise 1: Mean-variance portfolio analysis Assume the following objective function for the investor: max{2Et(rp,t+1)kVart(rp,t+1)} where E(rp,t+1) is the portfolio's expected yearly return and Var(rp,t+1) stands for the portfolio yearly returns' variance. The investor has 1000 to invest and allocates their wealth among a risky asset whose yearly return (rt+1) is normally distributed with expected return Et(rt+1)=6% per year and volatility Vart(rt+1)=10% per year, and a risk-free asset yielding the risk-free rate rf=5% per year. Assume that k=2, and the share price for the risky asset is S1=6.8 You are asked to: (a) Derive an equation for the optimal weight in the risky asset. (b) Compute the optimal portfolio that the investor will hold (i.e. number of shares of the stock to hold) and the amount of borrowing/lending. (c) What is the probability that the investor's yearly portfolio return will be above 10%