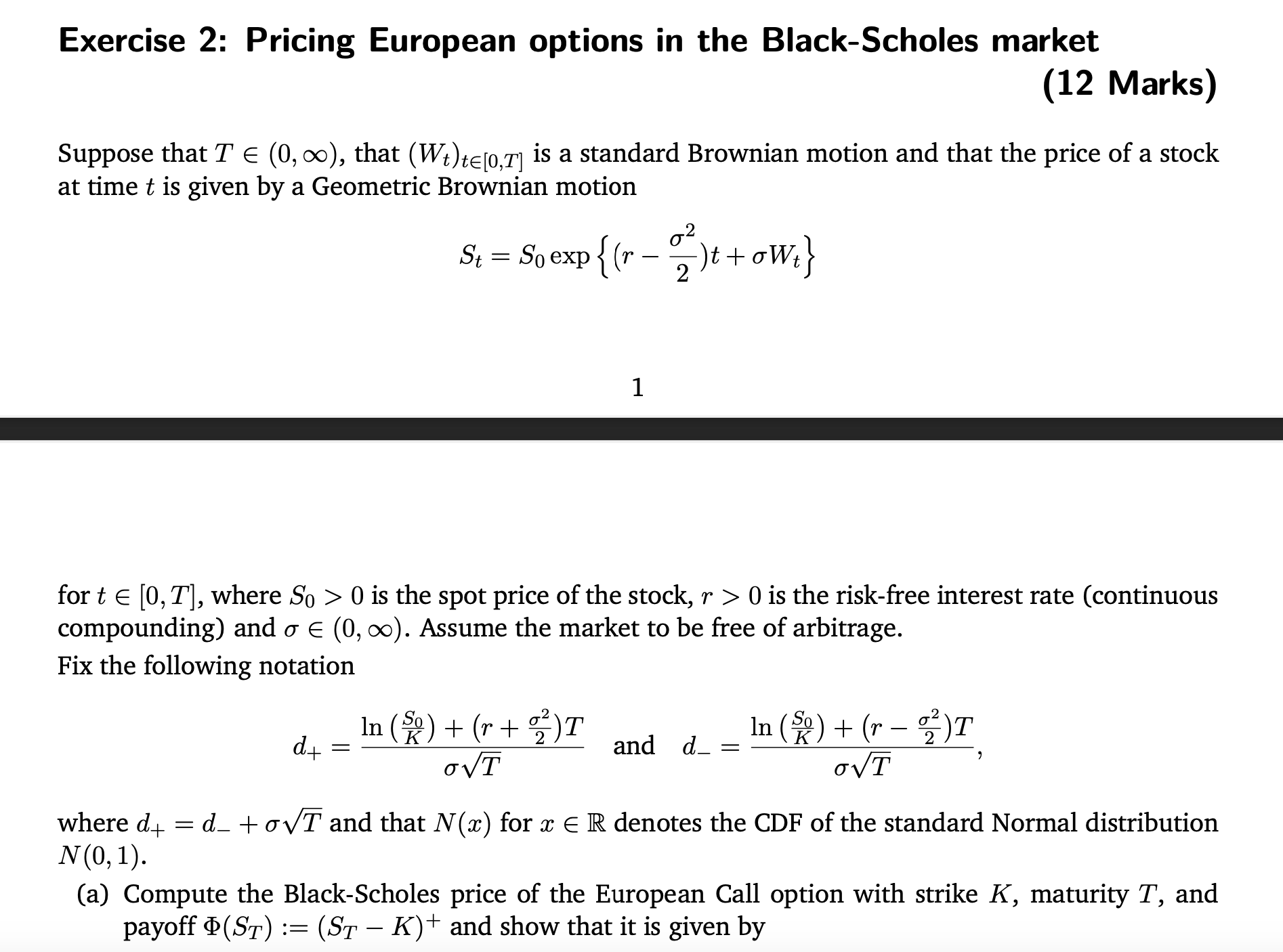
Exercise 2: Pricing European options in the Black-Scholes market (12 Marks) Suppose that T = (0,), that (W)te[0,T] is a standard Brownian motion and that the price of a stock at time t is given by a Geometric Brownian motion S = So exp { (r = 0 )t + oW} 2 1 for t [0,T], where So > 0 is the spot price of the stock, r > 0 is the risk-free interest rate (continuous compounding) and (0,). Assume the market to be free of arbitrage. Fix the following notation d+ = In (s) + (r + 2) T and d_ == In (s) + (r = ) T , + where d = d_ + T and that N(x) for x = R denotes the CDF of the standard Normal distribution N(0, 1). (a) Compute the Black-Scholes price of the European Call option with strike K, maturity T, and payoff (ST) (STK)+ and show that it is given by := [5 marks] (b) Compute the Black-Scholes price of the European Put option with strike K, maturity T, and payoff (ST) = (KST) and show that it is given by + o (Put(T, K)) = KeT N ( d_) SoN (d+). [5 marks] (c) Using the explicit expressions for II (Call(T, K)) and II (Put(T, K)) found above, show that the Put-Call parity holds in the Black-Scholes market model. [2 marks] Exerci 2.