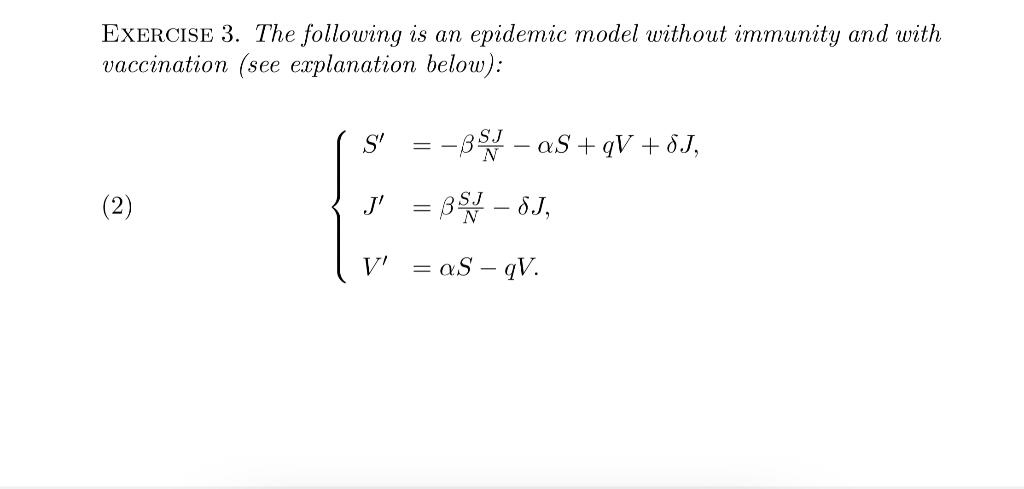
EXERCISE 3. The following is an epidemic model without immunity and with vaccination (see explanation below): S :-B$ - aS + qV +85, (2) J = BSV - SJ, = aS qV. The idea of the model is as follows: The disease provides no immunity. That means after you get infected you can recover but then become susceptible immediately (i.e. you go back to S class). Some individuals are vaccinated and they move to the vaccinated class where they cannot be infected. Some of the vaccinated people move back to the susceptible class due to loss of vaccine effectiveness. The meaning of the variables and parameters is as follows S: the susceptible population (healthy) J: the infected individuals V: the vaccinated individuals N = S + J +V=total population 8: the recovery rate of infected people B: the infection rate a the vaccination rate q: the rate of loss of vaccination protection (a) Show that the total population is constant (does not change with re- spect to time) (b) Since N is constant, write V = N-S-J and transform the above system in a 2x2 differential system in S and J (c) Find all possible equilibrium points (SJ). There should be two of them, one with J = 0 and one with J > 0 (d) Find the Jacobian of the S-J system and evaluate this Jacobian at every equilibrium point you found in part (c) (e) Write down the stability conditions for each of the two equilibria. Based on these conditions find the epidemic reproductive number. This quan- tity, when less than 1 should imply J = 0 is stable and when greater than 1 should imply J > 0 is stable. (f) What is the epidemic reproductive number in the absence of vaccina- tion? What is the proportion of susceptibles that need to be vaccinated to PREVENT an epidemic? (see the discussion at the end of the HW from WEEK 4) (9) Suppose that B = 0.3 and 8 = 0.1. Based on what you found so far answer the following: If no vaccination is administered (a = 0) does the disease go away or persist? If it persists, how much vaccination should be administered (a greater than what???) so that the disease is eliminated, assuming that this vaccination has a failure rate of q = 0.15