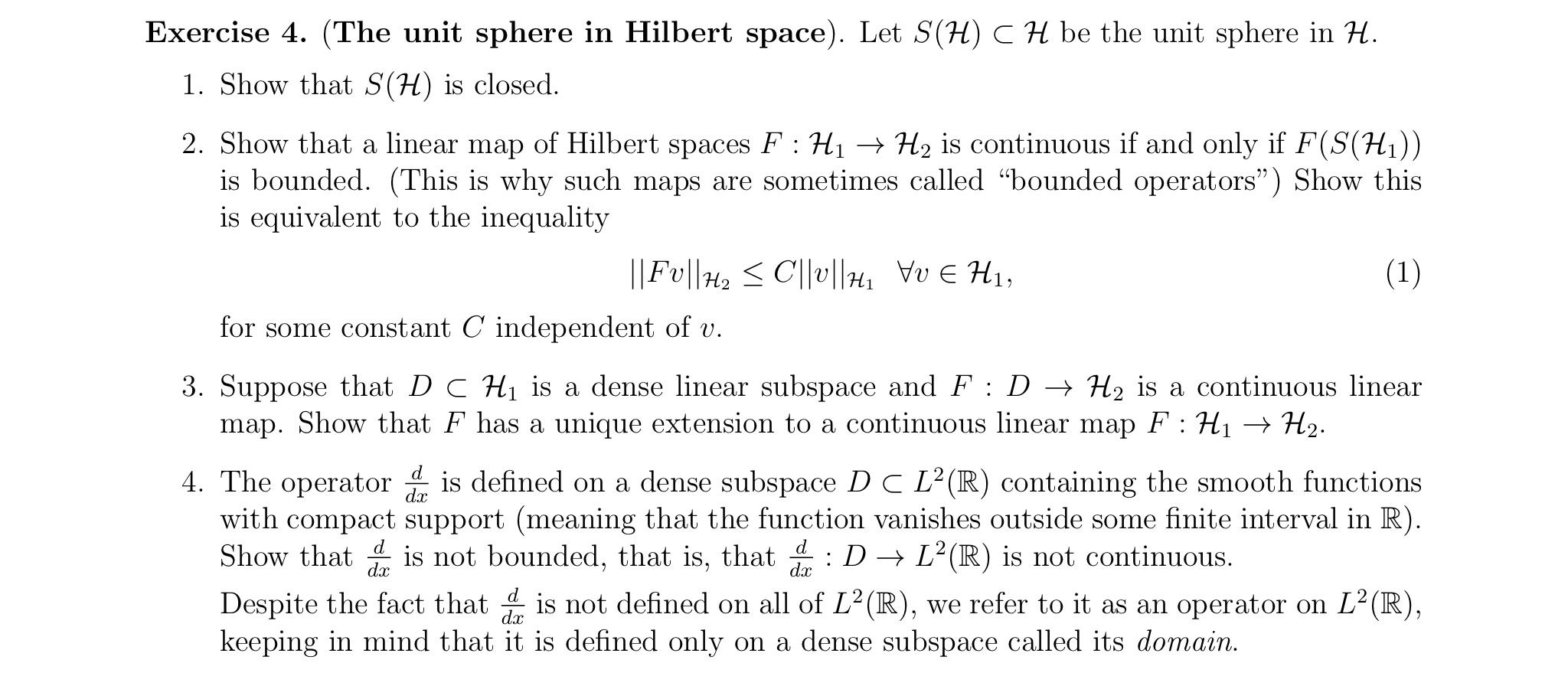
Exercise 4. (The unit sphere in Hilbert space). Let S(H) CH be the unit sphere in H. 1. Show that S(H) is closed. 2. Show that a linear map of Hilbert spaces F : H H2 is continuous if and only if F(S(H1)) is bounded. (This is why such maps are sometimes called bounded operators") Show this is equivalent to the inequality ||FV||H2 C ||V||H Vv E H1, (1) for some constant C independent of v. 3. Suppose that D C H is a dense linear subspace and F : D H is a continuous linear map. Show that F has a unique extension to a continuous linear map F: H1 H2. d dx 4. The operator is defined on a dense subspace DC L (R) containing the smooth functions with compact support (meaning that the function vanishes outside some finite interval in R). Show that is not bounded, that is, that 1: D L(R) is not continuous. Despite the fact that is not defined on all of L (R), we refer to it as an operator on L(R), keeping in mind that it is defined only on a dense subspace called its domain. dx dx dx