Explain the following questions as attached below.

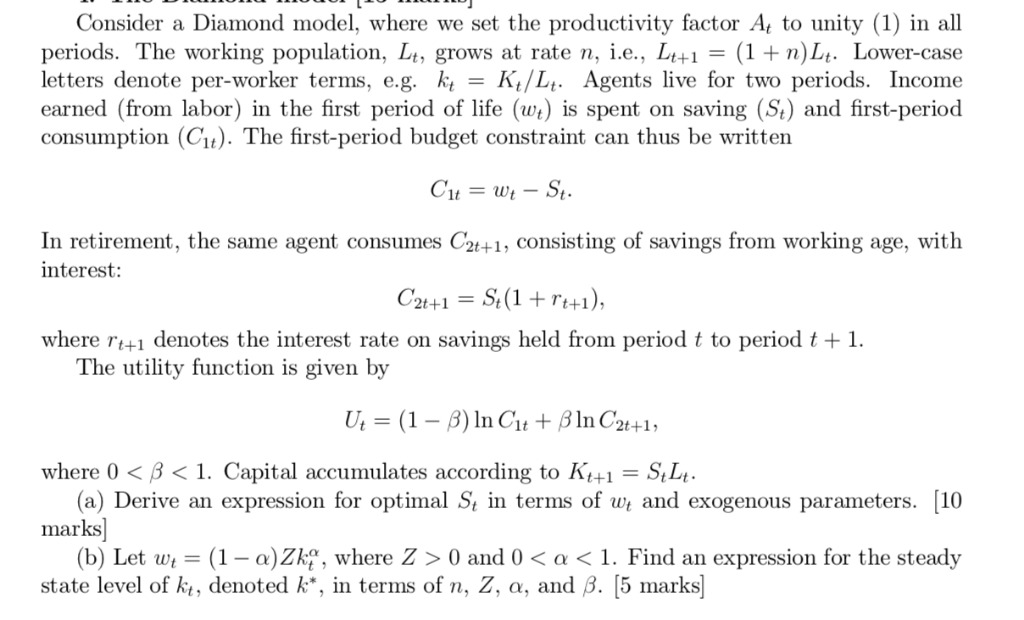
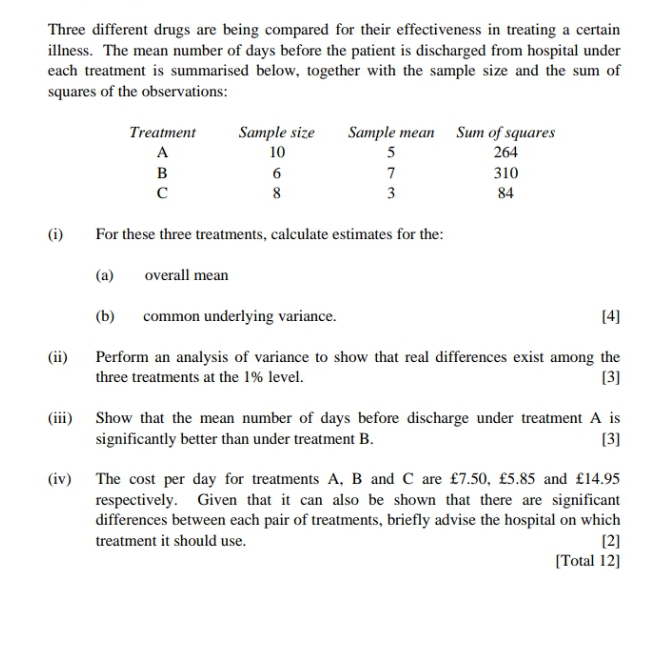
Question 1: Fact I: Consider the following setup that follows the standard Solow model in Country A. There are N consumers, each endowed with one unit of available time. Consumers do not value leisure and they divide output between consumption and savings according to the following rule: a fraction s of output is saved, and the rest is consumed. There is a representative firm that has a Cobb- Douglas production technology of the form Y = zF(K,N), where K denotes capital, N denotes Labour, z is (Total Factor Productivity (TEP). Initially country A had 100 units of Capital, 144 units of labour and population growth rate was 0.01. Suppose you are given the fact that in this economy depreciation d is 0.09 and at steady state, the output per-capita could be expressed as y* = (k*15. Now, consider the unfortunate situation where a disease took several lives, reducing the number of labour force equal to 81 units. 1.1 Describe and explain changes/effect of this disease on country A's per-capita output, and per capita capital in the steady state, comparing these with the initial steady state that was prevailing before the disaster. (Note: you are supposed to describe and explain changes in detail, following Solow Model. The numbers are provided to give you more details about the economy, but you are not required to provide mathematical derivationsumbers for this question). 1.2 If you were illustrating the old and new steady state in a diagram, with per-capita capital in x-axis, describe how your graph would change before and after the disease. Would you expect the growth rate of output per worker in country A smaller or greater than it was before the disease? Question 2: Consider country B, where every citizen is identical (like clones of each other) and each individual has one unit of labour to supply. The country produces only one commodity, food. Current aggregate output of food, Y, is produced using current inputs of land, L. and current labour, N, that is Y = zF(L,N), where z is total factor productivity (TP). Future population is N", population grows at a rate n = 0.01, and depends on consumption per worker, c - C/N. There is no money; wage payment is provided solely in terms of foods. In equilibrium all foods produced in the economy are consumed and this is a closed economy without any government, net export or savings. Consider the Malthus model in the context of country B. Suppose the economy is currently at steady state. Now suppose considerable amount of land is destroyed due to an earthquake in country B. Describe and explain changes/effect of this incidence on steady state land per capita, and standard of living. Explain how wage would be determined in country B and how this process (of determining wage in Mathus model) is different compared to the competitive equilibrium models we studied in Chapter 4 and 5. Explain if you think wage would be higher or lower in country B after the earthquake. Question 3: Fact II. Assume the endogenous growth model (from Chapter 8) perfectly explain the growth situation in the country Econland, where a representative consumer starts the current period with H. units of human capital and does not use time for leisure. In each period, the consumer has one unit of time, which can be allocated between work and accumulating human capital. Let u denote the fraction of time devoted to working in each period. The consumer's quantity of human capital is the measure of the productivity of the consumer's time when he or she is working. For each efficiency unit of labour supplied, the consumer receives the current real wage w. The consumer cannot save, but they can trade off current consumption for future consumption by accumulating human capital. b is a parameter that captures the efficiency of the human capital accumulation technology, with b > 0. The representative firm produces output using only efficiency units of labour. The production function is given by Y -zuHe where He is the units of labour in production. Sunnose the economy of Ecoaland was initially in a steady state with H.= Has He In the bane of giving the economy a"'""" " '\"'\""""' ---...........- I." \"""'" ""1 Consider a Diamond model, where we set the productivity factor A; to unity (1) in all periods. The working population, Lg, grows at rate R, i. e., Li\": (1 + n)L. Lower-case letters denote perworker terms, e.g. k, = K,/L,. Agents live for tam periods. Income earned (from labor) in the rst period of life (10,) is spent on saving (3,) and rstperiod consumption (Cu). The rst-period budget constraint can thus be written 011 = to: ' 31. In retirement, the same agent consumes 02, ule. Problems: 1. (Hand) Using the model where the growth rate for the usage of petroleum is constant and assuming that our current reserves are 3,000,000 million barrels, how long would these reserves last if the growth rate was constant at 4.7% (the rate for the base year) and 2%? 2. (Hand) Make an observation about how these compare to each other and how they compare to the length of time the petroleum reserves would last for the model where the growth rate is a function of time from our reading above, i.e. the growth rate is k() - 4 25 0.5 Specifically, comment on how the variable growth rate k(t) would compare with the fixed rate of 2% in the short term and in the long term (short being 1 or 2 years vs long term 25 to 50 years). No need to do any calculation just explain how these would be similar yet different (Hint Hint). 3. (Hand) Bituminous coal has estimated world reserves of 2,000,000 million tons with a consumption in the base year of 2200 million tons. If the rate of consumption has a constant growth rate of 4%, estimate the length of time until this mineral is depleted. 4. (Hand) (a) Verify that the growth rate function k(t) from question 2 has the right values when t = 0 and t = 25. (Refer to the bottom of page 2 to see what the "right" values are.) (b) Find a similar function of the form k(t) = = , so that &(0) = 0.04 and k(25) - 0.02. (You must solve this algebraically and show your work) (c) With this new function k(t) in mind what will the growth rate be after 50 years and after 100 years? Hand and Excel)Three different drugs are being compared for their effectiveness in treating a certain illness. The mean number of days before the patient is discharged from hospital under each treatment is summarised below, together with the sample size and the sum of squares of the observations: Treatment Sample size Sample mean Sum of squares A 10 264 B 6 310 84 (i) For these three treatments, calculate estimates for the: (a) overall mean (b) common underlying variance. [4] (ii) Perform an analysis of variance to show that real differences exist among the three treatments at the 1% level. [3] (iii) Show that the mean number of days before discharge under treatment A is significantly better than under treatment B. [3] (iv) The cost per day for treatments A. B and C are f7.50, f5.85 and f14.95 respectively. Given that it can also be shown that there are significant differences between each pair of treatments, briefly advise the hospital on which treatment it should use. [2] [Total 12]Three different drugs are being compared for their effectiveness in treating a certain illness. The mean number of days before the patient is discharged from hospital under each treatment is summarised below, together with the sample size and the sum of squares of the observations: Treatment Sample size Sample mean Sum of squares A 10 264 B 6 310 84 (i) For these three treatments, calculate estimates for the: (a) overall mean (b) common underlying variance. [4] (ii) Perform an analysis of variance to show that real differences exist among the three treatments at the 1% level. [3] (iii) Show that the mean number of days before discharge under treatment A is significantly better than under treatment B. [3] (iv) The cost per day for treatments A. B and C are f7.50, f5.85 and f14.95 respectively. Given that it can also be shown that there are significant differences between each pair of treatments, briefly advise the hospital on which treatment it should use. [2] [Total 12]