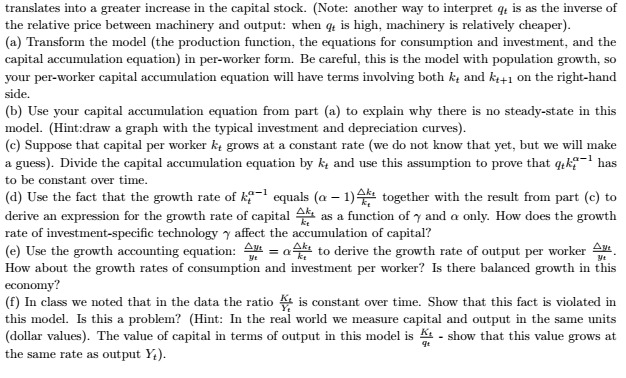

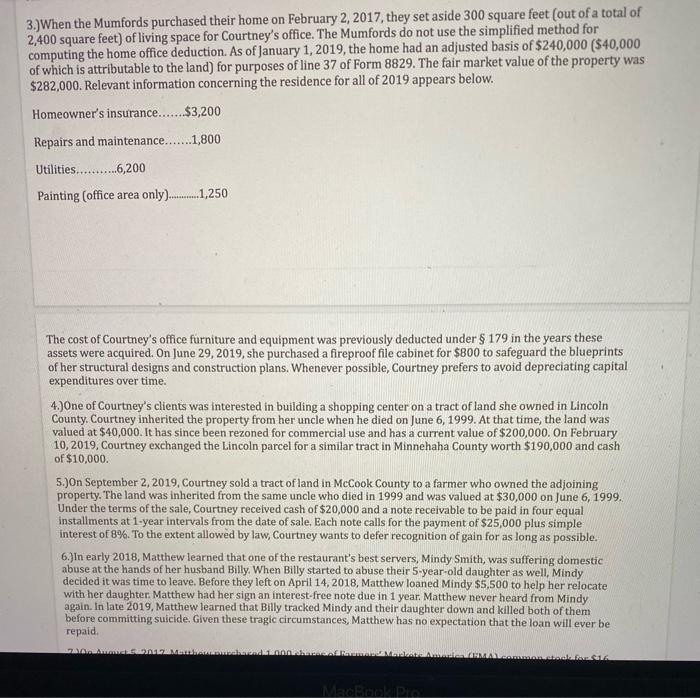

Explain the following .questions.
translates into a greater increase in the capital stock. (Note: another way to interpret q, is as the inverse of the relative price between machinery and output: when q, is high, machinery is relatively cheaper). (a) Transform the model (the production function, the equations for consumption and investment, and the capital accumulation equation) in per-worker form. Be careful, this is the model with population growth, so your per-worker capital accumulation equation will have terms involving both k, and kit on the right-hand side. (b) Use your capital accumulation equation from part (a) to explain why there is no steady-state in this model. (Hint:draw a graph with the typical investment and depreciation curves). (c) Suppose that capital per worker k, grows at a constant rate (we do not know that yet, but we will make a guess). Divide the capital accumulation equation by , and use this assumption to prove that q,k, has to be constant over time. (d) Use the fact that the growth rate of be equals (o - 1)4% together with the result from part (c) to derive an expression for the growth rate of capital k It as a function of y and o only. How does the growth rate of investment-specific technology y affect the accumulation of capital? (e) Use the growth accounting equation: 2M =04* to derive the growth rate of output per worker How about the growth rates of consumption and investment per worker? Is there balanced growth in this economy? (f) In class we noted that in the data the ratio ~ is constant over time. Show that this fact is violated in this model. Is this a problem? (Hint: In the real world we measure capital and output in the same units (dollar values). The value of capital in terms of output in this model is : - show that this value grows at the same rate as output Y?).1. Suppose that the representative firm owns its capital stock (K), hires labor (N), and produces output (Y) according to Y, = A,KPN, " where A, is productivity and 0 [J is a parameter and {J <: :3 is the subjective discount factor. agent faces two period budget constrains: c1 s="ml" and c2="(1" r here represents second assets savings. find optimal consumption savings decision of this agent. how do change with interest rate consider basic twcrperiod labor supply an that only works in rst period. let her be denoted by suppose time normalized to so has preferences over e given by: hence gets utility from leisure l times constraints: cl wage1 lending borrowing a tax pay>














