Factor Company is planning to add a new product to its line. To manufacture this product, the company needs to buy a new machine at a $495,000 cost with an expected four-year life and a $20,000 salvage value. Additional annual information for this new product line follows. (PV of $1, FV of $1, PVA of $1, and FVA of $1)

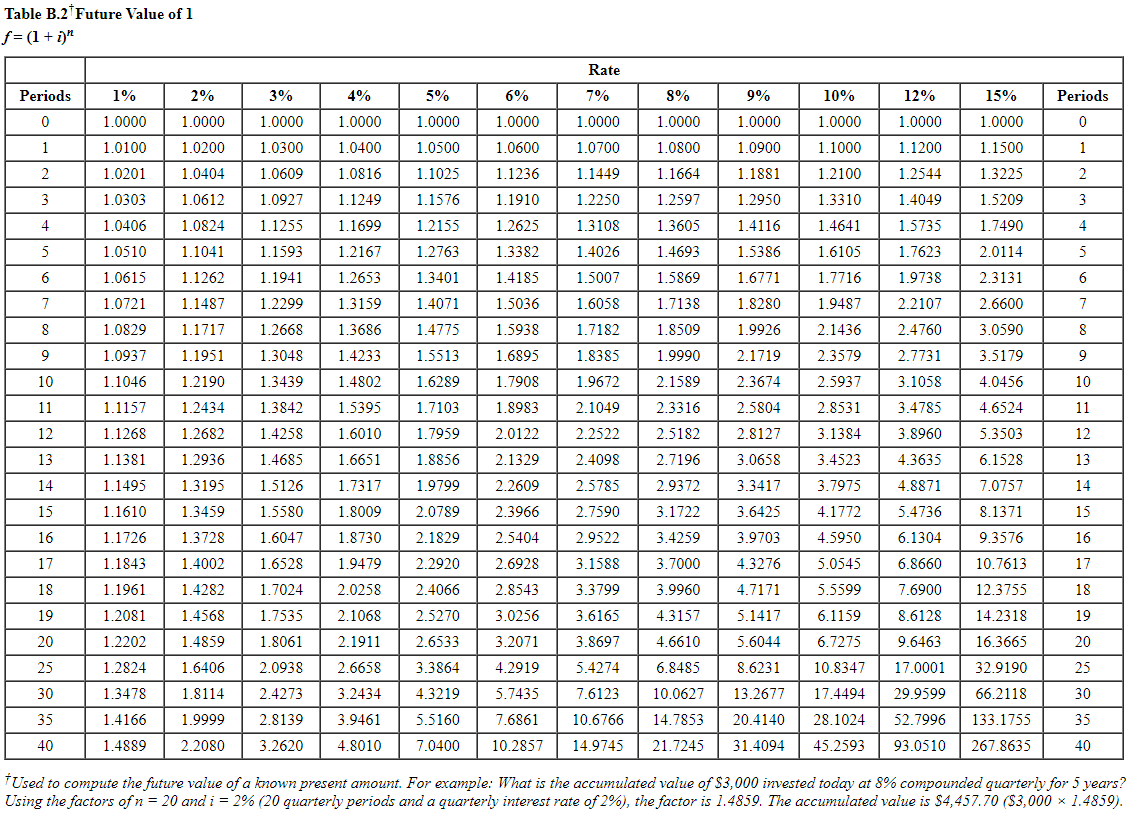
Note: Use appropriate factor(s) from the tables provided.

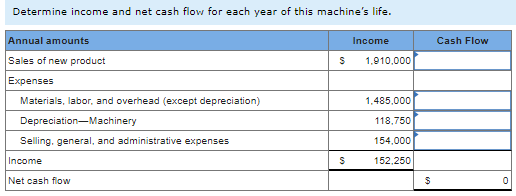
Sales of new product	$ 1,910,000
Expenses
Materials, labor, and overhead (except depreciation)	1,485,000
DepreciationMachinery	118,750
Selling, general, and administrative expenses	154,000

Required:

Determine income and net cash flow for each year of this machines life.

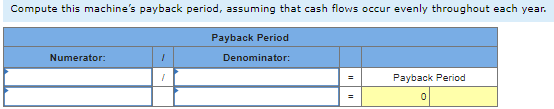
Compute this machines payback period, assuming that cash flows occur evenly throughout each year.

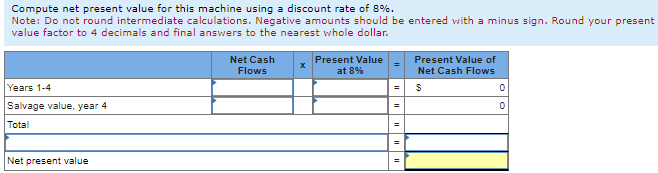
Compute net present value for this machine using a discount rate of 8%.

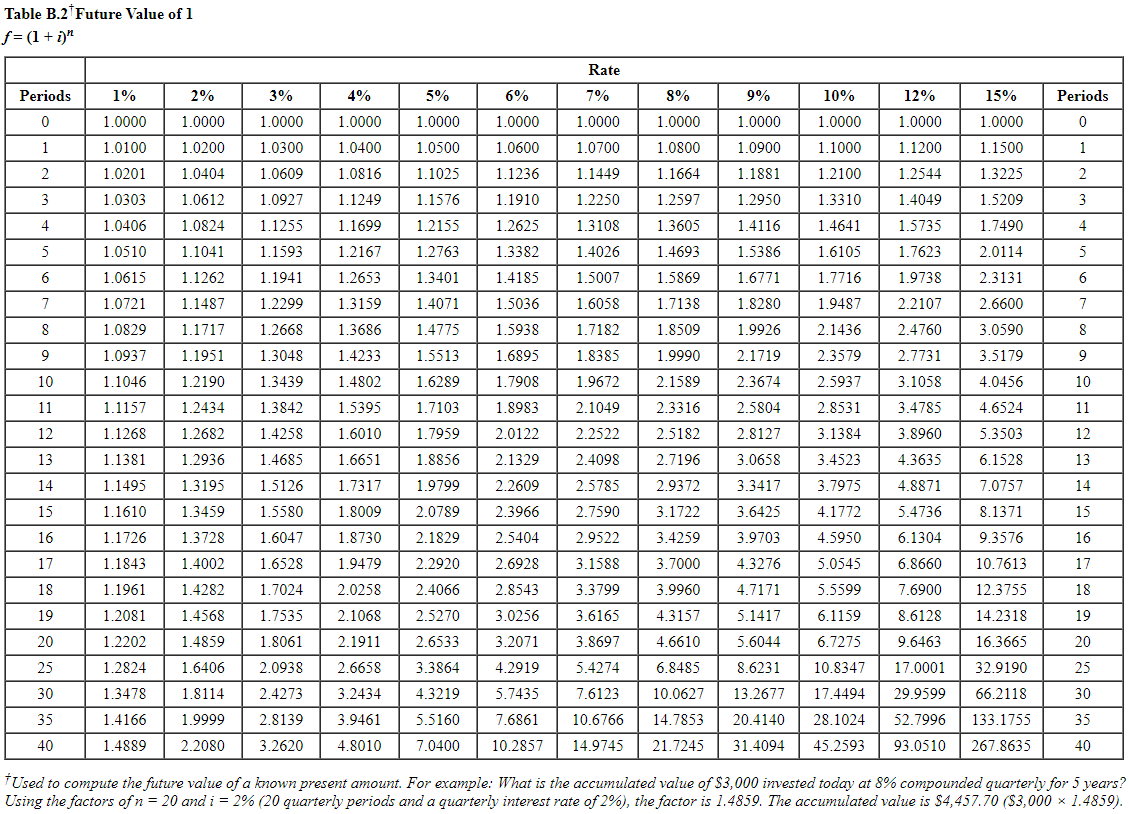
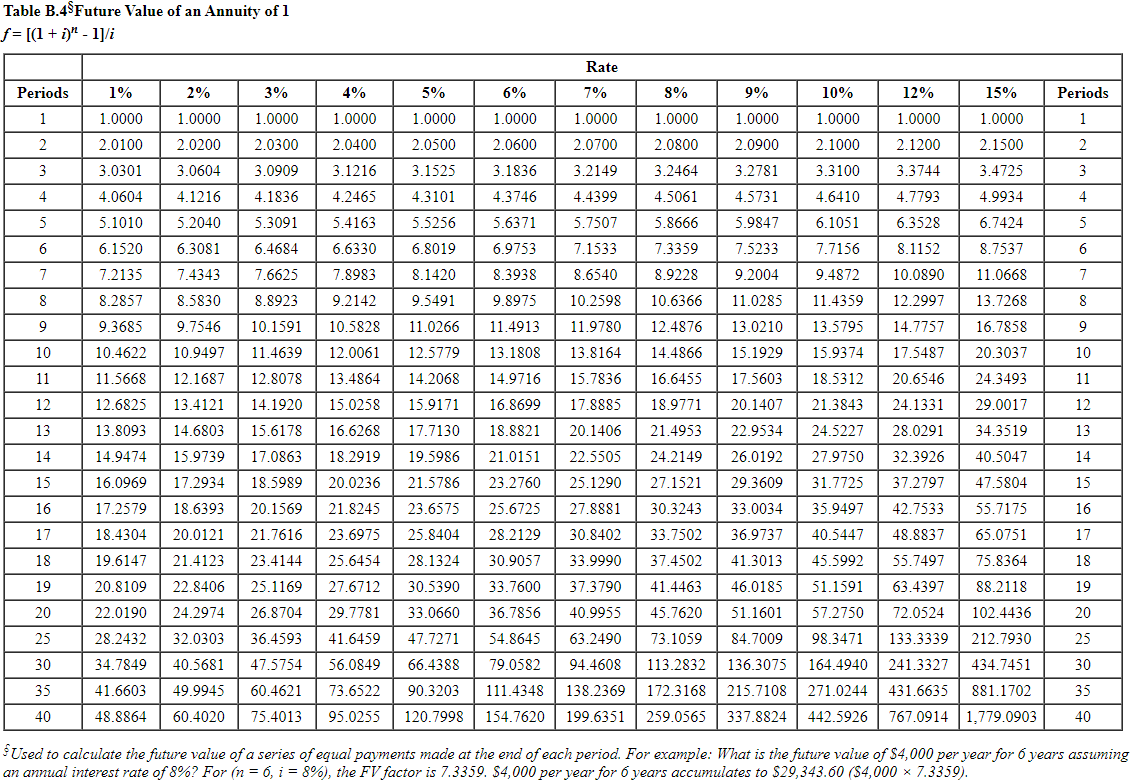Determine income and net cash flow for each year of this machine's life. Compute this machine's payback period, assuming that cash flows occur evenly throughout each year. \begin{tabular}{|l|c|c|c|c|c|} \hline \multicolumn{9}{|c|}{ Payback Period } \\ \hline & 1 & Denominator: & \\ \hline Numerator: & / & & = & \multicolumn{2}{|c|}{ Payback Period } \\ \hline & & & = & 0 & \\ \hline \end{tabular} Compute net present value for this machine using a discount rate of 8%. Note: Do not round intermediate calculations. Negative amounts should be entered with a minus sign. Round your present value factor to 4 decimals and final answers to the nearest whole dollar. Table B.1* Present Value of 1 p=1/(1+i)n in 6 years from today? Using the factors of n=12 and i=5% (12 semiannual periods and a semiannual rate of 5% ), the factor is 0.5568. You would need to invest $2,784 today ($5,0000.5568) Table B. 2 Future Value of 1 f=(1+i)n Using the factors of n=20 and i=2% (20 quarterly periods and a quarterly interest rate of 2%), the factor is 1.4859 . The accumulated value is $4,457.70 ( $3,0001.4859 ). Table B. 2 Future Value of 1 f=(1+i)n Using the factors of n=20 and i=2% (20 quarterly periods and a quarterly interest rate of 2%), the factor is 1.4859 . The accumulated value is $4,457.70 ( $3,0001.4859 ). Table B. 4 Future Value of an Annuity of 1 f=[(1+i)n1]/i