Financial Institution And Financial Market

Book: Financial Institutions Management 6^th edition by Anthony Saunders and Marcia Millon Cornett

1. 30-year mortgage loans are pretty popular in Chinese residential housing market. Suppose on its balance sheet, a commercial bank has a 30-year mortgage loan. The relevant information is as follows.

(1) The present value of the mortgage loan (i.e., total loan made today) = RMB 5,000,000;

(2) Suppose the annual prime lending rate = 6.55%, but for this mortgage loan there is a discount rate of 85%. That is, the annual lending interest rate is actually = 0.85 * 6.55%;

(3) The maturity of this mortgage loan = 30 years.

The bank offer the borrower two options to repay his mortgage loans, which are

(1) Monthly Straight Line (Average capital plus interest?).

With this option, the borrower's monthly payment (capital plus interest) are equalized for the 360 (12*30) months. That is,

Present Value _Mortgage loan =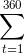= monthly payment/ (1+monthly interest rate) (1)

(2) Monthly Reducing Balance(Average capital?)

With this option, the borrower's payment on capital is constant. That is,

Monthly principal _t = Present value -Mortgage loan / 12*30 (2)

Notice that, under the two options the computation formulas for monthly interest are the same, but the actual interest computed are different due to different remaining principals. The interest is calculated based on the outstanding principal at the end of last month and the interest rate. That is,

interest_t= remaining principal_t-1* monthly interest rate (3)

where

remaining principal_t =total loan -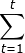 monthly principal_t t>1

= remaining principal_{t-1 -} monthly principal_t (4)

Hint 1: monthly interest rate= Actual annual interest rate/12

=Annualinterestrate*discount rate/12

Based on the information above, answer the following questions. The Excel file has been uploaded. The relevant computation for the first month has been given in the two sheets. Questions below from (1-1) to (1-2) should be done in EXCEL for your own understanding. Please do not report the EXCEL files. Questions of (1-3) to (1-4) need to be answered here.

(1-1) Suppose the bank is offering Option 1 to the borrower, what is the monthly payment? Using Equation (1) to answer this question.

(1-2) For the two options, using EXCEL to compute monthly interest (????), monthly principal ??????? monthly payment ????. You need two work sheets for the two options. Please also compute the present value of each monthly payment. How much is the sum of these present values of monthly payments?

(1-3) Based on the EXCEL results, please report a chart in which the horizon axis is labeled as months and the vertical axis labeled as values of monthly interest, monthly principal, and monthly payment.

(1-4) What are the durations of this 30-year mortgage loan under the two options.

Note that duration is in unit of years. Thus duration for a security with monthly cash flows is:

.

Hint 2: Steps for computation have been given in the EXCEL sheet. Here, I illustrated them again.

Steps under option (1) Monthly Straight Line.

Step 1: Computing Monthly Payment using Equation (1), which is identical for the whole column of monthly payment.

Step 2: Remaining principal before Month 1 = total loan (5,000,000);

Step 3: Monthly Interest in Month 1 = Remaining principal before Month 1 * Monthly Interest Rate;

Step 4: Monthly Principal in Month1=Monthly Payment-Monthly Interest in Month 1;

Step 5: Remaining principal in Month 1 = Remaining principal before Month 1 - Monthly principal in Month 1;

Then repeat step 2-step 5.

Step 6: Present value for each month from month 1 to month 360 (30 years) and the corresponding month's money value in present term

Present value_t = monthly payment_t / (1+monthly interest rate)^t

the month's money value in present_t =present value_t*t

Step 7: Computing Total Present Value of Cash Flows, Total Periods' Money Value In Present, Duration.

Check that Total Loan = total present value of the cash flows!

Total Periods' Money Value In Present= sum of each month's money value in present term;

Steps under option (2) Monthly Reducing Balance.

Step 1: Computing Monthly Principal using Equation (2), which is identical for the whole column of monthly principal.

Step 2: Remaining principal before Month 1 = total loan (5,000,000);

Step 3: Monthly Interest in Month 1 = Remaining principal before Month 1 * Monthly Interest Rate;

Step 4: Monthly Payment in Month1=Monthly Principal in Month1 + Monthly Interest in Month 1;

Step 5: Remaining principal in Month 1 = Remaining principal before Month 1 - Monthly principal in Month 1;

OR

Remaining principal in each Month = Total Loan - Monthly principal * Month Number;

Then repeat step 2-step 5.

Step 6: Present value for each month from month 1 to month 360 (30 years).

Step 7: Computing Total Present Value of Cash Flows, Total Periods' Money Value In Present, Duration.

(1-5) Using your EXCEL file, what happens to the durations of this 30-year mortgage loan under the two options when you vary the total loan made today?

2. For the same commercial bank, its balance sheet and duration structure are as follows.

Asset (millions)	Liability(millions)
3-month Zero-Coupon bonds: 3m; Rate 2%; Duration =?	Overnight FED funds: 2m; Rate 1%; Duration = 0 year (approximately)
1-year corporate loan: 2m; Rate 4%; Duration = 0.7326 year	1-year CDs: 7m; Rate 3%; Duration =?
30-year mortgage loan: 5m; Rate 6.55% (subject to 15% off); Duration: the one under Option 2 in question 1	Equity: 1m

(2-1) What is the RSA and RSL for a 1-year maturity bucket? What is the 1-year repricing gap?

(2-2) What will be the FI's net interest income at year-end if interest rates do not change?

(2-3) Suppose both rate sensitive assets and rate sensitive liabilities' interest rates increase by 1 percent. Calculate the change in net interest income after the interest rate increase.

(2-4) What are the durations of the 3-month Zero-Coupon bond and 1-year CDs? The durations of the FI's asset portfolio and its liability portfolio (excluding the Equity)?

Hint: The 1-year CDs has only one cash flow in its lifetime, and thus works like Zero-Coupon bond.

(2-5) To simplify the calculation, let's ignore the interest rate assigned for each security. Now, assuming all securities' market value are defined at a common market rate of 10%, and the FI expects the market rate will rise to 11%. What are the changes in the market values of asset portfolio, liability portfolio and the equity?

Hint:

(2-6) In case of (2-5). What are the market values of asset portfolio, liability portfolio and the equity after the market rate rises?

3. Suppose an FI has a trading portfolio of the following structure:

(a) six-year zero-coupon bonds with a total market value of $20 million. The current market yield on the bonds is 10 percent. The mean change in daily yields was zero while the standard deviation is 20 basis points. Yield changes are assumed to be independent and normally distributed over time.

(b) A EURO position of -15million (short in EURO). The current market exchange rate is 1.3470USD/EUR. (The FI suffers a loss if a bad moves occur, that is, if EURO appreciates tomorrow). Again, the mean change in daily USD-EURO exchange rate was zero while the standard deviation is 1 percent. Exchange rate changes are assumed to be independent and normally distributed over time.

Please answer the following questions.

(3-1) What is the maximum yield change expected if a 90 percent confidence limit is used?

(3-2) What is the modified duration of these bonds? the price volatility? and the daily earnings at risk (DEAR) of this bond asset?

(3-3) Suppose in question (a), it is the face value of $20 million, not market value. What will be the DEAR?

(3-4) What is the maximum EURO appreciation expected if a 90 percent confidence limit is used? the dollar equivalent value of the EURO position? and the daily earnings at risk (DEAR) of this EURO position?

(3-5) Suppose EURO appreciates when interest rate falls, and p= -0.5. What is the DEAR of the trading portfolio which has the six-year zero-coupon bonds with a total market value of $20 million and the EURO short position of -15million?

(3-6)What is the 10-day VAR of this trading portfolio?