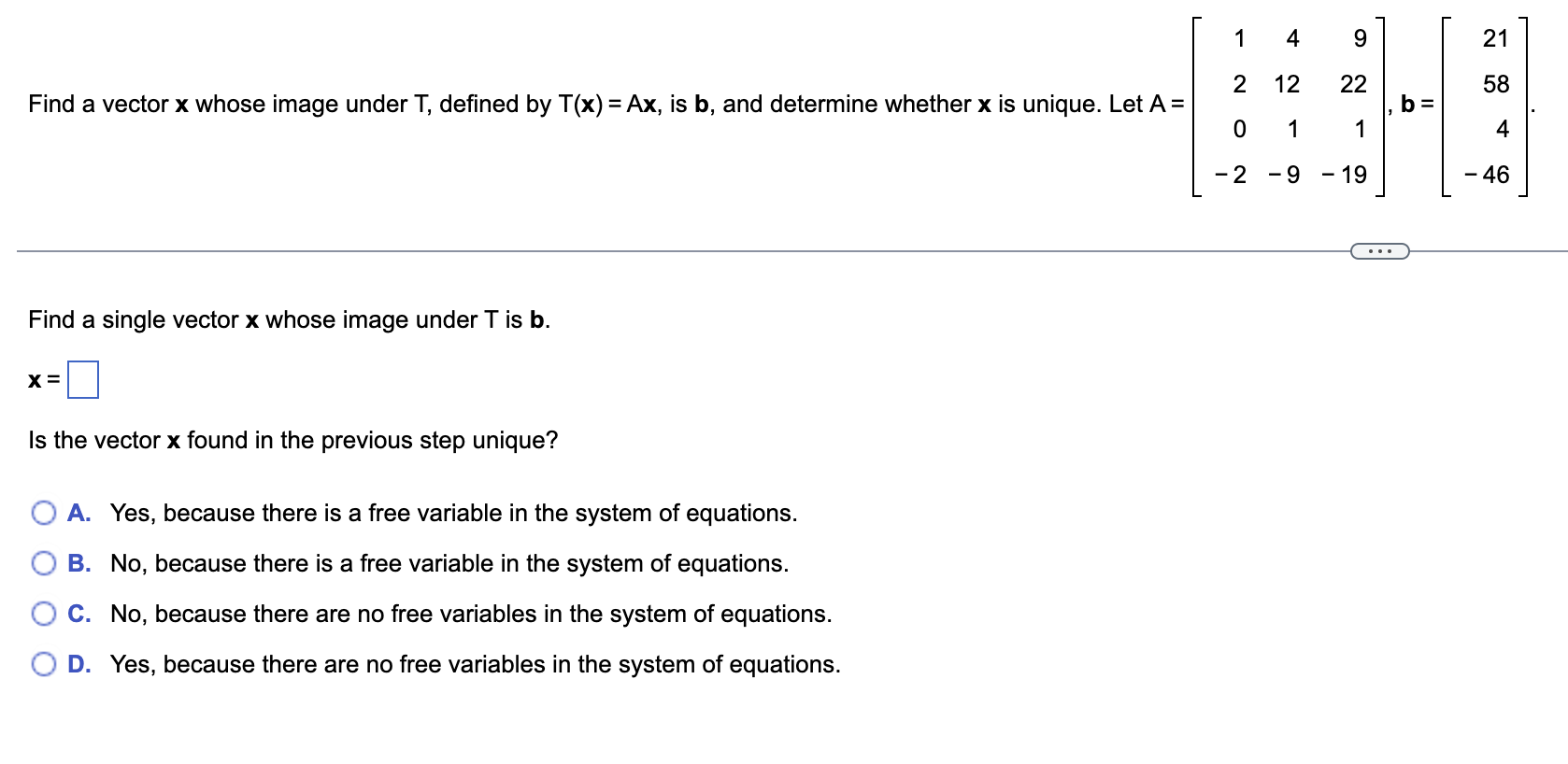
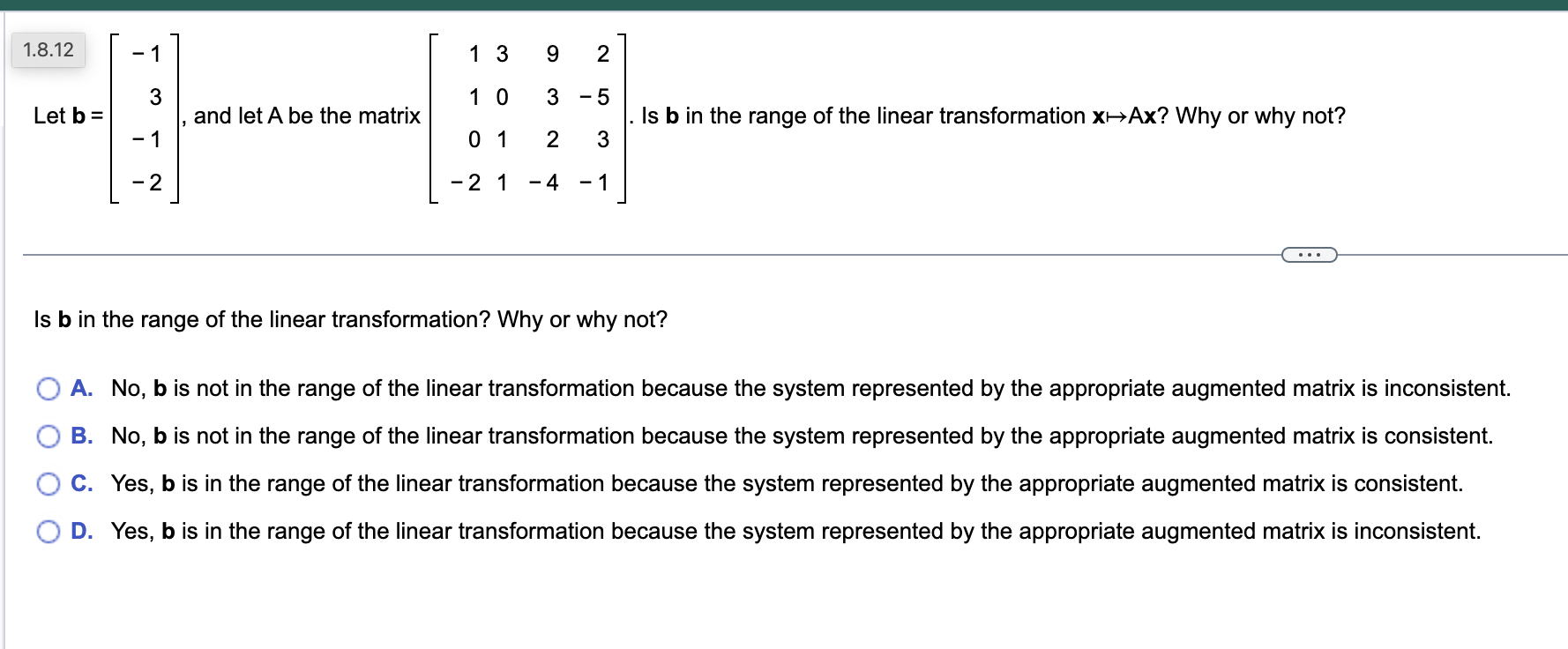
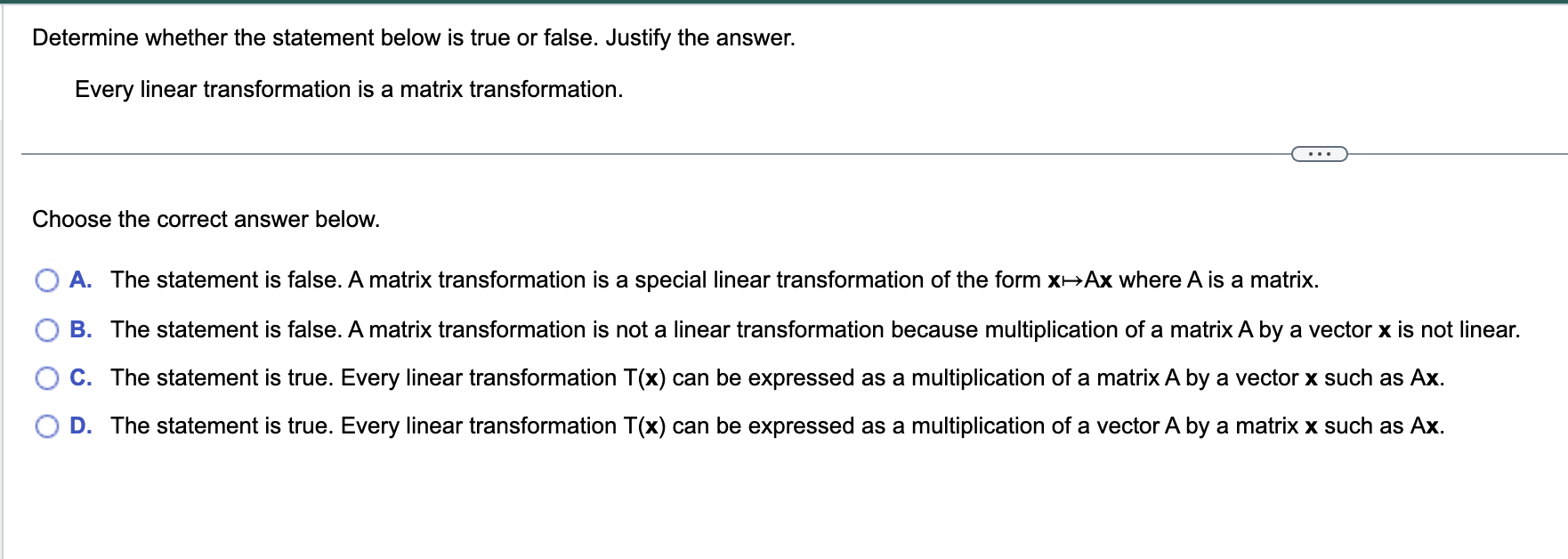
Find a vector x whose image under T, defined by T(x) = Ax, is b, and determine whether x is unique. Let A = Find a single vector x whose image under T is b. x= Is the vector x found in the previous step unique? A. Yes, because there is a free variable in the system of equations. B. No, because there is a free variable in the system of equations. C. No, because there are no free variables in the system of equations. D. Yes, because there are no free variables in the system of equations. 1 4 9 21 2 12 22 58 b = 0 1 1 4 -2-9 -19 -46 1.8.12 1 1 3 9 2 3 103-5 Let b = and let A be the matrix Is b in the range of the linear transformation X-Ax? Why or why not? -1 01 2 3 -2 -21 -4 - - 1 Is b in the range of the linear transformation? Why or why not? A. No, b is not in the range of the linear transformation because the system represented by the appropriate augmented matrix is inconsistent. B. No, b is not in the range of the linear transformation because the system represented by the appropriate augmented matrix is consistent. C. Yes, b is in the range of the linear transformation because the system represented by the appropriate augmented matrix is consistent. D. Yes, b is in the range of the linear transformation because the system represented by the appropriate augmented matrix is inconsistent. Determine whether the statement below is true or false. Justify the answer. Every linear transformation is a matrix transformation. Choose the correct answer below. A. The statement is false. A matrix transformation is a special linear transformation of the form X-Ax where A is a matrix. B. The statement is false. A matrix transformation is not a linear transformation because multiplication of a matrix A by a vector x is not linear. C. The statement is true. Every linear transformation T(x) can be expressed as a multiplication of a matrix A by a vector x such as Ax. D. The statement is true. Every linear transformation T(x) can be expressed as a multiplication of a vector A by a matrix x such as Ax. Assume that T is a linear transformation. Find the standard matrix of T. T: RR4, T (e1) = (4, 1, 4, 1), and T (2) = ( 5, 9, 0, 0), where e = (1,0) and e = (0,1). A = (Type an integer or decimal for each matrix element.)