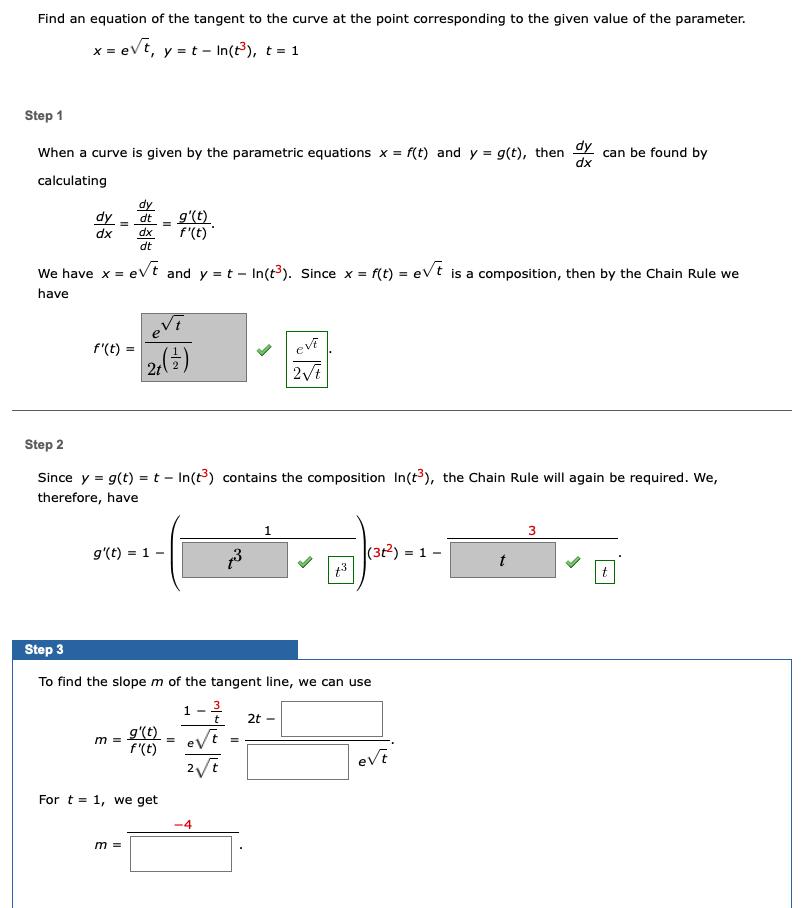
Find an equation of the tangent to the curve at the point corresponding to the given value of the parameter. x= et, y=t-In(t), t = 1 Step 1 When a curve is given by the parametric equations x = f(t) and y = g(t), then dy can be found by dx calculating dy dx dt g'(t) = = dx f'(t) dt We have x = et and y = t - In(t). Since x = f(t) = et is a composition, then by the Chain Rule we have ev f'(t) = 21 (1) evi 2t Step 2 Since y = g(t) = t - In(t) contains the composition In(t), the Chain Rule will again be required. We, therefore, have 1 g'(t)=1- +3 3 |(312) = t +3 Step 3 To find the slope m of the tangent line, we can use 1- t 20- m = g'(t) f'(t) et For t 1, we get = m = -4 et